The set of convex polygons which tile the plane is, as of $2017$, known: it consists of all triangles, all quadrilaterals, $15$ families of pentagons, and three families of hexagons. Euler's formula rules out strictly convex $n$-gons with $n\ge 7$. (The pentagonal case is by far the most difficult one.)
I am interested in pairs of convex polygons that can collectively tile the plane. Specifically, I am curious how many sides a polygon in such a pair can have.
Here are some conditions to impose on such a tiling, from weakest to strongest:
There is at least one copy of each tile. (Without this condition, one can trivially take a pair consisting of a tiling polygon and any other convex polygon, and just never use the latter shape.)
There are at least $k$ copies of each tile.
There are infinitely many of each tile.
Every tile borders a tile of the other type.
The tiling is $2$-isohedral, i.e., every tile can be carried to any other tile of the same shape by a symmetry of the tiling.
Each of these conditions implies those above it.
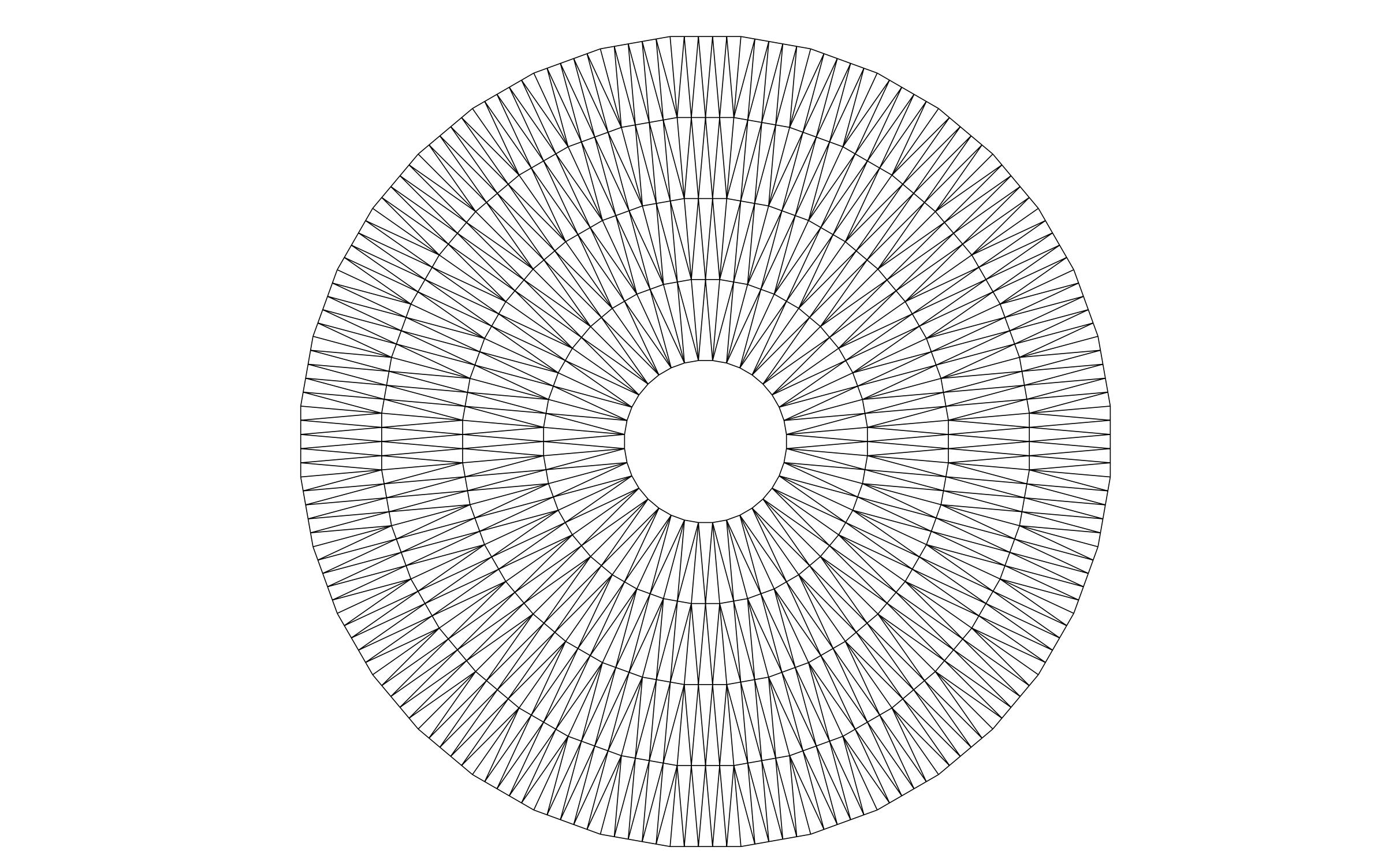
In the weakest case, the number of sides is unbounded, as exhibited by the following example:
(The tiling is constructed by decomposing "wedges" of central angle $2\pi/N$ into congruent isosceles triangles, and then combining the central triangles to yield an $N$-gon in the center.)
Requiring at least $k$ of each tile still yields arbitrarily high numbers of sides, by taking the above construction for $N=M\cdot k$ and subdividing the $N$-gon into $k$ "wedges" which are $(M+2)$-gonal.
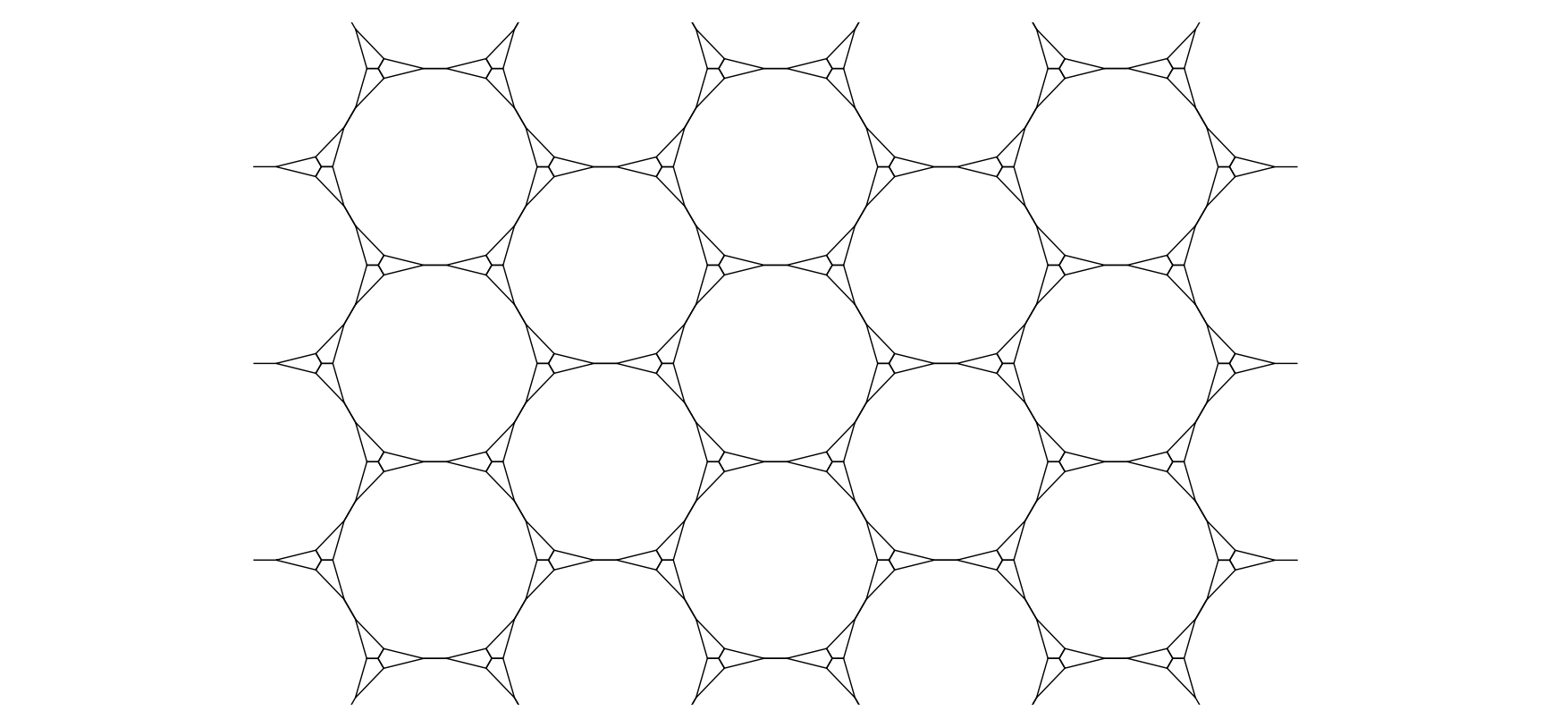
On the other end of the spectrum, I have found a $2$-isohedral tiling using regular $18$-gons, shown below:
After consulting this paper, it seems that the tiling pictured above is of type $4_2 18_{12}-1\text{a}\ \text{MN}\ \text{p}6\text{m}$ in their classification scheme (shown at the bottom of page 109); there are no $2$-isohedral tilings which allow for any higher number of contacts between different shapes, although type $3_1 18_{12}-1\text{a}\ \text{MN}\ \text{p}6\text{m}$ also works (and can be obtained from the above construction by cutting each kite-shaped tile in two). Thus, it is maximal among $2$-isohedral tilings.
What are the maximal tilings under weaker conditions? The maximal number of sides under each successively stronger restriction is a weakly decreasing sequence which goes $\infty, \infty, ?, ?, 18$. So far, I have no bounds on the missing two terms except that they are each at least $18$.
Some notes on this problem:
It is not necessarily the case that one of the tiles may tile the plane on its own; see this math.SE question for a counterexample.
If convexity is relaxed for either piece, the number of sides is unbounded even in the $2$-isohedral case (in fact, both pieces can simultaneously have arbitrarily many sides).