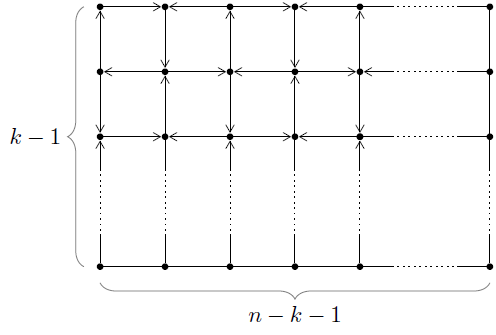
The question is why the statement in the title is true (is it?).
To elaborate, recall that Grassmannian cluster algebra, according to Scott`s paper Grassmannians and Cluster Algebras, is the cluster structure on $\mathbb{C}[\operatorname{Gr}(k,n)]$, corresponding to the following quiver, called $\Gamma_{k,n}$:
In case $k=2$ this is a chain of length $n-3$, so the corresponding cluster algebra is of type $\mathsf{A}_{n-3}$. It is also not hard to show that for $k=3$ and $n=6,7,8$ a suitable sequence of mutations transforms $\Gamma_{k,n}$ into a tree, which happens to be a Dynkin diagram of type $\mathsf{D}_4$, $\mathsf{E}_6$ or $\mathsf{E}_8$, respectively. In all other cases this cluster algebras is of infinite type.
Now I ran some computer experiments and it seems that in the infinite case the mutation class of $\Gamma_{k,n}$ contains no trees at all.
Q1: Does this experimental observation hold in all cases? If so, why?
Q2: Can one at least find some nice representative in each mutation class? Say, having the smallest number of (unoriented) cycles.