There are many identities known like
$$\sum_{k=0}^{n-1} \sin (k \cdot \theta + \varphi) = \frac{\sin\left(n \cdot \frac{\theta}{2}\right)}{\sin\left(\frac{\theta}{2}\right)} \cdot \sin \left(\frac{2 \varphi + (n-1)\cdot \theta}{2} \right)$$
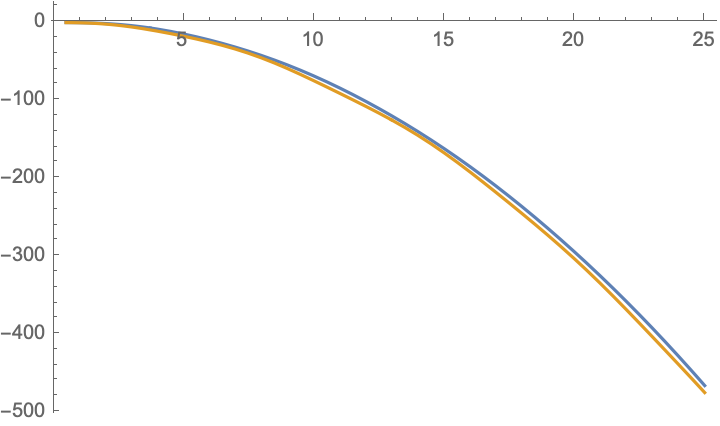
However, in such situations the angles add up and are equidistant. Instead, I pose myself the question whether there is a simplifying formula for $$\sum_{k=1}^{n} k \cdot \sin \left(\frac{x}{k} \right),$$ particularly, if $x$ is much larger than $n$.
For large $n$ you may approximate the sum by an integral, which gives $$\sum_{k=1}^{n} k \sin (x/k)-nx\simeq \int_0^\infty\bigl(k\sin(x/k)-k\bigr)\,dk=-\tfrac{1}{4}\pi x^2.$$ The plot compares the left-hand-side of this equation for $n=1000$ (blue curve) with the right-hand-side (gold) as a function of $x$.
If you rewrite everything using complex exponentials, then it's clear why a sum such as $$\sum_{k=0}^{n-1}\sin(k\theta+\phi)$$ has a nice closed formula, since it's just two geometric sums $$ e^{i\phi}\sum_{k=1}^{n-1} e^{\pm i\theta k}. $$ And if you want $\sum_{k=0}^{n-1}k\sin(k\theta+\phi)$, it's similar, there are nice formulas for $\sum_{k=1}^{n-1}kT^k$. On the other hand, the sum you're asking about looks like $$ \sum_{k=1}^n k\sin\left(\frac{x}{k}\right) = \frac12\sum_{k=1}^n \Bigl(ke^{\pi i x/k} + ke^{-\pi i x/k}\Bigr). $$ I don't think that there's a nice closed formula in general for $$ \sum_{k=1}^n kT^{1/k},\quad\text{nor for}\quad \sum_{k=1}^n T^{1/k}. $$ However, as Carlo indicated, one can often get a good approximation using an integral.
