1
$\begingroup$

Suppose we have two binomial random variables $X_i \sim B(\frac{a}{p_i},p_i)$ and $X_j \sim B(\frac{a}{p_j},p_j)$, where $a$ is a positive integer, and both $\frac{a}{p_i}$ and $\frac{a}{p_j}$ are integers. Assume $p_i > p_j$. In particular, we have $E[X_i] = E[X_j] = a$.

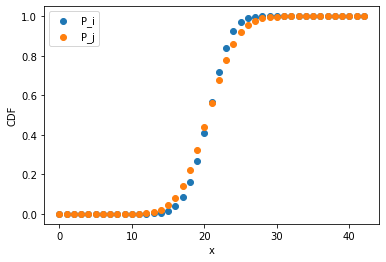
Let $F_{X_i} (x)$ and $F_{X_j} (x)$ denote the CDF's of $X_i$ and $X_j$, respectively. It seems true (with an example in the image below) that $F_{X_i} (x) < F_{X_j} (x)$ for $x < a$ and $F_{X_i} (x) > F_{X_j} (x)$ for $x > a$. Is there a simple way to prove it (or, is this result somewhat established in literature)?

Example

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ crossposted: math.stackexchange.com/q/3969967/87355 --- please don't do that, in particular not without disclosing it, to avoid wasteful duplication of efforts. $\endgroup$
    – Carlo Beenakker
    Commented Jan 2, 2021 at 13:14
  • $\begingroup$ Thanks for your response! One thing I find tricky here is that in the problem set-up $N$ actually changes with $p$ (i.e., $N = \frac{a}{p}$ for some constant $a$). Does the derivative method still apply here or maybe more tricks would be needed? $\endgroup$
    – messi22
    Commented Jan 2, 2021 at 17:39
  • $\begingroup$ @messi22: Yes, you are right, I didn't quite answer the question you asked. $\endgroup$
    – Christian Remling
    Commented Jan 2, 2021 at 18:59

1 Answer 1

Reset to default
4
$\begingroup$

This result is known. See e.g. Corollary 4 in this paper or its arXiv version.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks very much for your help! $\endgroup$
    – messi22
    Commented Jan 3, 2021 at 14:41

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .