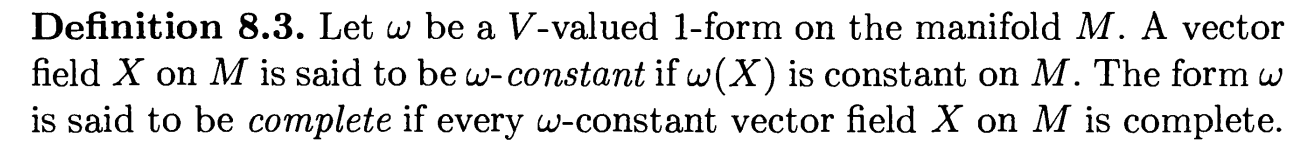In his Book Differential Geometry: Cartan's generalization of Klein's Erlangen Program, Sharpe gives the following definition of a complete 1-form:
Soon thereafter he gives the following example:
I don't understand how to conclude this from the definition. Actually, I think I have found a counterexample: Consider $M = \mathbb{R}^2$ and $N = \mathbb{R} \times \{ 0 \}$ with $$ \omega = \begin{pmatrix} \cos y \frac{dx}{1 + x^2} \\ \sin y \frac{dx}{1 + x^2} \\ dy \end{pmatrix} $$ A vector field $X$ on $M$ can only be constant if $\omega(X) \in \mathbf{e}_3 \cdot \mathbb{R}$, so it must be of the form $c \partial_y$ with $c$ constant. These vector fields are complete, so $\omega$ is complete. On the other hand, $ \omega|_N = \mathbf{e}_1 \frac{dx}{1 + x^2} $ so $(1 + x^2) \partial_x$ is $\omega|_N$-constant. But the flow of this vector field is $\phi(t) = \tan t$ which is only defined up to time $ \frac{\pi}{2} $.
Is this counterexample correct? Did I misunderstand the author? Is there some way to "fix" this result?