Let $x$ be an integer picked uniformly at random from $1 \ldots N$. Write $x = r^2 t$ where $t$ is square-free. How does the expected value of $r$ scale with $N$? Is anything known about the variance of $r$?
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
1
The asymptotic frequency of square-free integers is known to be $6/\pi^2$, see [1].
Denote by $P_n$ the uniform distribution on $[1,n]$ and by $E_n$ the corresponding expectation. Then $$E_n(r)=\sum_{k \le \sqrt{n}} k P_n(r=k) \sim \sum_{k \le \sqrt{n}} k \cdot \frac{1}{k^2} \cdot\frac{6}{\pi^2} \sim \frac{3}{\pi^2} \log(n) \,,$$ where $A \sim B$ means that $A/B \to 1$ as $ n \to \infty$. (In particular for $n=10^{10}$ the mean $E_n(r)$ is close to 7.) Also, $$E_n(r^2)=\sum_{k \le \sqrt{n}} k^2 P_n(r=k) \sim \sum_{k \le \sqrt{n}} \frac{6}{\pi^2} \sim \frac{6\sqrt{n}}{\pi^2} \,, $$ so the variance of $r$ is asymptotic to $6\sqrt{n}/\pi^2$ as well.
answered Dec 23, 2020 at 2:55
-
3$\begingroup$ I have never seen $\log_e 10 \approx \frac{7}{30}\pi^2$ being useful before: both about $2.303$ $\endgroup$– HenryCommented Dec 23, 2020 at 19:02
$\begingroup$
$\endgroup$
2
Just a little empirical data up to $N=10^{10}$, three superimposed random runs. Growing roughly linearly w.r.t. $\log_{10} N$ within that range.
answered Dec 23, 2020 at 2:54
-
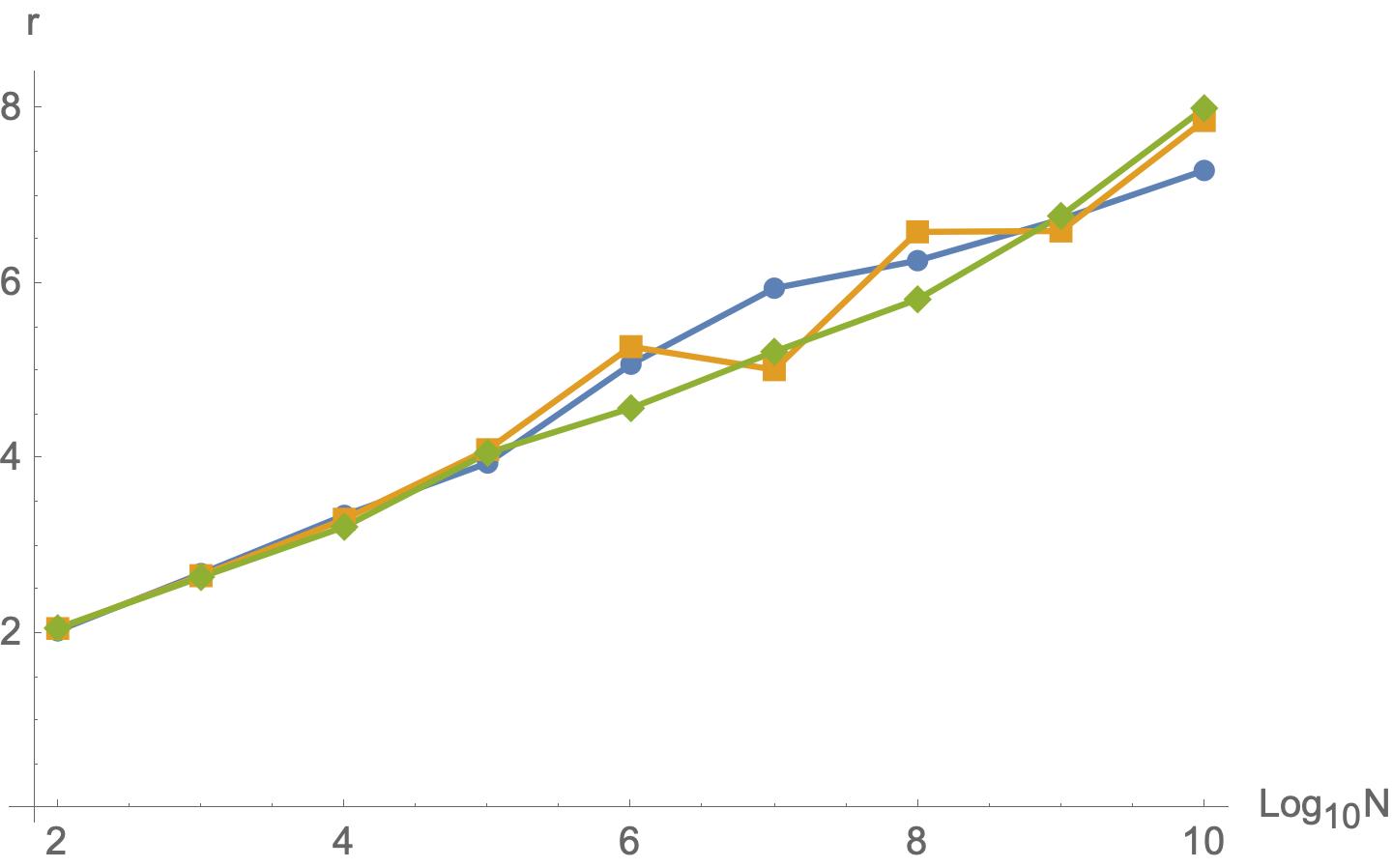
$\begingroup$ What is the slope and how close to $6/\pi^2$ is it? $\endgroup$– smciCommented Dec 23, 2020 at 10:36
-
2$\begingroup$ @smci: Slope is about $0.71$, but I plotted w.r.t. $\log_{10}$. The constant in Yuval's $E_n(r)$ is $3/\pi^2$, and $(3/\pi^2) \log(10) \approx 0.70$; so that matches. And, as Yuval says, for $N=10^{10}$, the expected value of $r$ is about $7$, which accords with my chart, and with $0.70 \times 10 = 7$. $\endgroup$ Commented Dec 23, 2020 at 14:15