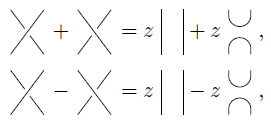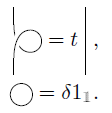5
$\begingroup$

Consider the quotient of the monoidal category of framed tangles by one of the two skein relations

Kauffman skein relations

together with the twist and dimension relations

Twist and dimension relations

Here $1_\mathbb{1}$ denotes the identity morphism of the unit object, i.e. the empty tangle, $z,t$ are invertible elements of a fixed ground ring, and $\delta = 1 - \varepsilon (t+\varepsilon t^{-1})/z$, where $\varepsilon \in \{\pm 1\}$ is the sign appearing in the chosen skein relation.

This yields two variants of the Kauffman skein category. The choice $\varepsilon=1$ leads to the Kauffman polynomial of links, while the choice $\varepsilon=-1$ leads to the Dubrovnik polynomial. Provided the ground ring contains a square root of negative one, these two link polynomials are equivalent (i.e. one can be obtained from the other by rescaling $z,t$ and an overall scaling). More generally, the endomorphism algebras of the two categories are isomorphic after a rescaling of $z,t$, and they are isomorphic to BMW algebras. (See Lemma 8.5 of this paper for the precise statement.) For this reason (I think), the difference between the two skein relations above is often overlooked, and authors choose the one that best suits their needs.

However, one can show that the two categories described above are not isomorphic as monoidal categories, even after rescaling parameters. (See Proposition 8.6 of this paper for a proof.) For the choice of $\varepsilon=-1$, there is a natural functor from the above category to categories of modules for quantized enveloping algebras of type $B,C,D$. I'd like to know if there is a similar action of the category for the choice $\varepsilon=1$. I haven't been able find anything about this in the literature, which surprises me since these categories are fairly well known and well studied.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

The Dubrovnik normalization is the "right" one for quantum groups for the simple reason that it deforms the symmetric (over=under) skein relation which is what holds for ordinary groups. That is, you can easily see that in the ordinary group case there's a unique relation between the three diagrams and it is over-under = 0, so it can take a functor from Dubrovnik, but can't take a functor from Kauffman.

You might be interested in the "recognition theorems" 3.1 and 3.2 from our paper with Scott and Emily which gives a very quick test for exactly when a ribbon category takes a functor from each of these categories. (These results weren't really due to us, we just nailed them down from existing stuff in the literature.) Section 1.1.4 also has careful statements of the relationship between Dubrovnik and BCD quantum group categories, note in particular that for type C the functor isn't ribbon if you use the usual ribbon structure on the quantum group side.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I understand why the Dubrovnik normalization is the natural one for quantum groups (for the reason you mention). But I'm somehow still unsatisfied that there isn't some nice categorical action (on something else) for the Kauffman normalization. $\endgroup$
    – Alistair Savage
    Commented Dec 16, 2020 at 20:28
  • $\begingroup$ Is your question whether there's some Hopf algebra in the Kauffman case? That is, whether there's some forgetful functor from the Kauffman category to vector spaces? $\endgroup$
    – Noah Snyder
    Commented Dec 16, 2020 at 21:21
  • $\begingroup$ Yes, that's probably a more precise formulation of what I'm looking for. I'd like a (nontrivial) functor to the category of modules over some Hopf algebra. $\endgroup$
    – Alistair Savage
    Commented Dec 16, 2020 at 21:26
  • $\begingroup$ I'm posting this as a comment rather than an answer because I haven't taken the time to investigate the statement fully enough to give a full answer. My understanding is that the Kauffman polynomial captures the SO(N) Chern-Simons invariants in the analogous way that the Homflypt polynomial captures the SU(N) invariants. In particular, I would venture a guess that a certain specialization of your Kauffman case will admit a functor to the subcategory of Rep_q(SL_2) where you have even weights, mapping the monoidal generator to the adjoint rep instead of the defining rep. $\endgroup$
    – David Jordan
    Commented Dec 17, 2020 at 16:17
  • $\begingroup$ It is unfortunately hard to find good references about the above statement, I did find SKEIN RELATIONS AND WILSON LOOPSIN CHERN—SIMONS GAUGE THEORY James H. HORNE, which states the above folklore, although I didn't neatly match the formulas to yours. Perhaps mathscinet search of papers citing that one will yield hits. $\endgroup$
    – David Jordan
    Commented Dec 17, 2020 at 16:19

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .