2
$\begingroup$

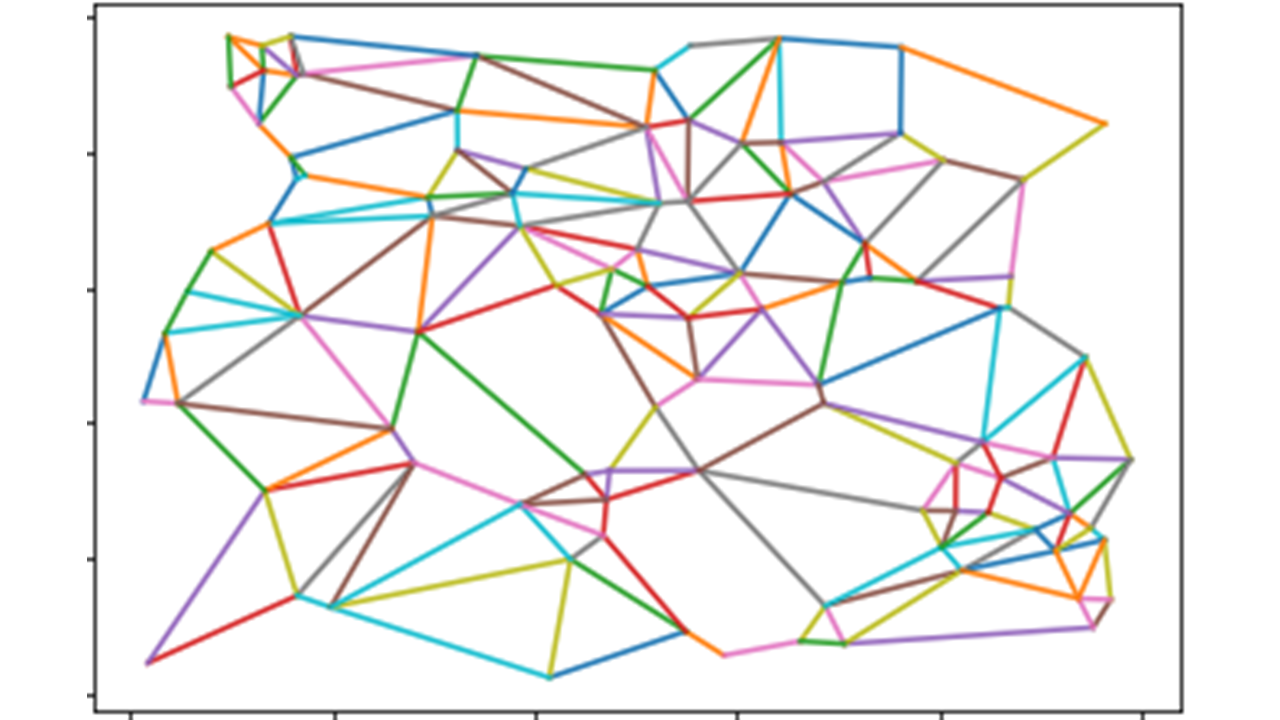
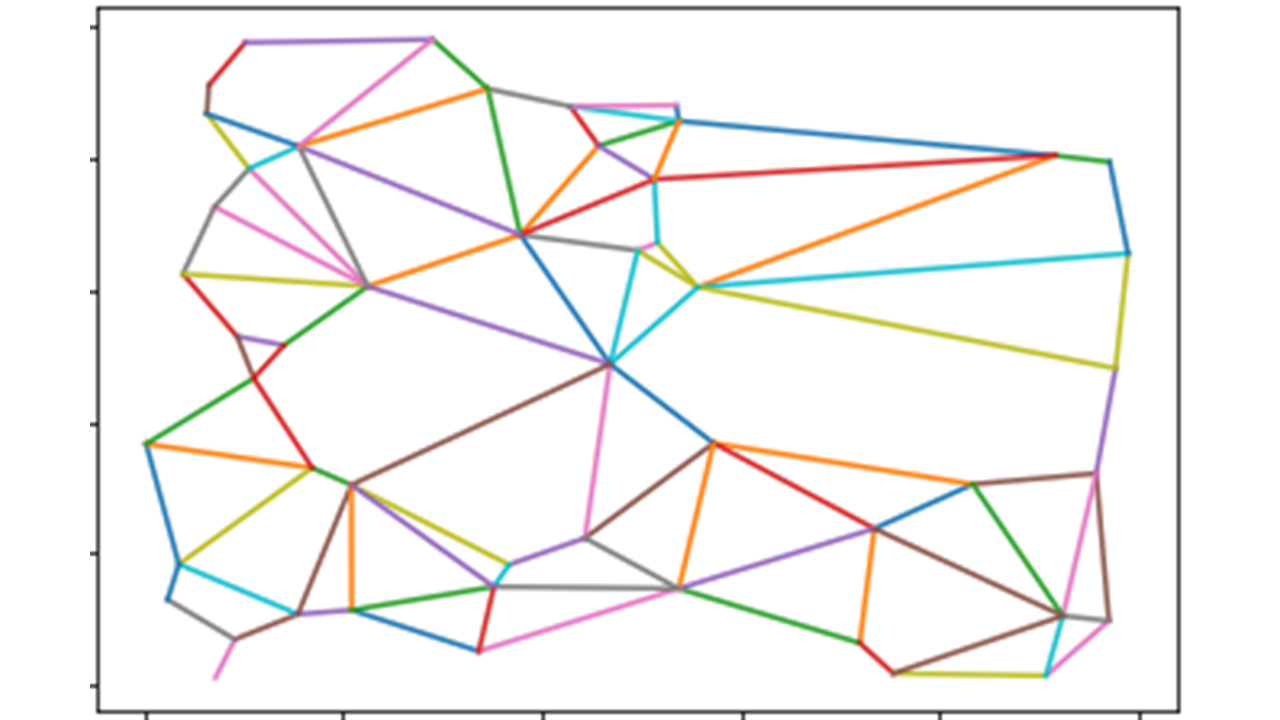
I have implemented an algorithm that filters the edges of simple complete graph with weighted edges according to a criterion that is inspired by elementary planar geometry and, to my surprise,

Biconnected spanner of planar pointset

in the majority of cases it produces a biconnected planar graph with convex regions,

Non-biconnected spanner of planar pointset

but sometimes there are also vertices of degree $1$; as can be seen in the lower left corner.

I would now like to know whether it would be mathematically correct, to speak of the union of line-segments, that constitute to the boundary of the outer face, as a hull of the planar points that defined the graph. Especially in the case where the generated graph isn't biconnected I'm not sure if it is justified to speak of a hull in that case.


Question:

what is the most fundamental definition of the term "hull" in mathematics, when fundamental definition shall mean "expressed in the language of the foundations of mathematics", e.g. in the language of set-theory, categories or whatever may serve as a fundament on which mathematics can be based.

Improve this question
$\endgroup$
4
  • 2
    $\begingroup$ The pictures and allusions to your algorithm don't seem to be terribly relevant to your actual question. In general "X hull of Y" seems to be used to mean "simplest/best thing with property X that contains Y" (see en.wikipedia.org/wiki/Hull). However, I don't think it's reasonable to expect that there's a single, rigorous, universal definition of "hull" from which all others can be derived (and attempting to formulate one would probably lead to something so clumsy as to be virtually useless). $\endgroup$
    – David Loeffler
    Commented Nov 15, 2020 at 10:22
  • $\begingroup$ While there is no universal definition, in accordance with common terminology I’d expect the “hull of the planar points that ...” to include all those points, and then some. (Whatever the “hull” is, it should behave as a closure operator.) Thus, I don’t think this is a good choice of a term. If I understand correctly your definition, I’d use a word like “boundary”, “border”, or “front”. $\endgroup$
    – Emil Jeřábek
    Commented Nov 15, 2020 at 10:43
  • $\begingroup$ @EmilJeřábek so the term "hull" would require biconnectivity of the graph? Then addig the shortest edge(s) that restore biconnectivity would yield something that can serve as (shape) hull? $\endgroup$
    – Manfred Weis
    Commented Nov 15, 2020 at 10:56
  • $\begingroup$ No, it would require that the hull includes all of the original graph rather than just the boundary. $\endgroup$
    – Emil Jeřábek
    Commented Nov 15, 2020 at 12:11

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .