About the dual of the cube lemma in homotopy theory

Question

Consider the Reedy category $2\rightarrow 1 \leftarrow 0$. Consider a map of diagrams of topological spaces $D\to E$ over this Reedy category:

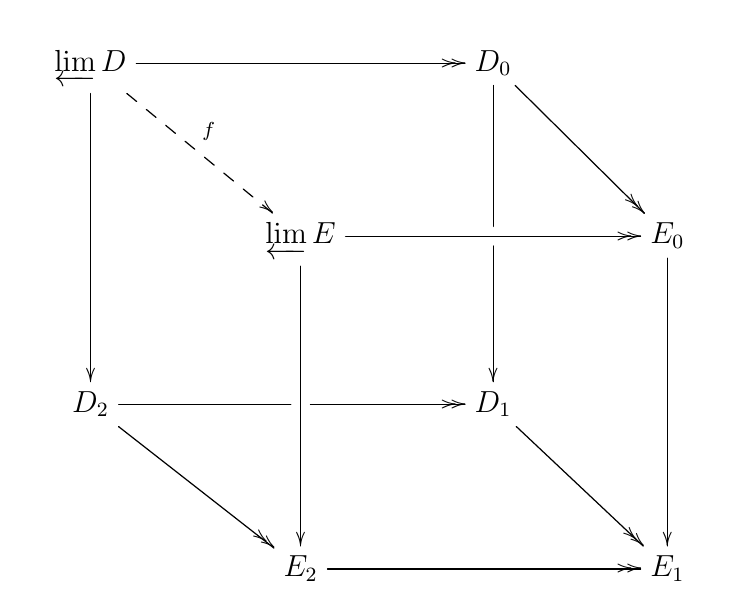
The maps which are fibrations are depicted with the symbol $\twoheadrightarrow$: the map of diagrams $D\to E$ is a pointwise fibration, and moreover the maps $D_2\to D_1$ and $E_2\to E_1$ are fibrations as well. This implies that the two diagrams $D$ and $E$ are Reedy fibrant. We suppose moreover that the map $D_2 \twoheadrightarrow E_2 \times_{E_1} D_1$ (not depicted in the image) is a fibration as well. Therefore the map of diagrams $D\to E$ is a Reedy fibration. For the Reedy model structure, the inverse limit is a right Quillen functor. This implies that the map $f$ from the inverse limit of D to the inverse limit of E is a fibration.

Here is now the question.

With these hypotheses, is it sufficient to conclude that

$$\varprojlim D \longrightarrow \varprojlim E \times_{E_0} D_0$$

is a fibration as well ? If not, what is missing ?

Answer 1

Yes, $D_0 \times_{D_1} D_2 \to (E_2 \times_{E_1} E_0) \times_{E_0} D_0$ is a fibration.

First, observe that $$(E_2 \times_{E_1} E_0) \times_{E_0} D_0 \cong E_2 \times_{E_1} D_0 \cong (E_2 \times_{E_1} D_1) \times_{D_1} D_0$$ by the pullback pasting lemma. Also, $$D_2 \times_{D_1} D_0 \cong D_2 \times_{E_2 \times_{E_1} D_1} ((E_2 \times_{E_1} D_1) \times_{D_1} D_0)$$ but we assumed that $D_2 \to E_2 \times_{E_1} D_1$ is a fibration, so $$D_2 \times_{E_2 \times_{E_1} D_1} ((E_2 \times_{E_1} D_1) \times_{D_1} D_0) \to (E_2 \times_{E_1} D_1) \times_{D_1} D_0$$ is also a fibration. But this is isomorphic to $D_0 \times_{D_1} D_2 \to (E_2 \times_{E_1} E_0) \times_{E_0} D_0$ so we are done. (So, in fact, we don't need to assume anything else is a fibration.)

I apologise for the lack of diagrams. I think it is easier to understand if you draw them yourself.