22
$\begingroup$

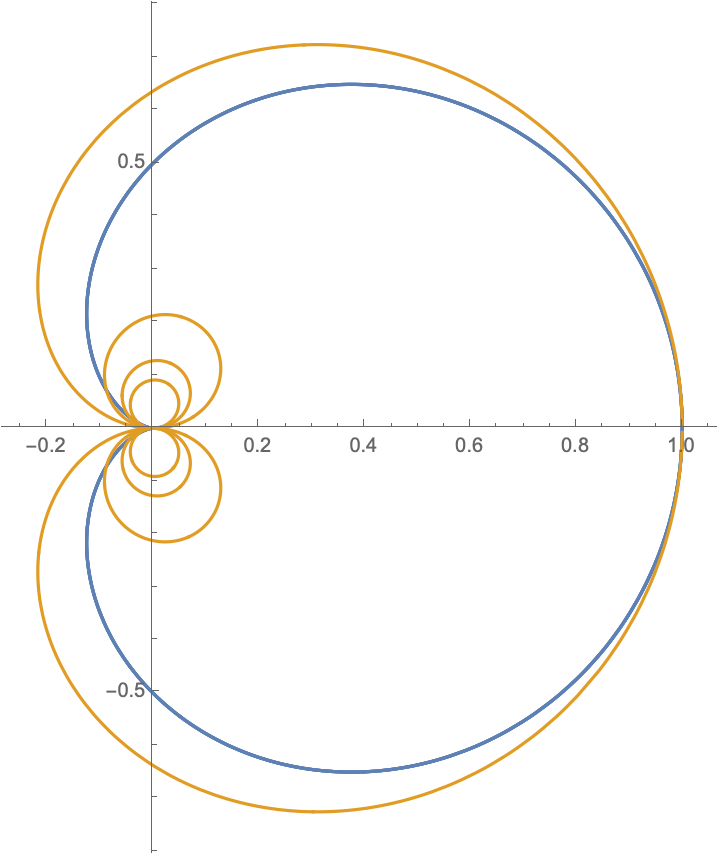
The curve, given in polar coordinates as $r(\theta)=\sin(\theta)/\theta$ is plotted below. cardioid-looking curve

This is similar to the classical cardioid, but it is not the same curve (the curve above is not even algebraic, I believe). Does this curve have a name? Does it show up somewhere? This curve has the property that it solves $\mathrm{Im}(1/z+\log(z))=0$, if this perhaps rings a bell.

This particular curve arises in some research I am working on at the moment, and it would be great if it perhaps connects to some classical area.

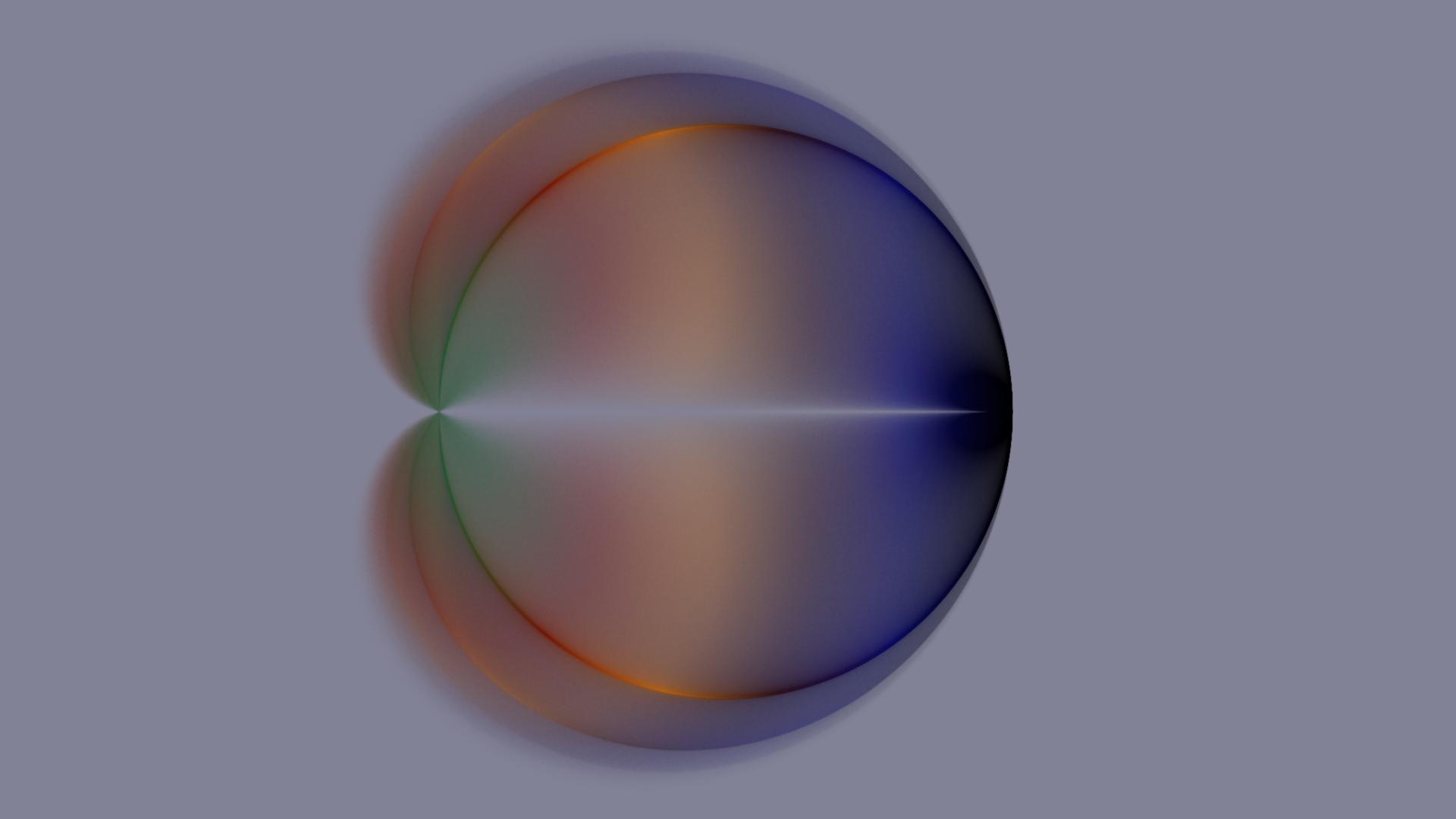
Edit: Thanks for the great references! As a reward, here is a more artistic rendering of the shape using a type of complex dynamical systems. Nice cardiodid

Improve this question
$\endgroup$
5
  • 9
    $\begingroup$ Check mathcurve.com. At least 100 named curves. $\endgroup$
    – skbmoore
    Commented Sep 15, 2020 at 20:17
  • $\begingroup$ That was a great resource! $\endgroup$
    – Per Alexandersson
    Commented Sep 15, 2020 at 20:30
  • 4
    $\begingroup$ For people too lazy to type, and because I like the enthusiasm of the name, a clickable version of @skbmoore's link: Encyclopédie des formes mathématiques remarquables. $\endgroup$
    – LSpice
    Commented Sep 15, 2020 at 20:38
  • $\begingroup$ It seems to be a limacon. $\endgroup$
    – user165496
    Commented Sep 17, 2020 at 10:59
  • 2
    $\begingroup$ @user165496 --- the limaçon has a different equation, in particular it lacks the infinite series of windings that the cochleoid has: en.wikipedia.org/wiki/Limaçon $\endgroup$
    – Carlo Beenakker
    Commented Sep 17, 2020 at 11:55

3 Answers 3

Reset to default
36
$\begingroup$

The name of the curve is cochleoid (= shell-shaped rather than cardioid = heart-shaped).

I compare the two below (gold = cochleoid, blue = cardioid). The distinction shell/heart refers to the additional windings remarked upon by მამუკა ჯიბლაძე , without these windings the two shapes would be qualitatively the same.

Improve this answer
$\endgroup$
1
33
$\begingroup$

Not an answer - just want to note that the curve has more hidden branches. They can be seen looking at the parametric equation $$ x=\frac{\sin(\varphi)}\varphi,\quad y=\frac{1-\cos(\varphi)}\varphi $$ where $\varphi=2\theta$

enter image description here

Improve this answer
$\endgroup$
2
$\begingroup$

From what LSpice and skbmoore shared https://mathcurve.com/courbes2d/cochleoid/cochleoid.shtml, There's this illustration of a helix on a cylinderenter image description here The picture in the middle is the view when lined up with the side of the cylinder. If I understand the site correctly, the line traced by the helix in this perspective is a cochleoid.

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ This is indeed true: say,$$x=t+\cos(t),y=-\sin(t),z=1+t$$is a helix, and projecting it from the point $(1,0,1)$ to the plane $z=0$ gives$$(\frac{1-\cos(t)}t,\frac{\sin(t)}t,0).$$Explicitly, to project you must send $(x,y,z)$ to $((x-z)/(1-z),y/(1-z),0)$; then just substitute the above parametric expressions for $t$. $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Sep 18, 2020 at 8:59

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .