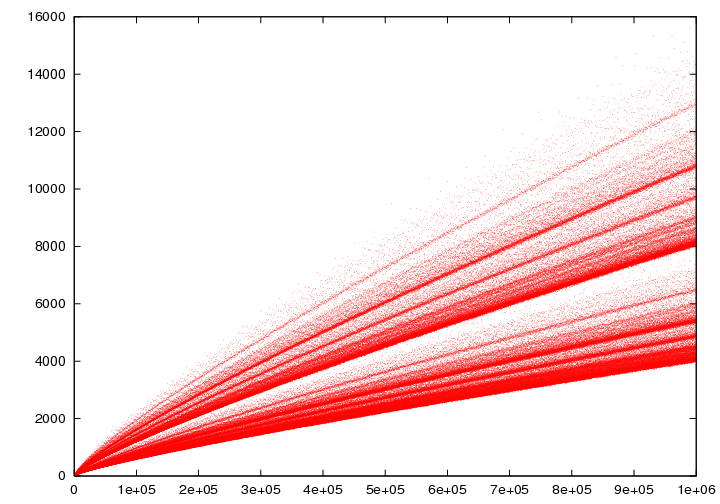
Let $g(2n)$ be the number of representations of $2n=p+q$ with primes $p$ and $q$. Many people have asked whether $g(2n) \ge 2$ when $2n = p+q$ for some primes $p$ and $q$. That is, does $g(2n) \ge 1$ imply $g(2n) \ge 2$? From the famous Goldbach Comet, it looks probable although it was not yet proved.
Now, what can we say about the following weaker problem?
For any sufficiently large prime $p$, is there a prime $q$ such that $p+q$ has another representation $p' + q'$?
To your first question: we don't know. To your second question: we know much more, namely if $N$ is a large odd number, then the number of representations $N=p_1+p_2-p_3$ with each $p_j$ a prime from $[2N,3N]$, has order of magnitude $N^2/(\log N)^3$. This can be proved in essentially the same way as we prove that $N$ can be written as a sum of three primes in that many ways. See also Harald Helfgott's response here.
Here is a graph showing the number of representations of $2n$ as a sum of two primes.
It suggests that something much stronger than what you ask about is true. And there are heuristics that predict what is shown. but not proofs.
Let $n \in 2\mathbb{N}^*$ large enough.
$$n = p+q, \ (p,q)\in\mathbb{P}^2 \iff (p, n-p) \in \mathbb{P}^2$$
You search for the quantative version of Goldbach's conjecure, Hardy and Littlewood in there 1923 paper "Some problems of ‘Partitio numerorum’; III : On the expression of a number as a sum of primes", conjecture that : $$G(n) \sim 2 C_2 \displaystyle {\small \Big( \prod_{\substack{p | n \\ \text{p prime} \\ 3 \leqslant p}} {\normalsize \dfrac{p-1}{p-2}} \Big)} \dfrac{n}{\log(n)^2}.$$
Where $G(n) = \#\{(p, n-p) \in \mathbb{P}^2 \, | \, p \leqslant n\}$, and : $C_2 = \displaystyle{\small \prod_{\substack{3 \leq p \\ \text{p prime}}} \left({\normalsize 1-\dfrac{1}{(p-1)^2}}\right)}$.
This conjecture agree perfectely with numeric checks, but unfortunately not proven up to now (and no hope to prove it soon).
You can see my try here : is there a link with the probabilistic model for prime numbers?
