3
$\begingroup$

Given $u\in\mathbb{C}$ and $v\in\mathbb{C}$ let's consider the following progression: $$z_n=u+nv\;\;\;\;\;\;\;\;\;n\ge 0$$

Is it possible to find progressions $z_n$ generating gaussian primes for an arbitrary long sequence of consecutive values of n?

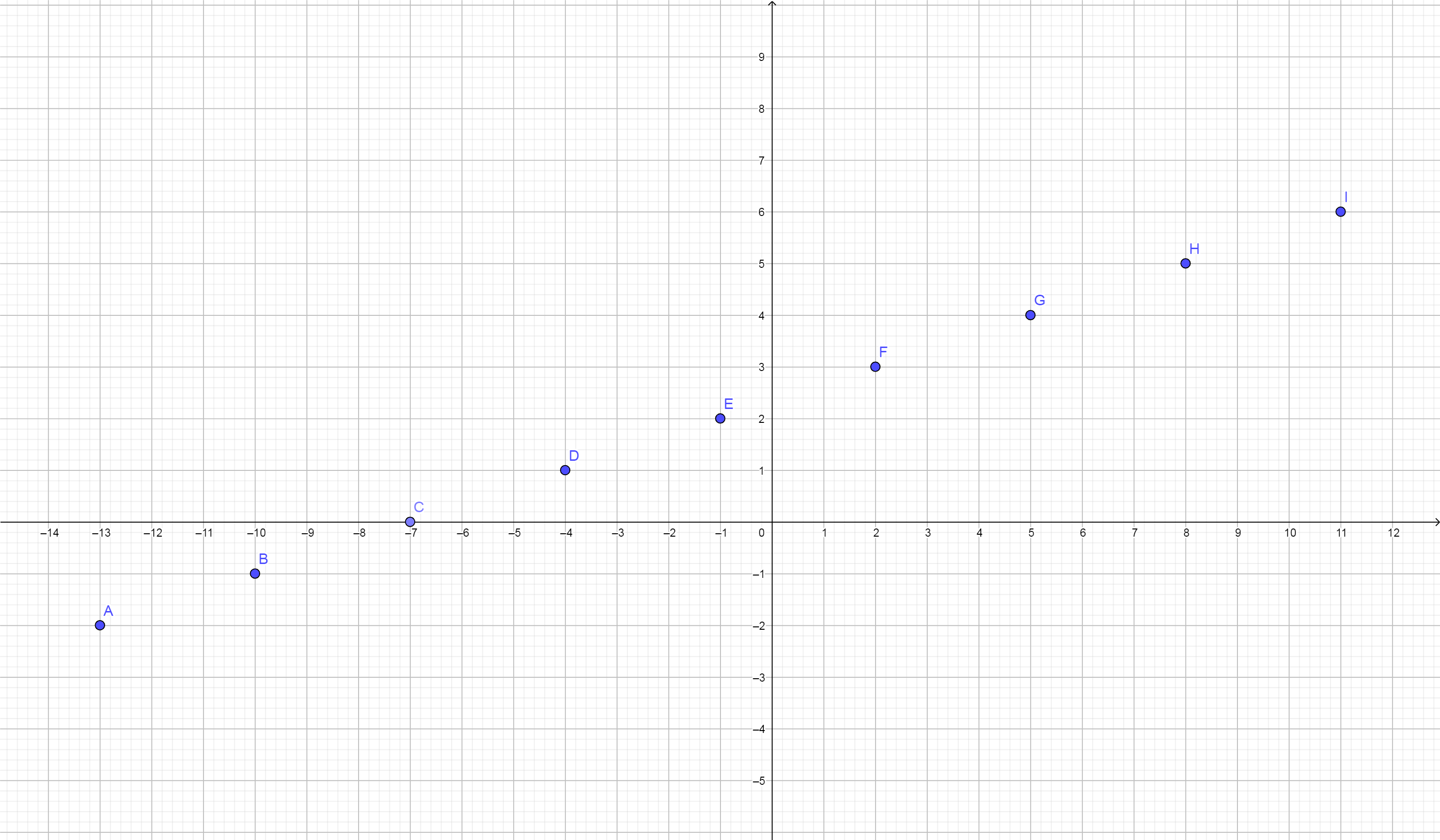
For example, $z_n=-13-2i+n(3+i)$ generates gaussian primes for all values $0\le n\le 8$ (examine the norm $|z_n|^2=10n^2-82n+173$):

enter image description here

If not, it is known the progression of maximum lenght?

Many thanks.

Improve this question
$\endgroup$
6
  • $\begingroup$ @StanleyYaoXiao Your claim about Gaussian primes is false (3 is a Gaussian prime, but its norm is 9). $\endgroup$
    – David Loeffler
    Commented Aug 20, 2020 at 19:48
  • $\begingroup$ @DavidLoeffler I was working under the supposition that only primes that split in $\mathbb{Z}[i]$ count as "Gaussian primes", which I believe is intended by the question (typically the expression $u + nv$ given in the question will never equal a rational integer). I can edit the comment to clarify this $\endgroup$
    – Stanley Yao Xiao
    Commented Aug 20, 2020 at 19:50
  • $\begingroup$ Since a Gaussian integer $x = a + ib$ with $ab \ne 0$ is prime if and only if its norm is a rational prime, your question is equivalent to asking for fixed Gaussian integers $u,v$ whether exist infinitely many rational integers $n$ such that the expression $|u|^2 + 2n\Re(u\overline{v}) + n^2 |v|^2$, which is a quadratic polynomial in $n$ with rational integer coefficients, is prime infinitely often. There is no irreducible quadratic polynomial for which we know the answer. $\endgroup$
    – Stanley Yao Xiao
    Commented Aug 20, 2020 at 19:51
  • $\begingroup$ Your assertion is still false -- what about 3i? Moreover, where in the question is it specified that $u, v$ can't be rational integers? $\endgroup$
    – David Loeffler
    Commented Aug 20, 2020 at 19:53
  • $\begingroup$ @DavidLoeffler it doesn't, but inferring from the example given and the assumption that the OP understands how to count arithmetic progressions in the rational primes (which is what one gets if $u,v$ are co-prime rational integers and $n$ a rational integer parameter), I believe it is reasonable to assume that $u,v$ are not rational integers nor of the form $\pm ix$ for rational integral $x$. $\endgroup$
    – Stanley Yao Xiao
    Commented Aug 20, 2020 at 19:57

2 Answers 2

Reset to default
6
$\begingroup$

The Green--Tao theorem also shows that there are arbitrarily long arithmetic progressions among the (rational) primes that are congruent to 3 modulo 4. See for instance this MO question Is the Green-Tao theorem true for primes within a given arithmetic progression?.

Since any rational prime that is 3 mod 4 is a Gaussian prime, this shows that the Gaussian primes contain arbitrarily long arithmetic progressions.

(This is perhaps a slightly unsatisfactory class of examples. I don't know if there are arbitrarily long arithmetic progressions of primes in $\mathbf{Z}[i]$ which aren't in $\mathbf{Z}$ or $i \mathbf{Z}$.)

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ A theorem of Tao arxiv.org/abs/math/0501314 says: given any finite sets of points $v_i \in \mathbb{Z}[i]$ there are infinitely many $a \in \mathbb{Z}[i], r \in \mathbb{Z}\setminus \{0\}$ such that all $a+rv_i$ are Gaussian primes. Choosing a shape of two parallel lines, say $v_{1,j}=j, v_{2,j}=i+j, j\in \{1, \ldots , k\}$, shows that there are also long progressions of Gaussian primes not all on the real line, which answers the question left open by David. One could also take lines, say, with 45 degrees angle. $\endgroup$
    – Christian Elsholtz
    Commented Aug 21, 2020 at 7:28
  • 2
    $\begingroup$ You should post that as an answer, it's great $\endgroup$
    – David Loeffler
    Commented Aug 22, 2020 at 8:40
2
$\begingroup$

A theorem of Tao arxiv.org/abs/math/0501314 says: given any finite sets of points $v_i \in \mathbb{Z}[i]$ there are infinitely many $a\in \mathbb{Z}[i],r\in \mathbb{Z}\setminus \{0\}$ such that all $a+rv_i$ are Gaussian primes. Choosing a shape of two parallel lines, say $v_{1,j}=j,v_{2,j}=i+j,j\in \{1, \ldots,k\}$, shows that there are also long progressions of Gaussian primes not all on the real line, (which also answers the question left open by David). One could also take lines, say, with 45 degrees angle.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .