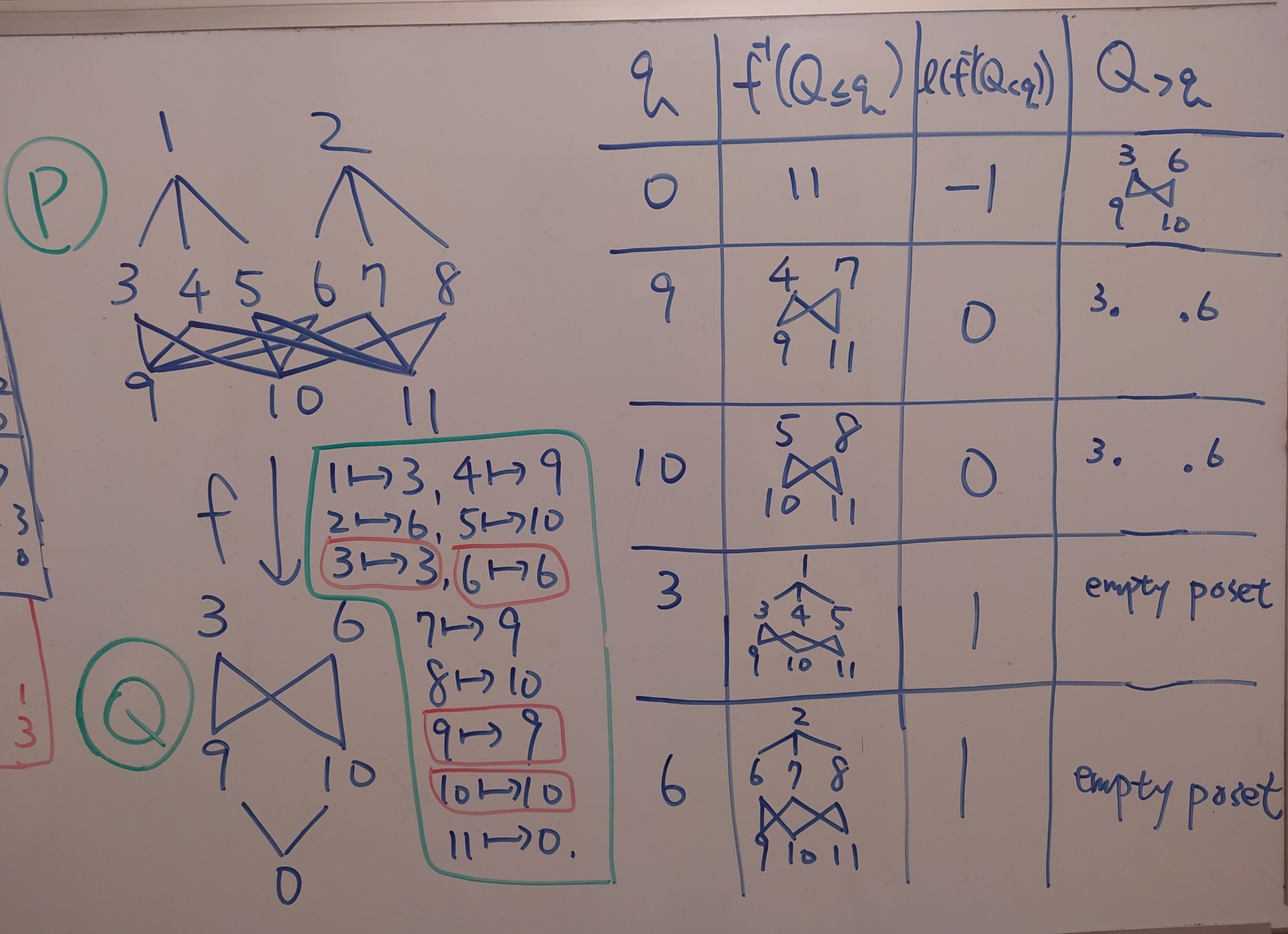
I am trying to implement Theorem 1.1 in the paper "Poset Fiber Theorems" by Bjorner, Wachs, and Welker. https://www.researchgate.net/publication/228782786_Poset_fiber_theorems
I am pretty excited to learn about the theorem, which is a generalization of the well-known Quillen fiber lemma and should be very useful for computation. But when I implement the theorem to the following example, the result seems inconsistent with my knowledge:
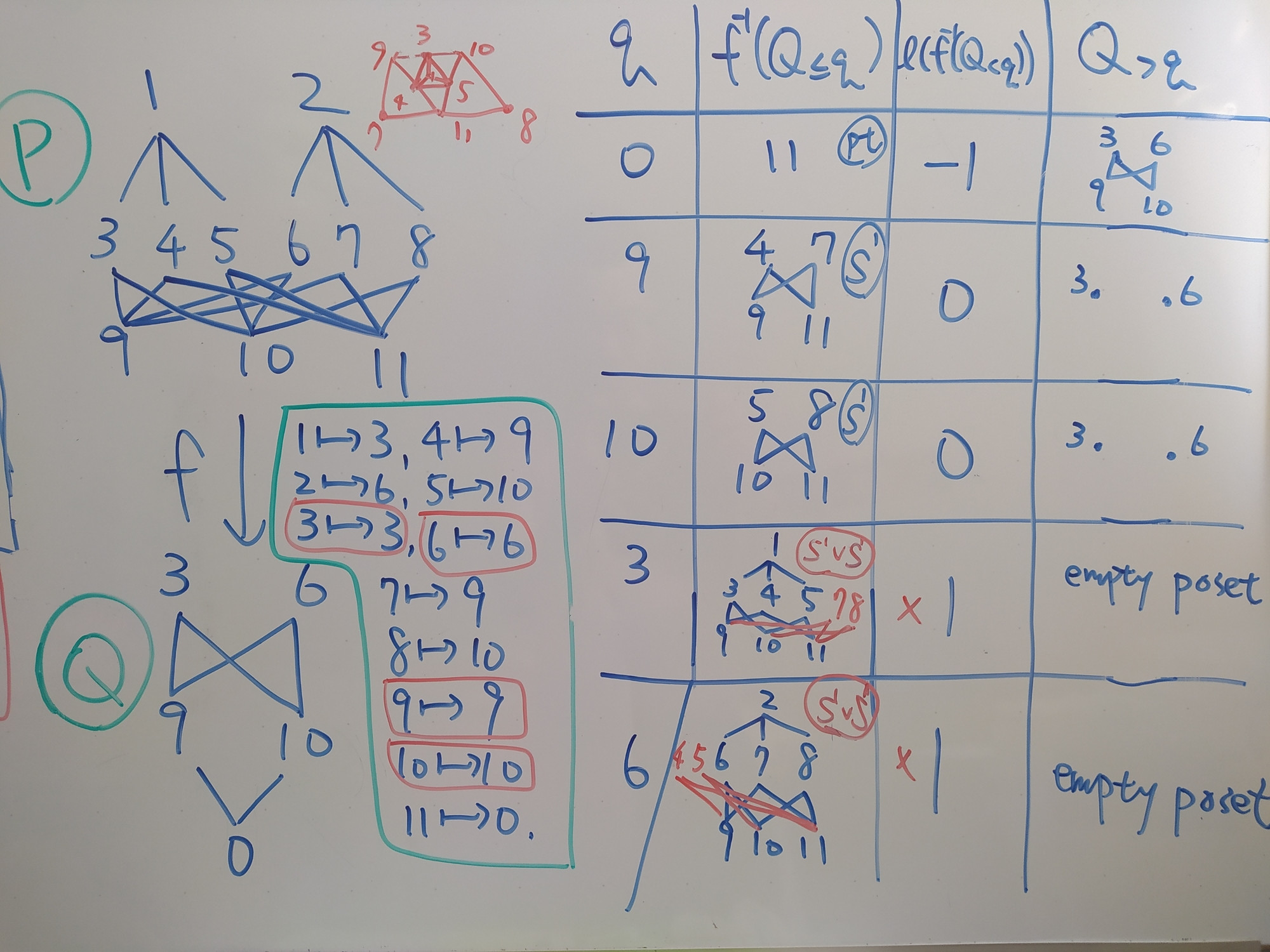
The posets are as shown in their Hasse diagrams and how the map is defined is: fixing $3,6,9,10$, mapping $11$ to a new minimum point $0$, and mapping other points downward by 1 level to the unique choice in $\{3,6,9,10\}$. From the construction, it is order-preserving and hence a poset map.
The conditions of Theorem 1.1 are satisfied as shown in the table on the right. Since $Q$ is the cone over the order complex $\Delta(\{3,6,9,10\})$, it is contractible. Thus, applying Theorem 1.1 in the paper, $\Delta(P)$, the order complex of $P$, is homotopic equivalent to a wedge of $\Delta(f^{-1}(Q_{\leq q}))\star\Delta(Q_{>q})$, where $\star$ denotes the join operation.
I am only interested in the homology group over fields, so I will use Betti numbers for the rest of the discussion. I also use Kunneth's formula for the join of simplicial complexes (see, e.g., the very beginning of https://arxiv.org/abs/math/0412552) in the case of field coefficients:
For $q = 0$, the reduced Betti numbers for $\Delta(f^{-1}(Q_{\leq q}))\star\Delta(Q_{>q})$ are all zeros.
For $q = 9$ or $10$, the reduced Betti numbers are $0,0,1$ (i.e. $H_k$ for $k = 0, 1, 2$).
For $q = 3$ or $6$, $f^{-1}(Q_{\leq q})$ is isomorphic to the face poset of a $2$-simplex. Hence, the reduced Betti numbers of $\Delta(f^{-1}(Q_{\leq q}))\star\Delta(Q_{>q})$ are all zeros.
Thus, by Theorem 1.1 in the paper, the reduced Betti numbers of $\Delta(P)$ are 0, 0, 2 (i.e. $H_k$ for $k = 0, 1, 2$) and $0$ for other dimensions.
However, it can be noted that $P$ is isomorphic to the face poset of the CW complex obtained by taking two copies of a $2$-simplex and identifying only their corresponding vertices. (You may visualize it as a pair of panties.) Thus, the reduced Betti numbers are 0, 2, 0 (i.e. $H_k$ for $k = 0, 1, 2$) and $0$ for other dimensions. I also verify the result by computer.
I have been checking logical bugs the whole day and cannot find a mistake. I am relatively new to these poset topology things and afraid that I have made some naive/stupid mistakes. Any comments, questions, suspicion about my computations, or pointing out my mistakes are welcome. Thank you.