4
$\begingroup$

$\DeclareMathOperator\Spin{Spin}\DeclareMathOperator\SL{SL}\DeclareMathOperator\SU{SU}$We know that $$ \Spin(1,3)=\SL(2,\mathbb C) $$ and $$ \Spin(4)=\SU(2) \times \SU(2). $$

The $\Spin(1,3)$ is the Lorentz version of Spin group, while $\Spin(4)$ is the Euclidean version of Spin group.

The spinor representations of $\Spin(4)$ is essentially the spinor representation of $\Spin(4)=\SU(2) \times \SU(2)$ thus it is labeled by two components of spinors, each for the spinor representation of $\SU(2)$.

  • How do we understand the spinor representations of $\Spin(1,3)$? Note that $\Spin(1,3)$ is non-compact. How is this $\Spin(1,3)$ spinor related to the spinor of the compact group $\Spin(4)$?

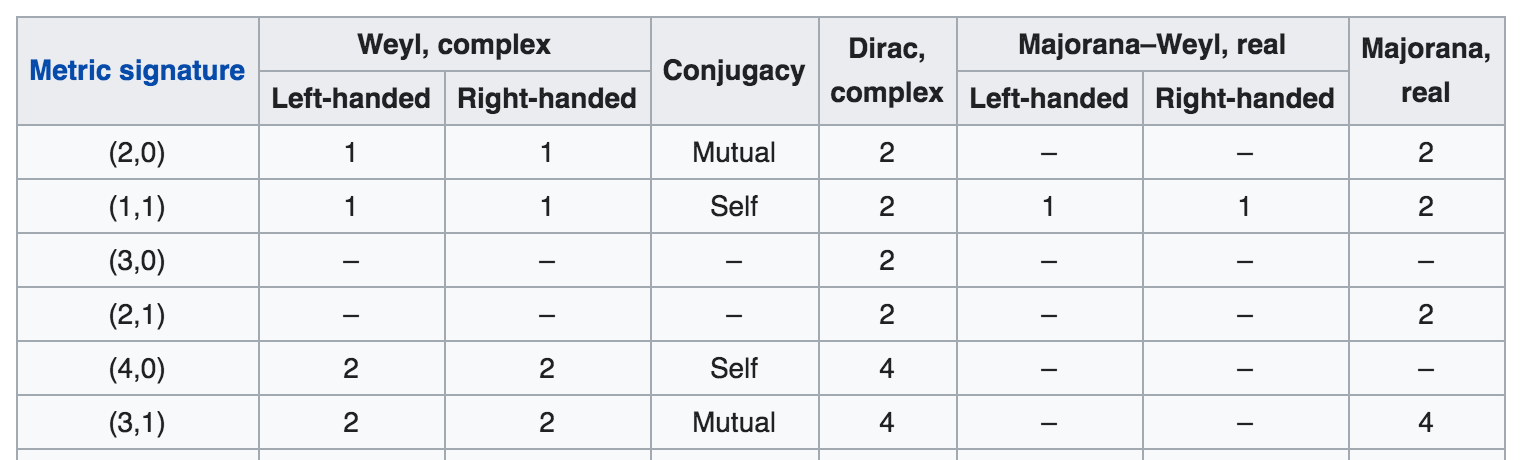
  • How do we understand the 2 dimensional spinor as complex Weyl and 4 dimensional spinor as complex Dirac but NO 4 dimensional real Majorana in $\Spin(4)$?

  • It is also said $\Spin(1,3)$ is the complexification of $\SU(2)$. How to see this from spinor representation living in the vector spaces?

  • How do we understand the 2 dimensional spinor as complex Weyl and 4 dimensional spinor as complex Dirac or real Majorana in $\Spin(3,1)$?

See https://en.wikipedia.org/wiki/Spinor#Spinors_in_representation_theory

enter image description here

Refs from google search:

http://www-personal.umich.edu/~williams/notes/spinor.pdf http://scipp.ucsc.edu/~haber/ph251/Spinor_Shijun https://en.wikipedia.org/wiki/Complexification_(Lie_group)

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Of your three questions I understand only the first one. I understand the two "spinor" representations of the group $G={\rm SL}(2,{\Bbb C}) $ (regarded as a *real* algebraic group) as the two 2-dimensional complex representations $$\rho,\bar\rho\,\colon G\to {\rm GL}(2,{\Bbb C})$$ given by the formulas $\,\rho(g)=g\,$ and $\,\bar\rho(g)=\bar g$, where the bar over $g$ denotes the complex conjugation. $\endgroup$
    – Mikhail Borovoi
    Commented Jul 27, 2020 at 17:52

0

Reset to default

You must log in to answer this question.