Non-degenerate simplexes in a Kan complex

Question

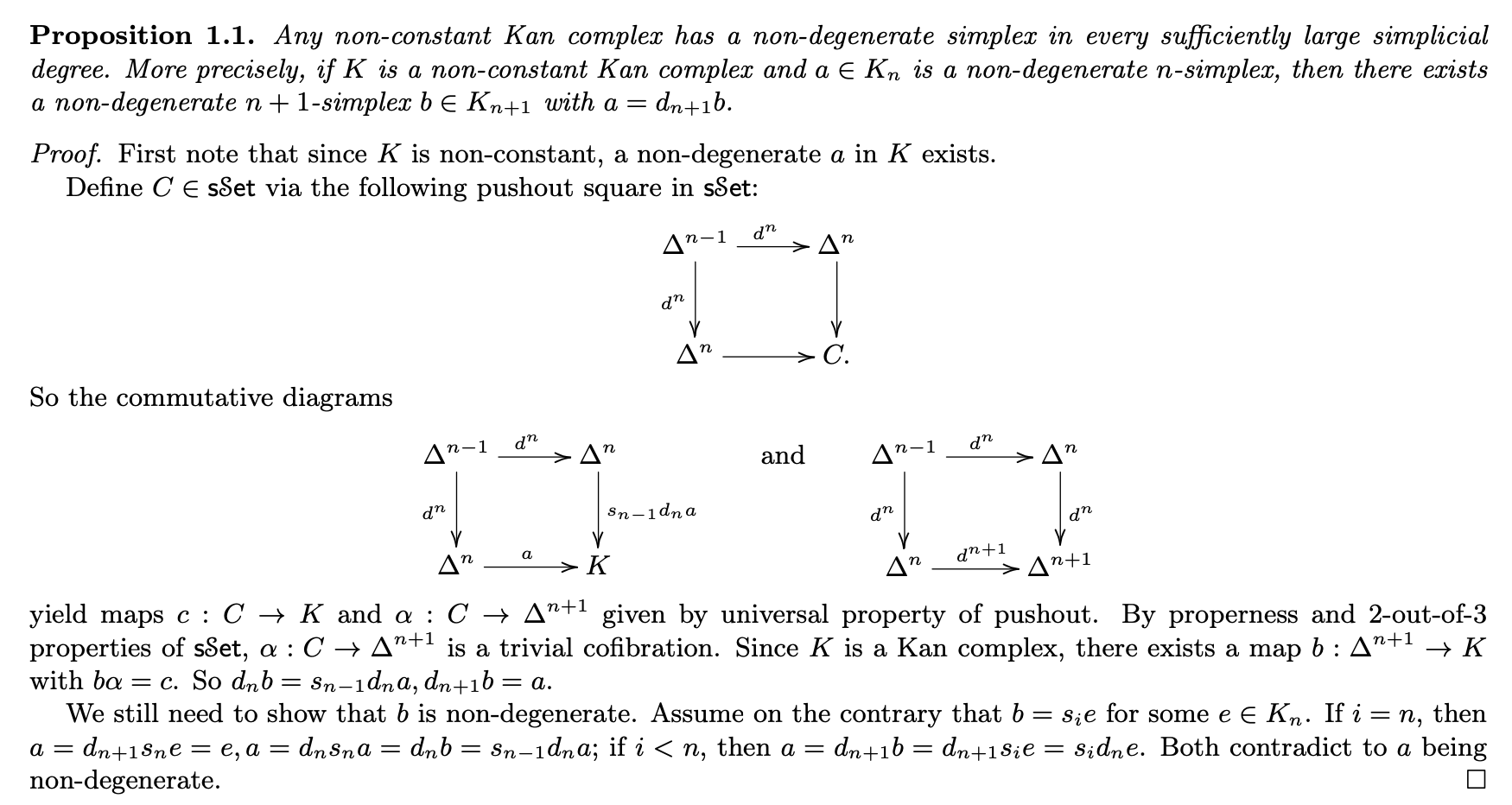
I have the following question on simplicial sets:

a non-constant Kan complex has a non-degenerate simplex in every sufficiently large simplicial degree?

It's Exercise 8.2.3 (p. 262) of Charles Weibel's book An Introduction to Homological Algebra. In fact the original question is not like this, but Weibel's errata list here http://www.math.umd.edu/~jmr/602/bookerrors.pdf has p.262 line -13: ‘every n’ should be ‘every sufficiently large n’. One may need to (admit and) use the fact that the standard simplices $\Delta^n\ (n>0)$ are not Kan complexes, being the first half of Exercise 8.2.3.

I've no idea about how to prove it and I've not seen this kind of result in standard books on simplicial sets like in May or Goerss-Jardine. I don't really need it but it might be useful, for example, using this, one can see that a non-constant finite simplicial set could not be a Kan complex. So I will be happy to see a solution for this.

Answer 1

As suggested in Tom Goodwillie's comment, I'll prove that if $f$ is a non-degenerate $n$-simplex in a Kan complex $X$ for $n>0$, then there exists a non-degenerate $(n+1)$-simplex $g$ such that $d_{n+1}g = f$.

Let $f: \Delta^n=\Delta^{\{0, \ldots, n\}}\to X$ be a non-degenerate simplex. Consider $f' = s_{n-1}d_n f: \Delta^{\{0, \ldots, n-1, n+1\}}\to X$, whose restriction to the first $n$ vertices agrees with that of $f$. These glue together to define $\bar f: \Delta^{\{0, \ldots, n\}}\cup_{\Delta^{\{0, \ldots, n-1\}}}\Delta^{\{0, \ldots, n-1, n+1\}}\to X$. Now I claim the following:

1. $\bar f$ extends to a simplex $g: \Delta^{\{0, \ldots, n+1\}}\to X$

2. The simplex $g$ is non-degenerate.

First, assume 1. and let us prove 2. Assume the contrary and suppose $g=s_i h$ for some $h: \Delta^n\to X$.

• If $i= n$, then this implies $f=d_{n+1}s_n h= h = d_n s_n h =f'$, but this is impossible since $f$ is non-degenerate and $f'$ is degenerate.
• If $i<n$, then $f=d_{n+1} g = d_{n+1}s_i h = s_i d_n h$, so again this contradicts to the assumption that $f$ is non-degenerate.

Therefore $g$ must be non-degenerate.

Now let us prove 1. It suffices to prove that the inclusion $i: \Delta^{\{0, \ldots, n\}}\cup_{\Delta^{\{0, \ldots, n-1\}}}\Delta^{\{0, \ldots, n-1, n+1\}}\to \Delta^{n+1}$ is an anodyne extension. For any $A\subset \{1, \ldots, n-1\}$ of cardinality $a$, let $\Lambda(A)$ be the horn $\Lambda^{a+2}_0 \hookrightarrow \Delta^{a+2} = \Delta^{\{0\}\cup A\cup \{n, n+1\}}\hookrightarrow \Delta^{n+1}$. Now observe that $i$ is the composition $i_{n-1}\circ\cdots\circ i_1 \circ i_{0}$, where $i_k$ is the "horn-filling inclusion" that fills $\{\Lambda(A)\mid |A|=k\}$.

Answer 2

Here I write an answer in the form I like (which I hope to be useful for others):

It's essentially the same as Naruki Masuda's answer above, but I don't like things like $\Delta^{\{0,1,\ldots,n-1,n+1\}}$, which I would write as the image of the map $d^n: \Delta^n\to\Delta^{n+1}$.