How can we see that the following $3$-manifolds are homeomorphic? I couldn't use the moves of Kirby calculus.
EDIT: An homeomorhism between $(-1/3)$-surgery $4_1$ and $(+1)$-surgery on $8_1$.
How can we see that the following $3$-manifolds are homeomorphic? I couldn't use the moves of Kirby calculus.
EDIT: An homeomorhism between $(-1/3)$-surgery $4_1$ and $(+1)$-surgery on $8_1$.
Here is a sequence of moves that gets you from left to right. (Pictures are done with Frenk Swenton's Kirby calculator.)
First, we convert from rational to integral surgery.
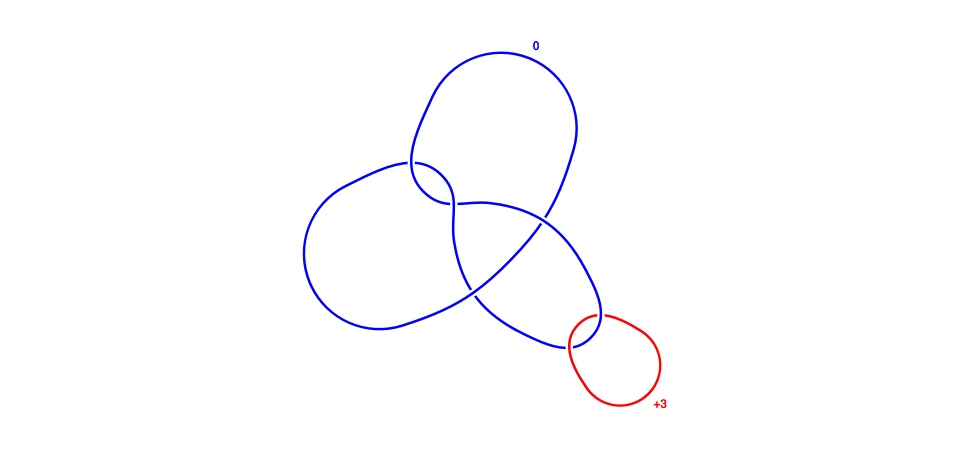 Then we blow up twice (this is standard for converting surgeries along the figure-8 knot to surgeries along the Borromean rings: we see the three rings here as the non-red sublink).
Then we blow up twice (this is standard for converting surgeries along the figure-8 knot to surgeries along the Borromean rings: we see the three rings here as the non-red sublink).
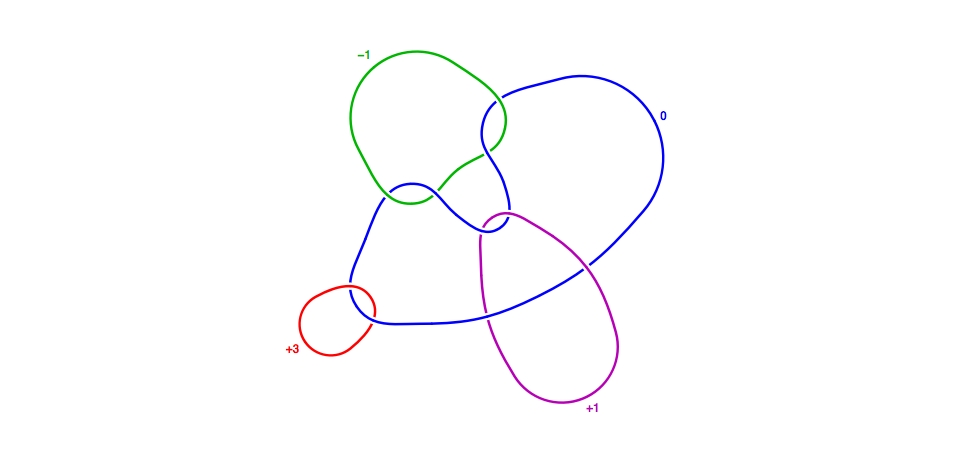 We want to convert the blue curve (which is a 0-framed unknot) into a 1-handle. We need to unlink things first, so we slide purple over red once...
We want to convert the blue curve (which is a 0-framed unknot) into a 1-handle. We need to unlink things first, so we slide purple over red once...
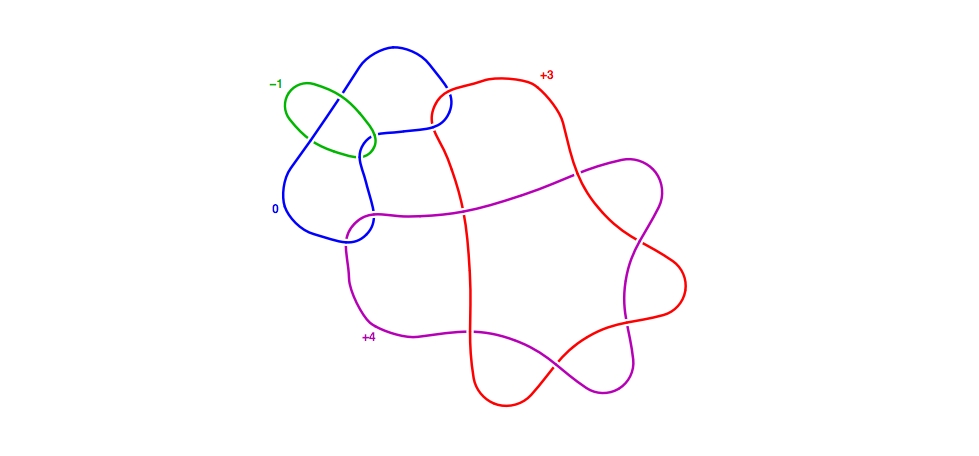 ... and then once more, along the band indicated here.
... and then once more, along the band indicated here.
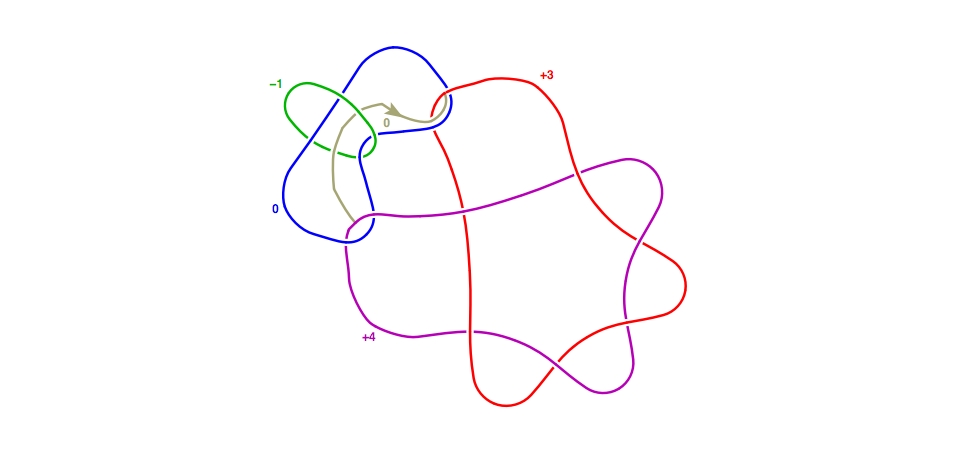 Now that we have a nicer (???) diagram ...
Now that we have a nicer (???) diagram ...
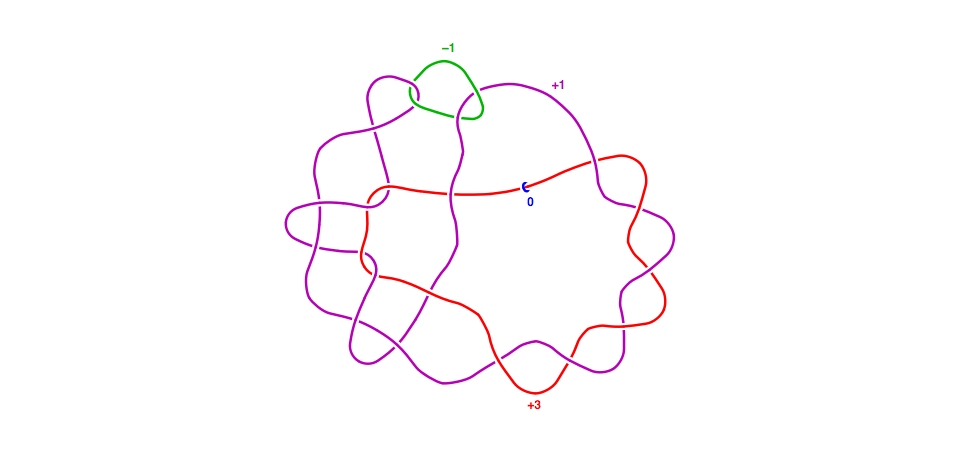 ... we can slam dunk the blue curve (or convert into 1-handle and cancel it with the +3-framed 2-handle, have it your way).
... we can slam dunk the blue curve (or convert into 1-handle and cancel it with the +3-framed 2-handle, have it your way).
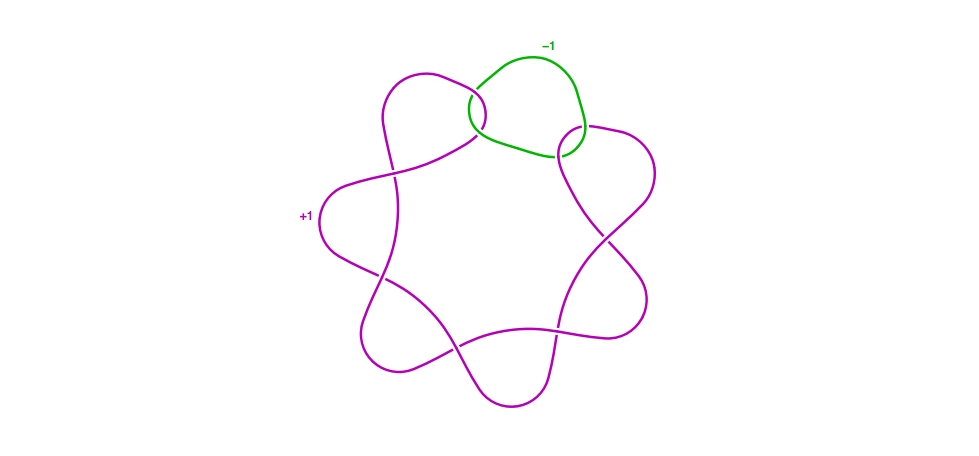 Now we perform an isotopy:
Now we perform an isotopy:
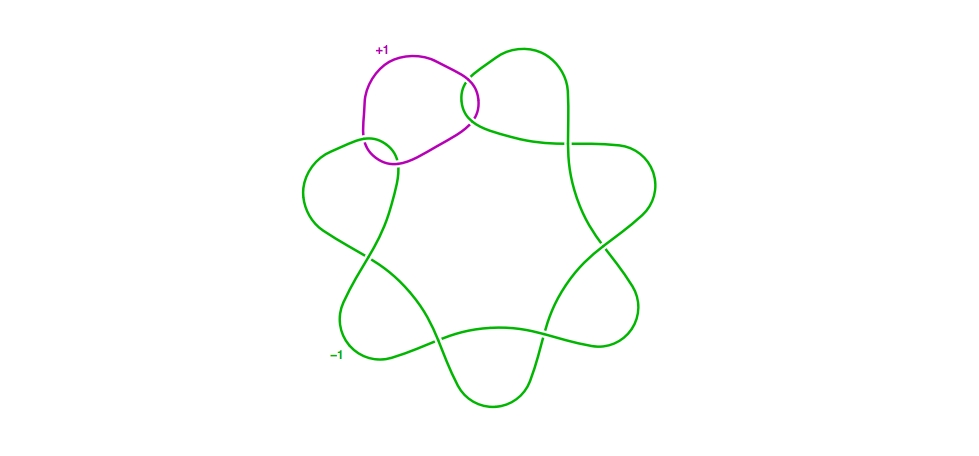 Blowing down the purple curve yields the surgery description on the right-hand side.
Blowing down the purple curve yields the surgery description on the right-hand side.
I'm adding this to supplement Marco's nice response. Let $8_1$ denote the original eight-crossing twist knot you drew, and let $m(8_1)$ denote its mirror. While we're at it, let "$\cong$" denote orientation-preserving homeomorphism.
If you blow down the $(+1)$-framed purple unknot in Marco's final figure, you obtain a surgery diagram for $(-1)$-surgery on $m(8_1)$, not on $8_1$. All told, Marco has shown $S^3_{-1/3}(4_1) \cong S^3_{-1}(m(8_1))$. Now, in general, for any knot $K \subset S^3$ and slope $r \in \mathbb{Q}$, there is an orientation-reversing homeomorphism from $S^3_r(K)$ to $S^3_{-r}(m(K))$. Thus we have a homeomorphism $S^3_{-1/3}(4_1)\cong S^3_{-1}(m(8_1)) \to S^3_{+1}(8_1)$ that is orientation-reversing.
On the other hand, we can show $S^3_{-1/3}(4_1) \not \cong S^3_{+1}(8_1)$ (as oriented 3-manifolds). First, take the mirror of all of Marco's diagrams. Note that, by considering framing curves, we must also flip the sign of every surgery. The new sequence of diagrams yields $S^3_{+1/3}(4_1) \cong S^3_{+1}(8_1)$ (where we've used $m(4_1)=4_1$ and $m(m(8_1))=8_1$). Now suppose $S^3_{-1/3}(4_1) \cong S^3_{+1}(8_1)$. Combining with the above, we obtain $S^3_{-1/3}(4_1) \cong S^3_{1/3}(4_1)$. However, this would be a "truly cosmetic surgery" on $4_1$, which we can rule out as below.
There might be easier arguments, but I'll use the Casson invariant $\lambda$. Let $\Delta_K(t)$ denote the Alexander polynomial, normalized so that $\Delta_K(t^{-1})=\Delta_K(t)$ and $\Delta_K(1)=1$. For example, $\Delta_{4_1}(t)=-t+3-t^{-1}$. Then, as a special case of Theorem 2.4 in "Surgery formulae for Casson's invariant and extensions to homology lens spaces" by Boyer and Lines, we have $$\lambda(S^3_{1/q}(K))=\frac{q}{2} \Delta''_K(1).$$ For $K=4_1$, we have $\Delta_{4_1}''(1)=2$, so $\lambda(S^3_{1/q}(4_1))=q$. It follows that $S^3_{-1/3}(4_1) \not \cong S^3_{1/3}(4_1)$ because these have different Casson invariants.
I checked that they are homeomorphic via SnapPy. Although not as satisfying as a proof, at least it is a useful check.
Ian's answer can be turned into a (uninformative) proof as follows:
In[17]: M.identify()
Out[17]: [m004(0,0), 4_1(0,0), K2_1(0,0), K4a1(0,0), otet02_00001(0,0)]
In[19]: N.identify()
Out[19]: [m074(0,0), 8_1(0,0), K5_2(0,0), K8a11(0,0)]
So those are hopefully the correct manifolds.
In[20]: M.dehn_fill((-1,3))
In[21]: N.dehn_fill((1,1))
We Dehn fill as indicated.
In[22]: Tm = M.filled_triangulation()
In[23]: Tn = N.filled_triangulation()
These are now "material" triangulations of the closed manifolds.
In[24]: Tm.isomorphisms_to(Tn)
Out[24]: []
This is very sad - the material triangulations are not combinatorially isomorphic. So we mess around a bit using .simplify and .randomize. When that does not work we use even more brute force:
In[32]: for i in range(100):
...: Tn.randomize()
...: if len(Tm.isomorphisms_to(Tn)) > 0: print("yay")
...:
yay
yay
yay
yay
So, they are homeomorphic. Note that the .isomorphisms_to method ignores orientation...
This is gives a second update to Sam Need's and Ian Agol's line of attack. However, Ian's computation uses some under the hood snappy functions (really SnapPea's kernel code isometry_closed.c), which does reports True if a homeomorphism between the manifolds is found.
However, one can `de-automate' the process if one wishes to get a few more details of the proof. Here is the method.
Take both cusped manifolds the 4_1 complement (aka the figure 8 complement) and 8_1 complement.
Fill one,
drill out short geodesics, and
check if that manifold obtained by drilling and filling is homeomorphic to the other and if so, record.
In[161]: M = Manifold('4_1')
In[162]: N = Manifold('8_1')
In[163]: N.dehn_fill((1,1),0)
In[164]: ND = N.drill(0)
In[165]: ND.volume(), ND.homology(), ND.identify()
Out[165]: (2.0298832128, Z, [m004(0,0), 4_1(0,0), K2_1(0,0), K4a1(0,0), otet02_00001(0,0)])
In[166]: ND.is_isometric_to(M,True)
Out[166]:
[0 -> 0
[-1 0] [ 3 1]
Does not extend to link,
0 -> 0
[1 0] [3 1]
Does not extend to link,
0 -> 0
[-1 0] [ 3 1]
Does not extend to link,
0 -> 0
[1 0] [3 1]
Does not extend to link,
0 -> 0
[ 1 0] [-3 -1]
Does not extend to link,
0 -> 0
[-1 0] [-3 -1]
Does not extend to link,
0 -> 0
[ 1 0] [-3 -1]
Does not extend to link,
0 -> 0
[-1 0] [-3 -1]
Does not extend to link]
What this is saying is that the filled and drilled manifold is homeomorphic to the the 4_1 complement but not framed the same way. The (1,0) curve for the filled and drilled manifold is the (-1,3) or (1,3) curve of the 4_1 complement. Meaning that the (1,1) filling of the 8_1 complement is homeomorphic to the (1,3) filling of the (standardly framed) 4_1 complement.
Snappy works a little better with cusped manifolds and ideal triangulations. It sidesteps some of Sam Need's brute force approaches for small complexity manifolds where computations with ideal triangulations (especially their canonizations) are robust.
Edit after the edit:
The original knots of the question are from Livingston's Knot Info. See the figure-eight knot $4_1$: It is different from $4_1$ in the Rolfsen's knot table.
According to Rolfsen's knot table, what Golla proved is $$S^3_{-1/3}(m(4_1)) = S^3_{-1}(8_1))$$ so that $$S^3_{-1/3}(4_1) = S^3_{-1}(8_1).$$
And this isomorphism seems to be known. See for example Tosun's paper pg. 9.
Edit after a fruitful discussion with Marco Golla:
My claim "$\Sigma(2,3,19) = S^3_{+1}(8_1)$" is based on Example 1.4 of Saveliev's book Invariants of Homology 3-Spheres.
According to Theorem 1.1. (3) of Brittenham and Wu, $S^3_{+1}(8_1)$ is hyperbolic and $S^3_{-1}(8_1)$ is Seifert fibered.
It seems that there is a sign/orientation inconsistency somewhere...
o____________________________________________________________________________
Golla and Hayden gave awesome responses to the question. Their arguments can be generalized in the following fashion.
Following their notations, recall that $K_1$ is the figure-eight $4_1$ knot and in general $K_n$ is the twist knot $(2n+2)_1$ in $S^3$.
They together proved $$S^3_{1/3}(K_1) \cong S^3_{+1}(K_3).$$
Note that the right-hand side is the Brieskorn sphere $\Sigma(2,3,19)$.
a- Handle diagrams of Golla is generalized to the next case as follows:
b- With the observations of Hayden, we have $$S^3_{1/4}(K_1) \cong S^3_{+1}(K_4).$$ There is a pattern in the Kirby calculus diagrams. Thus we may eventually prove that $$S^3_{1/n}(K_1) \cong S^3_{+1}(K_n).$$ Similarly, the right-hand side is the Brieskorn sphere $\Sigma(2,3,6n+1)$.
c- This part is about rational homology cobordism classes of $\Sigma(2,3,6n+1)$.
Definition: A knot $K$ in $S^3$ is called rationally slice if it bounds a smoothly properly embedded disk $D$ in a rational homology ball $X$.
Theorem(Kawauchi, (Kaw79) + (Kaw09)) Any hyperbolic amphichiral knot in $S^3$ is rationally slice. Consequently, $K_1$ is rationally slice in $S^3$.
Now we need an extra observation which is probably known to experts in low-dimensional topology and can be seen as the rational analogue of Gordon's theorem:
Lemma: For each $n$, $S^3_{1/n}(K_1)$ bounds a rational homology ball.
Proof: The figure-eight knot $K_1$ bounds a smooth disk $D$ in a rational homology ball $X$. The tubular neighborhood of $D$, $\nu(D)$, is $B^2 \times D$ in $X$.
Think $K_1$ and $D$ respectively as a belt sphere and co-core of $4$-dimensional $2$-handle $B^2 \times B^2$. So, we have $B^2 \times D = (X \setminus \nu(D))⋃ B^2 \times B^2.$
Now remove this $2$-handle and reattach it with a framing differing from the initial one by $n$ left-handed twists. Then the boundary $3$-manifold changes by $1/n$-surgery on $K_1$. Since we don't change the rational homology of $4$-manifold, we are done.
Therefore, we have a "theorem":
Theorem: For each $n$, Brieskorn spheres $\Sigma(2,3,6n+1)$ bounds a rational homology ball.
Remark: The cases $n=1$ and $n=3$ are known by Fintushel-Stern (FS84) and Akbulut-Larson (AL18). For the cases $n=2$ and $n=4$, they bound contractible $4$-manifolds due to classical results of Akbulut-Kirby (AK79) and Fickle (F84). Hence they a priori bound rational homology balls.