Abundancy index and non-solvable finite groups

Question

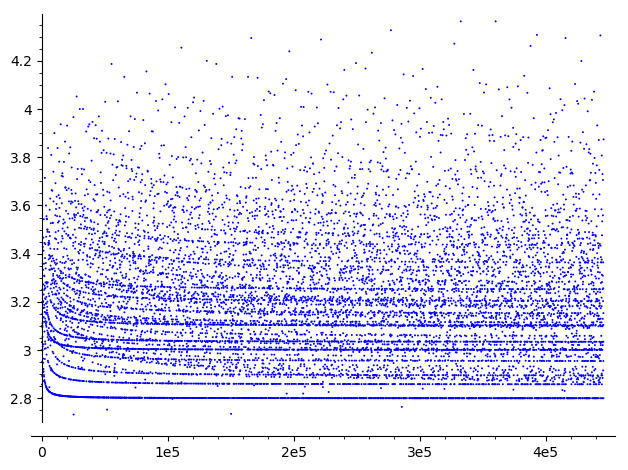
Let $\sigma$ be the sum-of-divisors function. A number $n$ is called abundant if $\sigma(n)>2n$. Note that the natural density of the abundant numbers is about $25 \%$. The abundancy index of $n$ is $\sigma(n)/n$. The following picture displays the abundancy index for the $10000$ first orders of non-solvable groups (see A056866).

Observe that for $G$ non-solvable with $|G| \le 446040$ then $|G|$ is abundant, with minimal abundancy index $\frac{910}{333} \simeq 2.73$.

Question 1: Are the non-solvable groups of abundant order?

Note that the number of integers $n \le 446040$ with $\sigma(n)/n \ge 910/333$ is exactly $19591$, so of density less than $5 \%$ with more than half of them being the order of a non-solvable group. Among those which are not the order of a non-solvable group, the maximal abundancy index is $512/143 \simeq 3.58$, realized by $n=270270$, whereas there are exactly $896$ numbers $n \le 446040$ with $\sigma(n)/n > 512/143$, which then are all the order of a non-solvable group.

Question 2: Is a number of abundancy index greater than $512/143$ the order of a non-solvable group?
Weaker version 1: Is there $\alpha >3$ such that a number of abundancy index greater than $\alpha$ must be the order of a non-solvable group?
Weaker version 2: Is there $\beta < 1$ such that a number $n$ of abundancy index greater than $\beta e^{\gamma} \log \log n$ must be the order of a non-solvable group?

Recall that $\limsup \frac{\sigma(n)}{n \log \log n} = e^{\gamma}$ with $\gamma$ the Euler-Mascheroni constant.

Finally, there are non-solvable finite groups $G$ with $|G| \gg 446040$ and abundancy index less than $\frac{910}{333}$. The non-abelian simple groups $G$ with $|G|=n \le 749186071932$ and $\sigma(|H|)/|H|>\sigma(n)/n$ for all non-abelian simple groups $H$ of order less than $n$ are exactly the 39 the simple groups $\mathrm{PSL}(2,p)$ with $p$ prime in {5, 37, 107, 157, 173, 277, 283, 317, 563, 653, 787, 907, 1237, 1283, 1307, 1523, 1867, 2083, 2693, 2803, 3413, 3643, 3677, 4253, 4363, 4723, 5443, 5717, 6197, 6547, 6653, 8563, 8573, 9067, 9187, 9403, 9643, 10733, 11443}. Let $n_p:=|\mathrm{PSL}(2,p)| = p(p^2-1)/2$.

It follows that for $G$ non-abelian simple with $|G| \le 749186071932$ then $|G|$ is abundant, with minimal abundancy index $$\sigma(n_{11443})/n_{11443} = 50966496/21821801 \simeq 2.33.$$

The following picture displays the adundancy index of $n_p$ for $p$ prime and $5 \le p \le 10^6$.

The minimal is $579859520520/248508481289 \simeq 2.3333 \simeq 7/3$, given by $p=997013$.

Question 3: Is it true that $\inf_{p \ge 5, \text{ prime}} \sigma(n_p)/n_p = 7/3$?

Question 4: Is the abundancy index of the order of a non-solvable group greater than $7/3$?

---

Fun fact: the smallest integer $n$ such that there exists two non-isomorphic simple groups of order $n$ is $20160$, whereas the biggest integer that is not the sum of two abundant numbers is $20161$ (see A048242). Any explanation?

Answer 1

I can answer Questions 1 and 4.

Make sure you look at S. Carnahan's answer. It deals with Questions 2 and 3.

Questions 1 and 4: If a finite group $G$ is not solvable then $|G|$ is divisible by $|G_0|$ for some finite simple group $|G_0|$. By the CFSG, either $12\bigm||G_0|$ or $G_0$ is a Suzuki group. If $12\bigm||G_0|$ then $$\frac{\sigma(|G|)}{|G|}\geq\frac{\sigma(|G_0|)}{|G_0|}>\frac{\sigma(12)}{12}=\frac{7}{3}.$$ If $G_0$ is a Suzuki group then $320\bigm||G_0|$ and $$\frac{\sigma(|G|)}{|G|}\geq\frac{\sigma(|G_0|)}{|G_0|}>\frac{\sigma(320)}{320}>\frac{7}{3}.$$ Thus, every non-solvable group has abundancy index larger than $\frac{7}{3}$.

Answer 2

As I mentioned in a comment, Question 2 (in its revised form) has a negative answer, because odd natural numbers have unbounded abundancy index, while the Odd Order Theorem implies all groups of odd order are solvable.

Weaker version 2 has a positive answer: If $\beta$ is sufficiently close to 1, then any $n > 1$ whose abundancy index is greater than $\beta e^\gamma \log \log n$ is a multiple of 60, so there is a group of order $n$ that is unsolvable.

As I mentioned in a different comment, Question 3 is true subject to well-known open conjectures, such as [Dickson's conjecture][1]. In particular, it suffices to show that there are infinitely many primes $p$ such that $p-1$ is 4 times a prime and $p+1$ is 6 times a prime. [1]: https://en.wikipedia.org/wiki/Dickson%27s_conjecture