Generalization of Tucker circle, Conway circle and van Lamoen circle

Question

Theorem 9.1 in this paper as follows is a generalization of Turker circle. Turker circles is a generalization of many circles as: Cosine Circle, circum circle, First Lemoine Circle, Gallatly Circle, Kenmotu Circle, Taylor Circle, Apollonius Circle.

The Conway circles and Floor van Lamoen circle are also special case of Turker circle.

I also given some anothert nice special cases of Theorem 9.1, example Theorem 9.2, Theorem 9.3, Theorem 9.6, Theorem 9.7 of that paper.

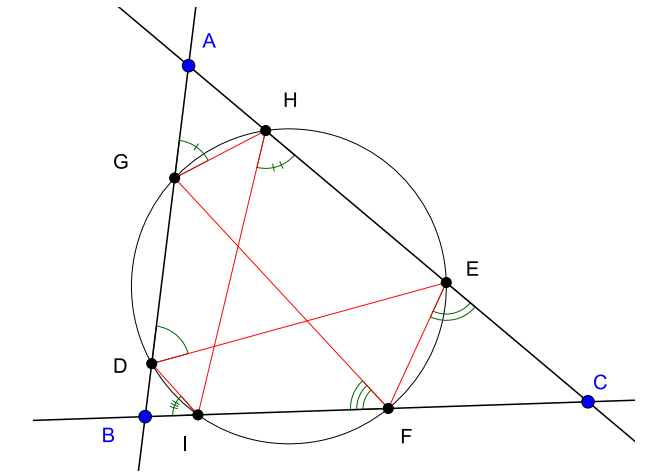
Theorem 9.1. Let $ABC$ be a triangle, let points $D$, $G$ be chosen on side $AB$, points $I$, $F$ be chosen on side $BC$, points $E$, $H$ be chosen on side $CA$, let $k$, $l$ are real number such that:

$\begin{cases} \angle EDA =kA+lB+(1-k-l)C\\ \angle FEC =(1-l)A+(k+l)B-kC\\ \angle GFB = (1-k-l)A+kB+lC\\ \angle HGA =-kA+(1-l)B+(k+l)C \\ \angle IHC=lA+(1-k-l)B+kC \end{cases}$

Then six points $D, E, F, G, H, I$ lie on a circle and $ \angle DIB = (k+l)A-kB+(1-l)C$

Coverse of Theorem 9.1: Let $ABC$ be a triangle, let points $D$, $G$ be chosen on side $AB$, points $I$, $F$ be chosen on side $BC$, points $E$, $H$ be chosen on side $CA$ and six points $D, E, F, G, H, I$ lie on a circle. Then exist two real numbers $k$, $l$ such that:

$\begin{cases} \angle EDA =kA+lB+(1-k-l)C\\ \angle FEC =(1-l)A+(k+l)B-kC\\ \angle GFB = (1-k-l)A+kB+lC\\ \angle HGA =-kA+(1-l)B+(k+l)C \\ \angle IHC=lA+(1-k-l)B+kC \\ \angle DIB = (k+l)A-kB+(1-l)C \end{cases}$

My question: Is the converse of Theorem 9.1 true?

Answer 1

For the converse, take first one triangle $GIE$ then define the line sides of $ABC$ by the angles formed on the vertices. In notation $(\vec{IE},\vec{IG})=N, (\vec{GI},\vec{GE})=L$ and $(\vec{EG},\vec{EI})=M$.

Take line $(GG')$ such that $(\vec{GE},\vec{GG'})=s$, line $(II')$ with $(\vec{IG},\vec{II'})=t$ and line $(EE')$ with $(\vec{EI},\vec{EE'})=u$.

Finally $(GG')\cap (EE')=A$, $(GG')\cap (II')=B$, and $(II')\cap (EE')=C$. Also say $(\vec{AB},\vec{AC})=\hat{A}$, $(\vec{CA},\vec{CB})=\hat{C}$ and $(\vec{BC},\vec{BA})=\hat{B}$.

This is just the given figure but with directed angles in a more general way. Assuming we have the points on the side segments of $ABC$ (there is a version where the points can be on the lines of the sides)

So $\begin{cases}\hat{A}=M+u-s\\\hat{B}=L+s-t\\\hat{C}=N+t-u\end{cases}$ Assuming the cocyclicity of the points (and from the given figure) we want to prove that there exist $k,l$ so that $\begin{cases}s-u=k\hat{B}+l\hat{C}-(k+l)\hat{A}\\u-t=k\hat{A}+l\hat{B}-(k+l)\hat{C}\end{cases}$ for any $s,u,t$ and the given $\hat{A},\hat{B},\hat{C}$.

For that the linear system should be invertible; a direct calculation shows that this is the case when $\hat{A}^2+\hat{B}^2+\hat{C}^2\neq \hat{A}\hat{C}+\hat{B}\hat{C}+\hat{A}\hat{B}$

The rest is angle chasing of the given arcs and lines, for example in the figure $\begin{cases}\widehat{FEC}=N+M-C\\\widehat{HGA}=L+M-A\\\widehat{DIB}=L+N-B\end{cases}$ which are the given values.