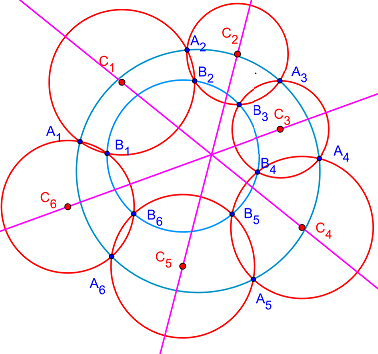
In 2013, I found a new problem as follows: Let six points $A_1$, $A_2$, ...$A_6$ lie on a circle $(O_1)$, and the six points $B_1$, $B_2$,...,$B_6$ lie on another circle $(O_2)$. If the quadruples $A_i$, $A_{i+1}$, $B_{i+1}$, $B_i$ lie on a circles with centers $C_i$ for $i=1,2,...,5$ then $A_6$, $A_1$, $B_1$, $B_6$ lie on a circle (namely center of the new circle is $C_6$) and $C_1C_4$, $C_2C_5$, $C_3C_6$ are concurrent.
The proof [3][4] show that the hexagon $C_1C_2C_3C_4C_5C_6$ circumscribed around a conic section with two Focus are $O_1$, $O_2$
The Theorem was re-discovered by Szilasis in 2017 (see [5])
Converse of theorem: Let a hexagon $C_1C_2C_3C_4C_5C_6$ which $C_1C_4, C_2C_5, C_3C_6$ are concurrent then exist many configuration of eight circles as figuration above.
If the converse theorem is true, so the theorem is equivalent with an old theorem due to Brianchon
Special case: Let a hexagon $C_1C_2C_3C_4C_5C_6$ circumscribed a conic with two focus $O_1$, $O_2$. Draw six circles $(C_1)$, $(C_2)$,....,$(C_6)$ with centers $C_1$, $C_2$,...$C_6$ and radii $C_1O_1$, $C_2O_1$, ...., $C_6O_1$ respectively. Let circles $(C_i)$ meets circle $(C_{i+1})$ again at $A_i$ then six points $A_1, A_2,..., A_6$ lie on a circle, namely circle $(A)$. Similarly: Draw six circles $(C_1)$,$(C_2)$,....,$(C_6)$ with centers $C_1$, $C_2$,...$C_6$ and radii $C_1O_2$, $C_2O_2$, ...., $C_6O_2$ respectively. Let circles $(C_i)$ meets circle $(C_{i+1})$ again at $B_i$ then six points $B_1, B_2,..., B_6$ lie on a circle, namely the circle $(B)$.
Question 1: Is the converse of theorem true?
Question 2: How can one prove the radius of circle $(A)$ is equal to the radius of circle $(B)$?
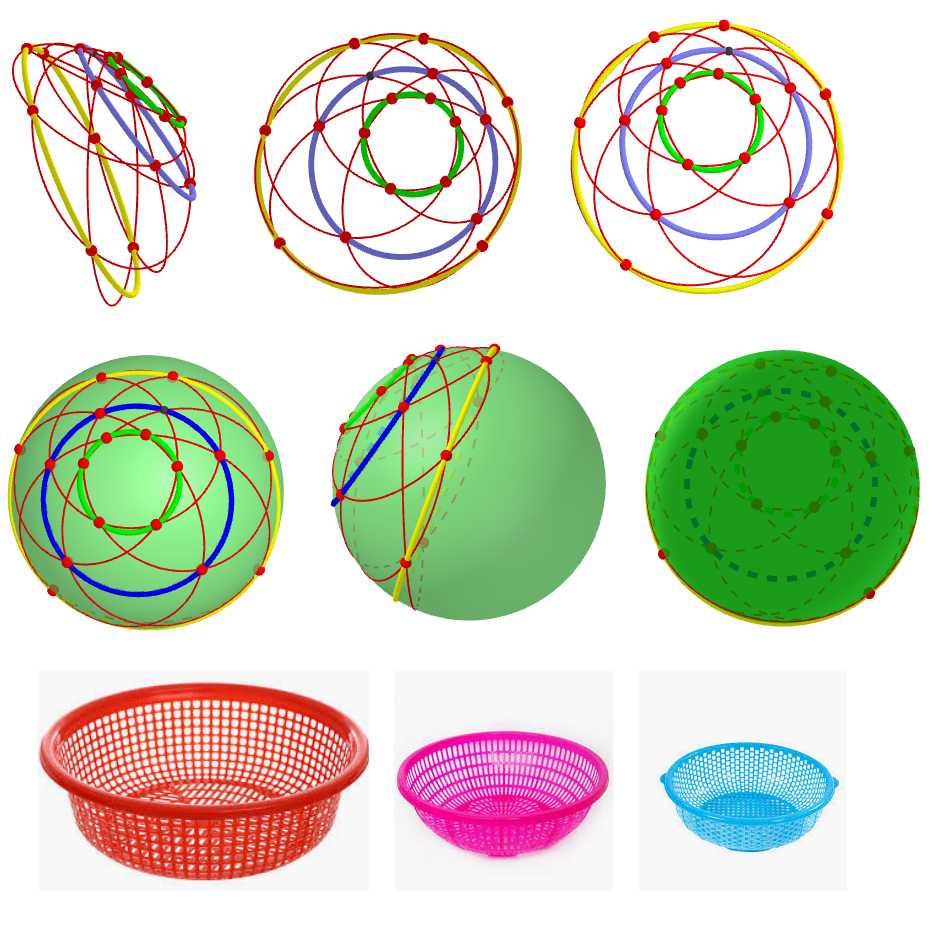
The dual of theorem on the ball creat a beautifull basket (see theorem dual in [8])
Some relation problem
Dao's theorem on six circumcenters
Some Problems On Apollonian Gasket
Can generalization of a generalization Pascal theorem, Pappus theorem to Higher Dimensions?
A chain of six circles associated with six points on a circle (in Mobius plane)
$N$-$th$ closed chain of six circles
Reference:
1 - Dao, O.T.: Problem 3845, Crux Mathematicorum, 39, Issue May 2013
2-https://www.geogebra.org/material/show/id/Zk3F5y5X
3 - J. Chris Fisher, Problem 3945, Crux Mathematicorum, Volume 40, Issue May, 2014
[4]-Michel Bataille, Solution to Problem 3945, Crux Mathematicorum, Volume 41, Issue May, 2015
[5]-Gábor Gévay, A remarkable theorem on eight circles, Forum Geométrico rum, Volume 18 (2018), 401--408
[6]-Ákos G.Horváth, A note on the centers of a closed chain of circles