2
$\begingroup$

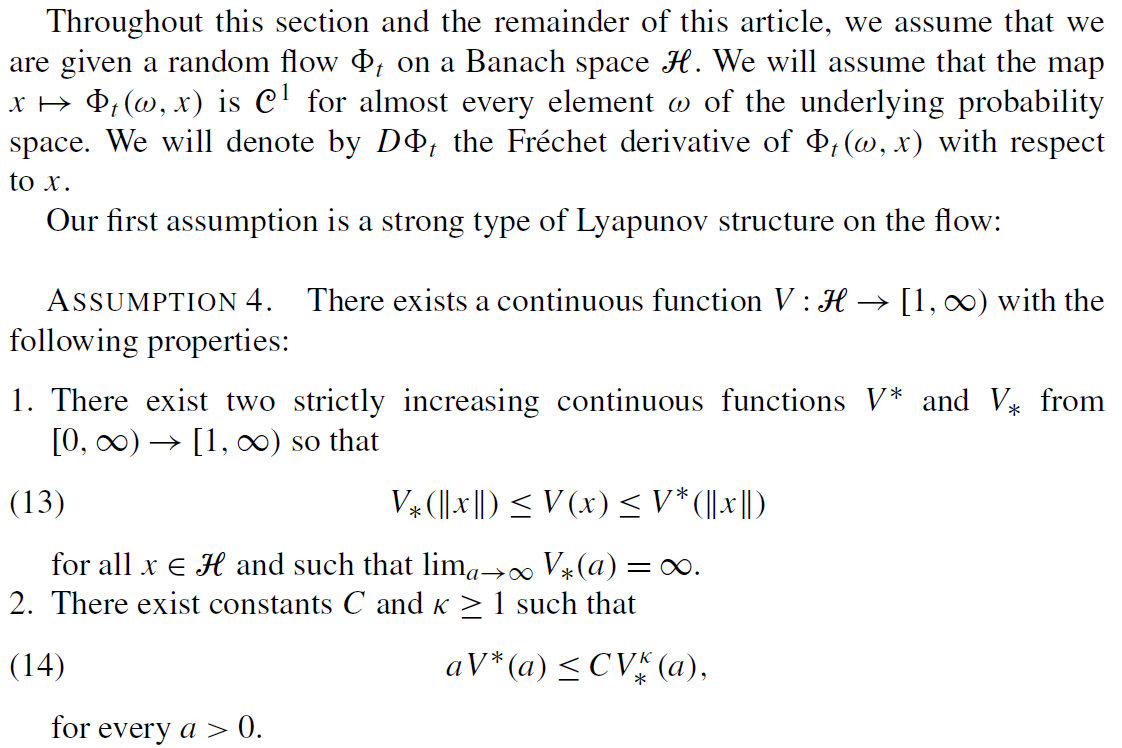
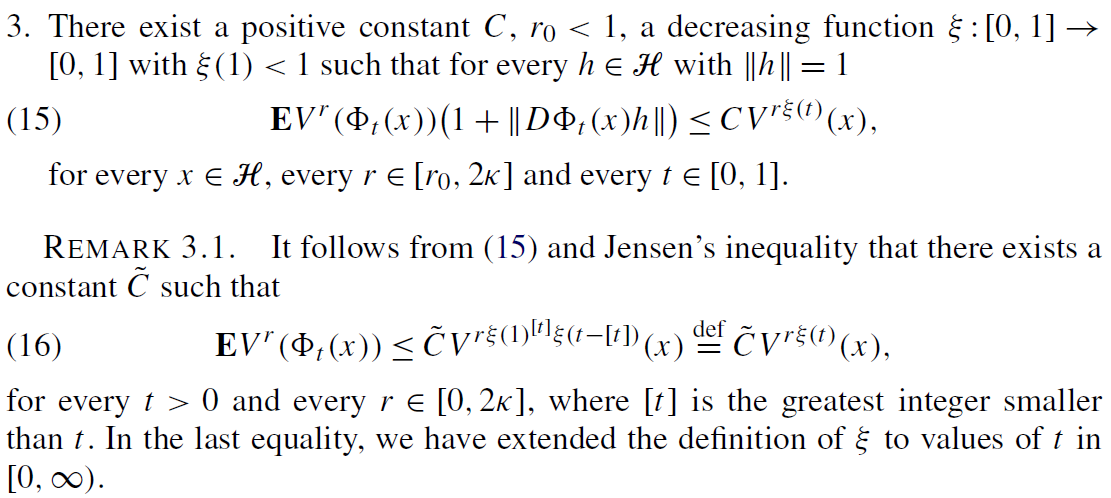
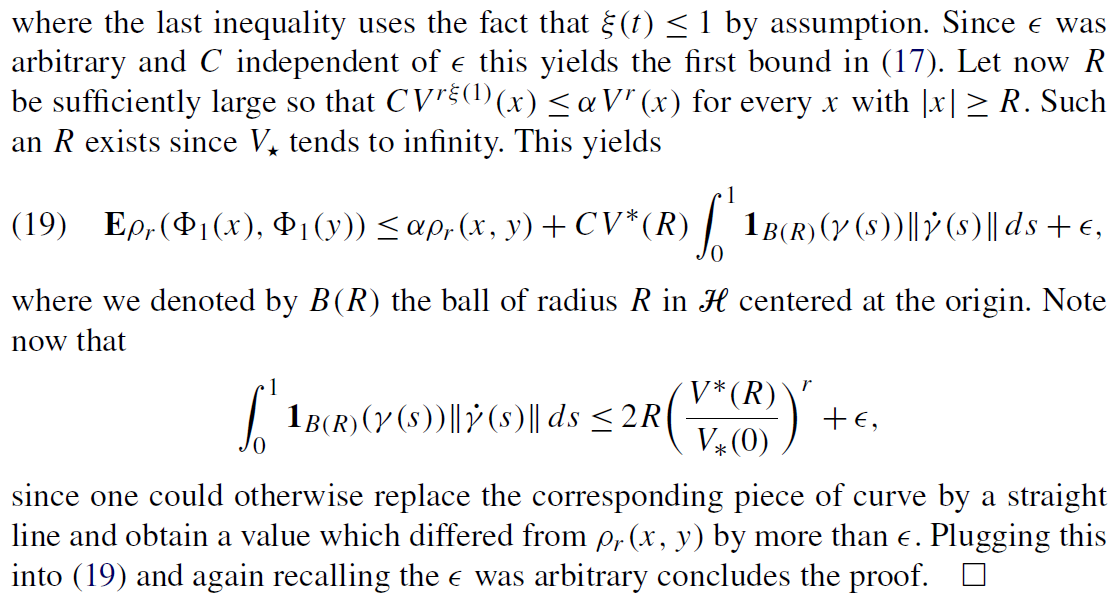
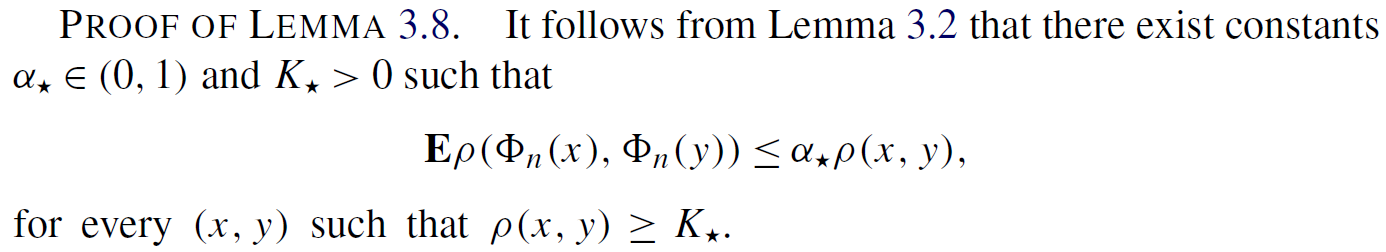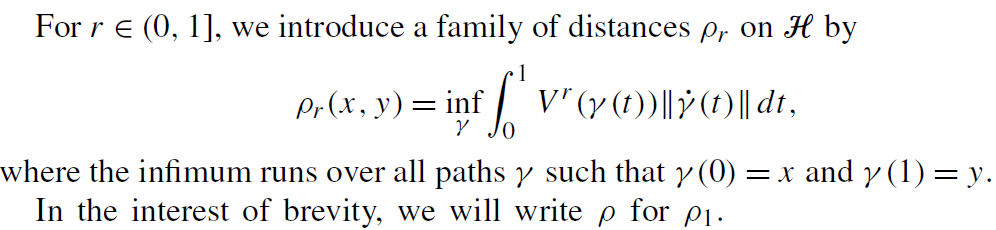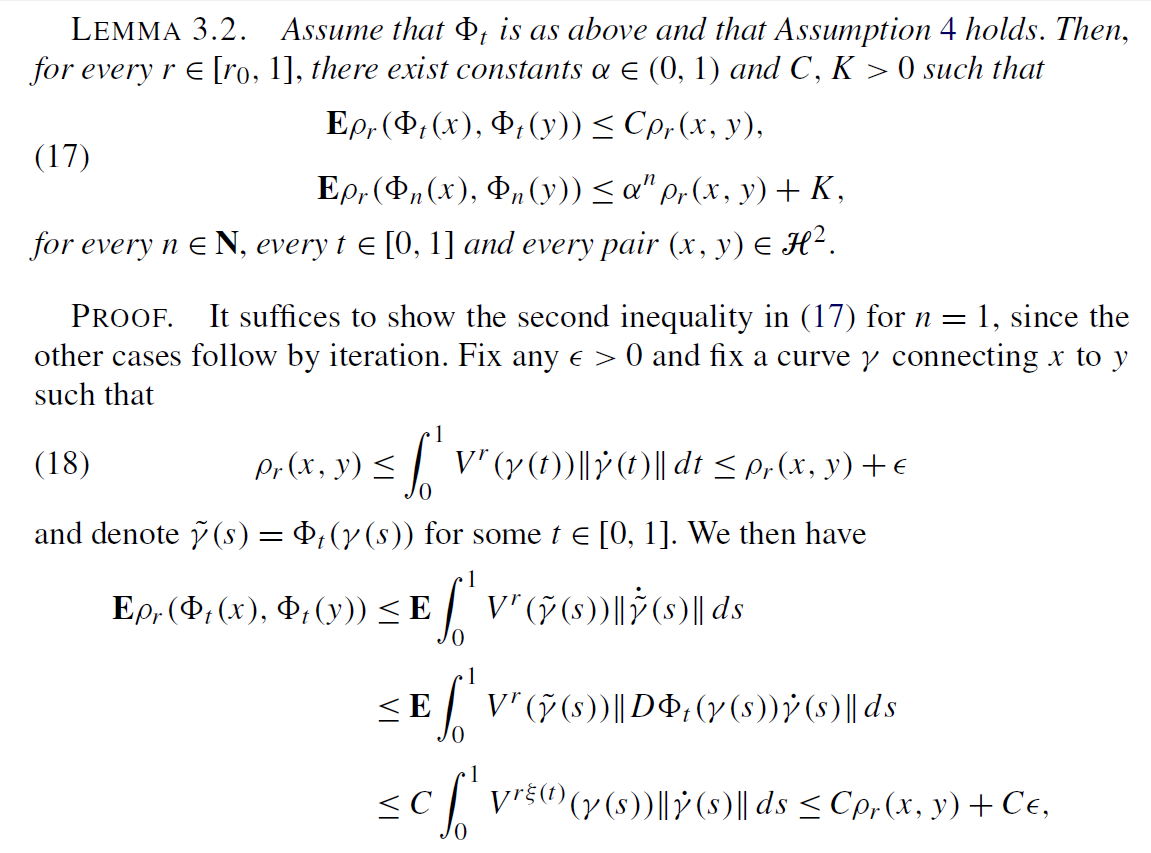I'm trying to understand the following conclusion from this paper (see below for the relevant paragraphs):

enter image description here

I'm not sure whether they really mean that it follows from the statements of Lemma 3.2 (since I absolutely don't see how this conclusion is possible) or from its proof (which I could imagine).

Taking a closer look to the proof of Lemma 3.2-ii, we see that the result actually is that for all $r\in[r_0,1)$ and for all $\alpha\in(0,1)$, there is a $K\ge0$ such that $$\operatorname E\left[\rho_r(X^x_1,X^y_1)\right]\le\alpha\rho_r(x,y)+K\;\;\;\text{for all }x,y\in E\tag1.$$ And, as always, this extends to bounds for $\operatorname E\left[\rho_r(X^x_n,X^y_n)\right]$ by the semigroup property.

In the proof the additive $K$ corresponds to a bound on an integral restricted to an open ball. Maybe the assumption $\rho(x,y)\ge K_\ast$ can be used to show this integral is $0$?

enter image description here enter image description here enter image description here enter image description here enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

No, you just have to take $K_*$ such that $K \leq (\alpha_*-\alpha)\rho(x,y)$, and so for example $K_* = \frac{K}{\alpha_*-\alpha}$.

If I am not missing something, a good way of doing the proof could be:

Since $\alpha<1$, there exists $\alpha_*\in (\alpha,1) \subset (0,1)$. Now take $K_* = \frac{K}{\alpha_*-\alpha}$ and $\rho(x,y) ≥ K_*$. Then $$ \begin{align*} \alpha\,\rho(x,y) + K &= \alpha\,\rho(x,y) + (\alpha_*-\alpha)\,K_* \\ &≤ \alpha\,\rho(x,y) + (\alpha_*-\alpha)\,\rho(x,y) = \alpha_*\,\rho(x,y) \end{align*} $$

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you for your answer. A few remarks: It should be $K_\ast:=\frac K{\alpha_\ast-\alpha}$, since otherwise $K_\ast$ is negative. And the first inequality in your displaced equation is an equality. $\endgroup$
    – 0xbadf00d
    Commented Jun 13, 2020 at 18:37
  • $\begingroup$ Yes, corrected ;) $\endgroup$
    – LL 3.14
    Commented Jun 13, 2020 at 18:51
  • $\begingroup$ They proceed in the proof of Lemma 3.8 by showing a rather simple inequality: i.sstatic.net/hJRkW.png. It seems like they are claiming that $$1-\alpha_\ast+\alpha_\ast d(x,y)\le\frac{1+\alpha_\ast\beta K_\ast}{1+\beta K_\ast}d(x,y),$$ but I'm not able to see how they obtain this. They say that it follows from $d(x,y)\ge1+\beta K_\ast$ and so I've tried to add $d(x,y)-(1+\beta K_\ast)\ge0$ to the right-hand side. However, I wasn't able to arrange the terms so that they match the right-hand side. Do you've got an idea? $\endgroup$
    – 0xbadf00d
    Commented Jun 14, 2020 at 6:15

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .