Consider a triangulated polygon in the 2-dimensional plane, where each vertex is labelled green, blue, or orange. Sperner's Lemma asserts that a fully-colored triangle exists in the triangulation, if the boundary vertices a suitably labelled.
Looking at the close combinatorial link between Sperner's Lemma and Tucker's Lemma (Direct combinatorial link between Sperner's and Tucker's Lemma?), I am interested in structural results about the boundary labelling for Sperner's Lemma.
To specify my question, I am using an example of a Sperner labelling of the boundary vertices (not showing the triangulation and inside vertices).
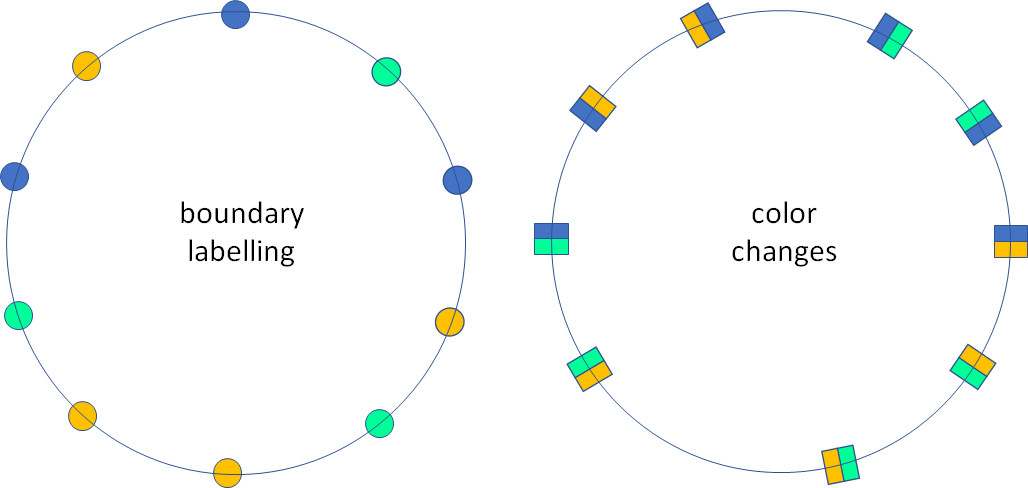
It is not obvious at first sight that this is a valid Sperner labelling. But the number of color-changes (e.g. from blue to green) is uneven, so the conditions of Sperner's Lemma are met. This can best be seen in the diagram on the right which just shows the color changes.
How is this complex labelling example related to the minimum base case? Base case means when the boundary has the minimum labelling with just three color-changes:
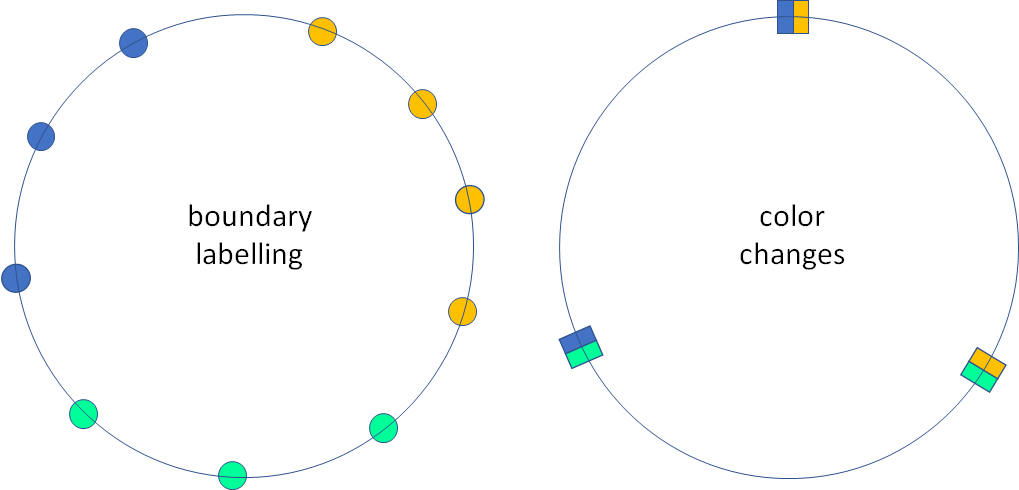
My working hypothesis is that every valid Sperner labelling of the boundary follows from the minimum base case in a unique way: by adding layers of even-numbered color changes. This is of key interest when studying the link with Tucker's Lemma which requires an antipodally symmetric boundary labelling.
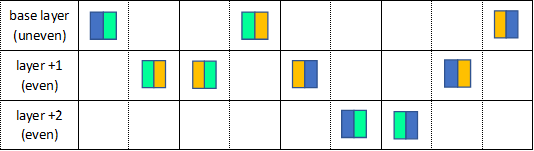
For clarification of this idea, the example from above decomposes into three layers. Starting from the (uneven) base layer and adding two (even) layers of color-changes:
I have searched for results in combinatorics and topology in that direction, but could not find anything. Would someone by able to point me to a good reference?
