Location of bulk and edges for Gaussian random matrices

Question

I have some trouble to understand the difference between the "bulk" and the "edges" of the spectral density of random matrices (for instance in this question).

From my understanding, all properties of random matrices eigenvalues are actually only valid in the bulk (correlations for instance). But where does the separation begin ?

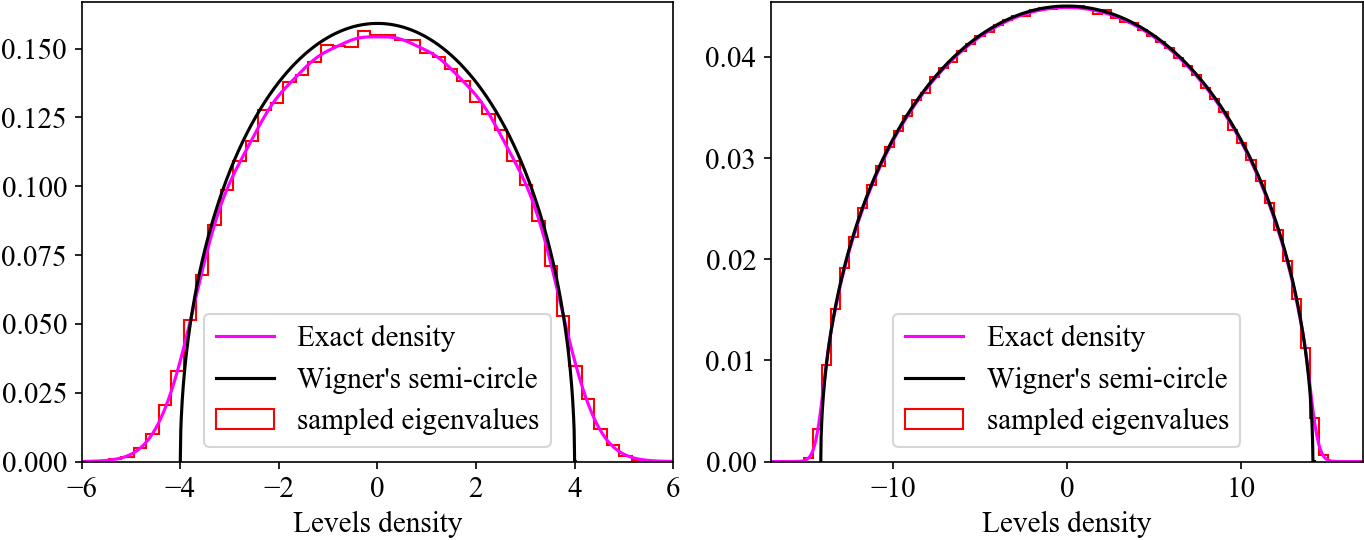
Let's take the example of Gaussian matrices. For instance, for 8x8 or 100x100 random matrices from the GOE, the spectral density looks like:

I initially thought "the bulk" designated for these matrices the inner part of the Wigner semi-circle, while the edges were the outer parts. Is such a crude guess a valid approximation (for practical applications for instance) ?

Answer 1

It is important to distinguish results that apply only in the limit of a large matrix size $N$, from results that apply for small $N$ as well. The theory of random matrices addresses both large-$N$ properties as well as small-$N$ properties, and there are physical applications in both regimes.

I understand from your question that your interest is in large-$N$ properties of the GOE. Then "bulk" versus edge refers to the support $(-W,W)$ of the Wigner semicircle, rescaled such that $W={\cal O}(1)$. The transition from bulk to edge region is at a separation of order $N^{-2/3}$ from $\pm W$. The deviation of the eigenvalue density from the Wigner semicircle in the edge region is described by the Tracy-Widom law.

But do keep in mind that the theory of random matrices is not restricted to the large-$N$ regime. For example, chaotic scattering from a billiard with an $N\times N$ transmission matrix is described by the circular ensembles for any $N$, even as small as $N=1,2,3,\ldots$. In that case there is no notion of bulk versus edge, but there are universal properties that can be measured in experiments, such as the $T^{-1/2}(1-T)^{-1/2}$ distribution of the transmission probability $T\in(0,1)$ for $N=1$.