Let $K/ \mathbb Q $ be a finite Galois extension and let $X$ be a proper non-empty subset of the Galois group $G=Gal(K/ \mathbb Q)$ that is closed under conjugation. Consider a set of integer primes $P$ such that for all sufficiently large primes $p$, the following equivalence holds $$p \in P \iff \text{ the conjugacy class of the Frobenius element }\sigma_p \text{ is contained in }X$$
Now let $E$ be a multiplicative set of natural numbers (that is, for all coprime $m, n \in \mathbb N$, we have the equivalence $mn \in E \iff m \in E$ or $n \in E$) such that the set of prime numbers in $E$ is exactly the set $P$ above and let $E' := \mathbb N \setminus E$ denote the complement of $E$. Consider the indicator sequence $(a_n)_{n \geq 1}$ of $E'$ (so that $a_n := 1 \iff n \in E'$ and $a_n=0$ otherwise) and let $F(s) := \sum_{n \geq 1} a_n n^{-s}$ be the Dirichlet Series corresponding to the sequence $(a_n)_{n \geq 1}$.
I want to show that the function $F$ analytically continues to a region of the form given in the image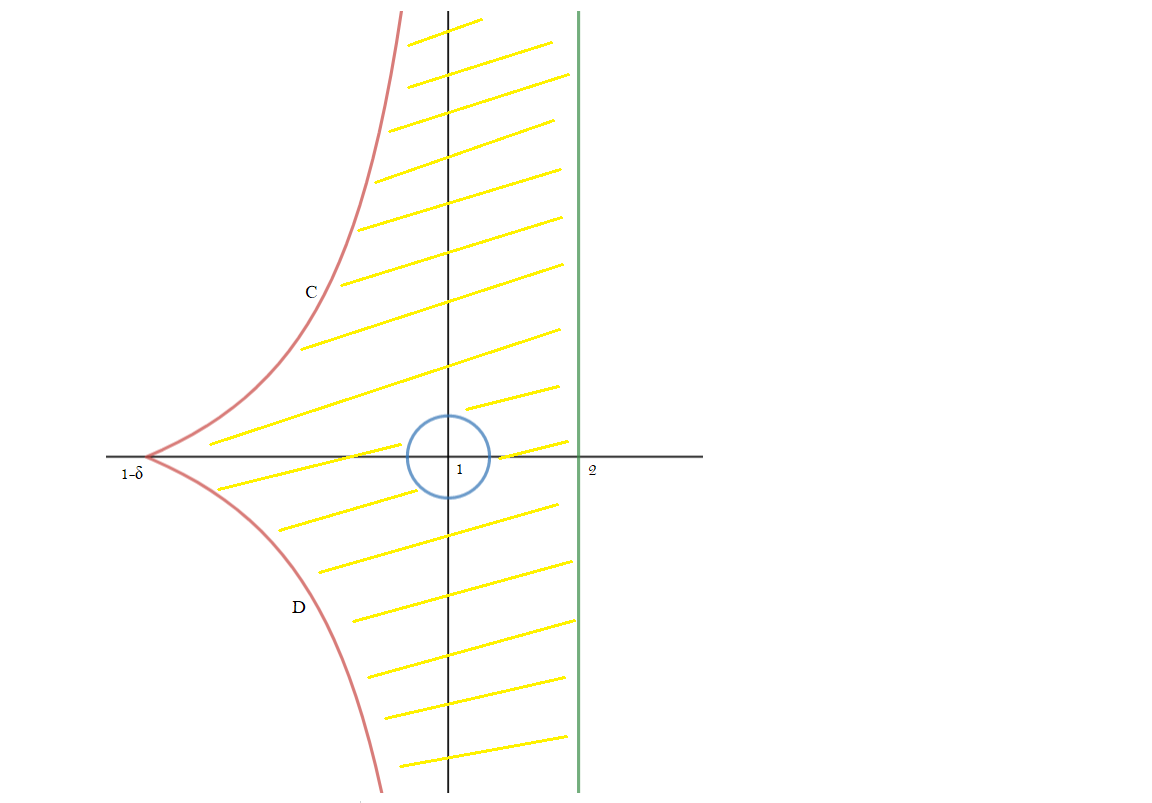 
where $\delta>0$ is fixed, the circle around the point $1$ is of radius $\epsilon < \delta$ and the infinite branches $C$ and $D$ are defined by
$$\Re(s) = 1 - \frac{a}{(\log (2+|\Im(s)|))^A}$$
(where $a$ and $A$ are fixed positive numbers, note that the interior of the circle has been excluded from the aforementioned region) such that in this region we have
$$F(s) = O((\log |\Im(s)|)^A) \text{ as } |\Im(s)| \rightarrow \infty$$
The only results of this kind I am somewhat familiar with are those on the analytic continuation of the usual Riemann Zeta Function (which I read in Apostol's ``Introduction to Analytic Number Theory"). Although I have obtained some other immediate observations (for instance: the natural and Dirichlet density of $P$ must both be $|X|/|G| \in (0,1)$ by the Chebotarev Density Theorem and that the sequence $(a_n)$ should be multiplicative hence we can get something akin to an `Euler-Product' representation of the Dirichlet Series $F(s)$), I have no general idea on how to get started on this problem and I would really a proof or a reference containing a complete (and preferably not too inaccessible) proof of the same. Thank you.
P.S.: It says here (Continuation up to zero of a Dirichlet series with bounded coefficients) that a Dirichlet series with bounded coefficients need not be meromorphically continuable to the right of zero, but I haven't found any positive results on M.O. in this direction.
Edit (19-05-2020): I found the following result (although I don't know how to show this one either), which I think might be relevant:
If $f_P(s) = \sum_{p \not\in P} p^{-s}$, then $f_P$ extends into a holomorphic function to the right of the curve $C$ and $D$ (in the image) except for the real axis from $1-\delta$ to $1$, that is into a region of the form
$$R:= \left\{ s \in \mathbb{C} \Bigg| \Re(s) \geq 1-\frac{a}{(\log T)^A}, \Im(s) \neq 0 \right\} \cup \Big((1, \infty) \times 0 \Big)$$
where it also satisfies the bound $f_P(s) = O(\log \log (2+|\Im(s)|))\text{ as }|\Im(s)| \rightarrow \infty$.
I could also show that the function $h(s):= \log F(s) - f_P(s)$ is holomorphic for $\Re(s) \geq 1$. But I am still not sure how I can complete the proof from here. I would appreciate a proof even if it assumes these the two results.
