Are there any nice models of surfaces with non-constant negative curvature, analogous to the Poincare disk for constant negative curvature. I have found lots of general results and theory but no nice clean models.
-
5$\begingroup$ Until you specify what you mean by model, and how exactly you give the curvature function, this question is impossible to answer. $\endgroup$– Andy SandersCommented May 4, 2020 at 16:12
-
3$\begingroup$ To clarify, something like a subset of the plane with a riemanian metric where the geodesics and horocycles are easy to describe. $\endgroup$– ericfCommented May 4, 2020 at 20:00
-
2$\begingroup$ This previous question has a nice discussion of related issues and ideas: mathoverflow.net/questions/37651/… $\endgroup$– Andy SandersCommented May 4, 2020 at 20:15
-
$\begingroup$ The question remains imprecise. Should it be interpreted as: "what is a classification of simply connected complete negatively curved surfaces"? or maybe those with a cocompact [cofinite volume] isometry group, if one is interested in compact [finite volume] surfaces. I understand that the answer is expected to be given in terms of model, i.e., say, explicit Riemannian structures, say, on explicit simply connected open subsets of the plane. $\endgroup$– YCorCommented May 12, 2020 at 10:21
4 Answers
If you just want examples for which it's not hard to figure out how the geodesics behave, here's a class of examples with negative and non-constant curvauture in the plane where the geodesics are relatively easy to understand:
Let $a$ and $b$ be smooth functions on $\mathbb{R}$ such that $a(x)+b(y)>0$ for all $x,y\in\mathbb{R}$ and consider the metric $$ g = \bigl(a(x) + b(y)\bigr)(\mathrm{d}x^2 + \mathrm{d}y^2) $$ on $\mathbb{R}^2$. The curvature of this metric is $$ K = \frac{a'(x)^2+b'(y)^2-\bigl(a''(x)+b''(y)\bigr)\bigl(a(x)+b(y)\bigr)} {2\,\bigl(a(x)+b(y)\bigr)^3} $$ It's easy to choose $a$ and $b$ so that $K<0$. For example, $a(x) = x^2+1$ and $b(y) = y^2+1$ gives a complete metric on $\mathbb{R}^2$ that has non-constant negative curvature $K = -4/(x^2{+}y^2{+}2)^{3}<0$.
Note that, taking $a$ (respectively, $b$) to be a constant gives a metric $g$ that has a Killing vector field, namely $\partial/\partial x$ (respectively, $\partial/\partial y$), but, for generic choices of $a$ and $b$, the metric $g$ will have no Killing vector field.
As for geodesics, the good thing about these metrics (called Liouville metrics in the literature) is that their geodesic flows are integrable: Any unit speed geodesic $(x(t),y(t))$ satisfies $$ \bigl(a(x)+b(y)\bigr)\bigl(\dot x^2+\dot y^2\bigr) = 1 \quad\text{and}\quad \bigl(a(x)+b(y)\bigr)\bigl(b(y)\,\dot x^2- a(x)\,\dot y^2\bigr) = c $$ for some constant $c$. (Note that, when either $a$ or $b$ is constant, this second 'first integral' of the geodesic equations specializes to the well-known 'Clairaut integral' for surfaces of revolution.)
In particular, $$ \bigl(b(y)-c)\bigr)\,\dot x^2 - \bigl(a(x)+c)\bigr)\,\dot y^2 = 0, $$ and, assuming that you are in a region when $a(x){+}c$ and $b(y){-}c$ are both positive, $$ \frac{\mathrm{d}x}{\sqrt{a(x)+c}} \pm \frac{\mathrm{d}y}{\sqrt{b(y)-c}}=0, $$ which gives two foliations of this region by geodesics, which can be found by quadrature.
In any case, you will have good qualitative control over these geodesics and can draw some nice pictures.
Added remark (12 May 2020): As an example of what one can do with this more explicit information, you might be interested in this answer of mine to an old question about Riemannian surfaces for which one can compute an explicit distance function.
Minimal surfaces without umbilics. They are nice and have negative Gaussian curvature. The catenoid is a particular example.
-
$\begingroup$ Hey Sebastian, you're certainly right that generally speaking the Enneper-Weierstrass parameterization is a nice way to generate examples of negatively curved surfaces. But, they do tend to be immersed and therefore extrinsic in nature, so they are quite different than the kind of model that the Poincare disk is. Still, this is a nice class of examples. $\endgroup$ Commented May 4, 2020 at 19:54
-
3$\begingroup$ Hi Andy, I see your point. On the other hand we do not have an extrinsic model for the hyperbolic ball in 3-space as well, and the explicit form for the metric of a minimal surface is given on the domain of the conformal parametrisation. Of course, one can ask which complete embedded examples without umbilics do exist. But even with the further constraint of finite total curvature we have at least one (class of) example(s). $\endgroup$ Commented May 5, 2020 at 7:19
You don't even need to settle for a model. You can make such a surface. Take any positive strictly convex smooth function ($f(x) > 0$ and $f''(x) > 0$) and rotate it around the x axis.
The single-sheet hyperboloid, $x^2 + 1 = y^2 + z^2$, has some nice geodesics, but also some messier ones: https://math.stackexchange.com/questions/1601158/how-can-we-find-geodesics-on-a-one-sheet-hyperboloid
You may be able to calculate the geodesics with methods given here: Geodesics on a hyperbolic paraboloid (which is yet another negative curvature surface)
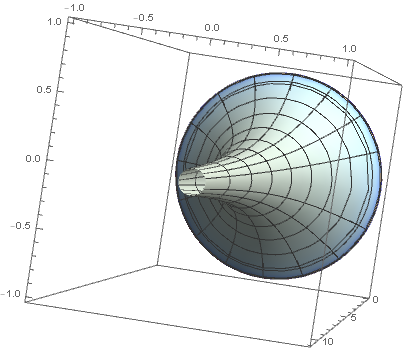
You can play around with this in Mathematica following the suggestions here on Mathematica StackExchange. E.g.
gccolor[{Cos[u] Sech[v], Sin[u] Sech[v], v + v^2 - Tanh[v]},
{u, 0, 2 \[Pi]}, {v, -2, 3}]
gives the following graph, with different blues for different negative curvatures.