Exponential towers of $i$'s

Question

It's well known that the expression $i^i$ takes on an infinite set of values if we understand $w^z$ to mean any number of the form $\exp (z (\ln w + 2 \pi i n))$ where $\ln$ is a branch of the natural logarithm function.

Since all values of $i^i$ are real, all values of $i^{i^i}$ (by which I mean $i^{\left( i^i \right)}$) are on the unit circle, and in fact they form a countable dense subset of the unit circle.

I can't figure out what's going on with $i^{i^{i^i}}$, though. I've posted a pdf version of Mathematica notebook at https://jamespropp.org/iiii.pdf containing images starting on page 2. Each image shows the points in the set of values of $i^{i^{i^i}}$ lying in an annulus whose inner and outer radii differ by a factor of 10. For instance, here's what we see in the annulus whose inner and outer radii are 100 and 1000 respectively.

Can anyone see what's going on?

Also, what happens for taller exponential towers of $i$'s? Does the set of values of $$i^{i^{i^{{\ \kern3mu\raise1mu{.}\kern3mu\raise6mu{.}\kern3mu\raise12mu{.}\ }^i}}}$$ become dense in the complex plane once the tower is tall enough? The following picture makes me think that for a tower of height five, the set of values is everywhere dense.

Answer 1

Let $f : \mathcal{P}(\mathbb{C}) \rightarrow \mathcal{P}(\mathbb{C})$ be the function on the powerset of the complex numbers defined by:

$$ f(A) = \left\{ \exp\left( \frac{\pi i}{2}(4n + 1)z \right) : z \in A, n \in \mathbb{Z} \right\} $$

Then your question is asking about $f^k(\{1\})$ and its (topological) closure.

One thing to note is that, for any set $A$, we have $\overline{f(A)} = \overline{f\left(\overline{A}\right)}$, where the overline denotes closure. This follows from the fact that the expression $\exp\left( \frac{\pi i}{2}(4n + 1)z \right)$ is continuous as a function from $\mathbb{C} \times \mathbb{Z}$ to $\mathbb{C}$. So, if we want to study the closure of $f^n(\{1\})$, we are allowed to take the closure after each application of $f$.

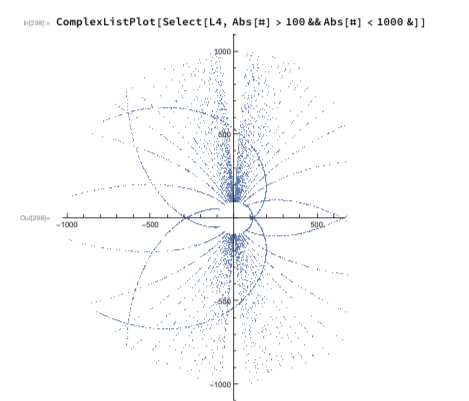
The OP remarked that $\overline{f^3(\{1\})}$ is the unit circle. Then the set:

$$ B :=\left\{ \frac{\pi i}{2}(4n + 1)z : z \in \overline{f^3(\{1\})}, n \in \mathbb{Z} \right\} $$

is precisely the union of a circle of radius $\frac{\pi i}{2} k$ for each odd integer $k$. Next, we need to take the image of this set $B$ under $\exp$. As the function $\exp$ is many-to-one, this is best understood by first reducing $B$ modulo $2 \pi i$ so that it lies in the strip with imaginary part $[-\pi, \pi)$.

Here's a picture of the first six circles in $B \mod 2 \pi i$:

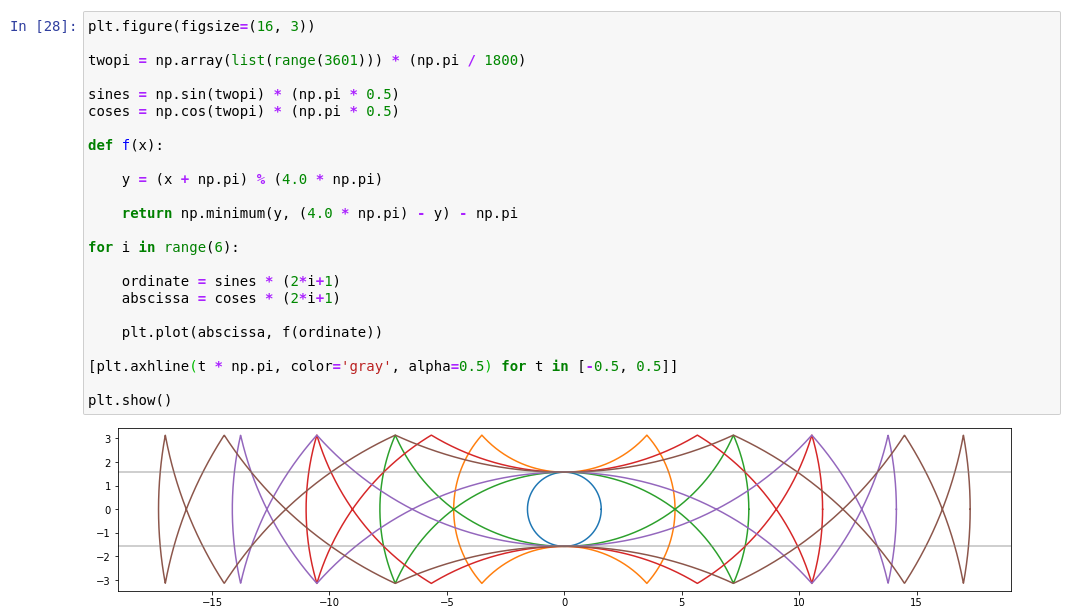
The intersection of $B \mod 2 \pi i$ with a line of fixed real part is a set of points which contains two limit points (the intersection with the light grey lines in the above plot). The closure of $B \mod 2 \pi i$ therefore contains all of these circles together with the two light grey lines (and nothing else).

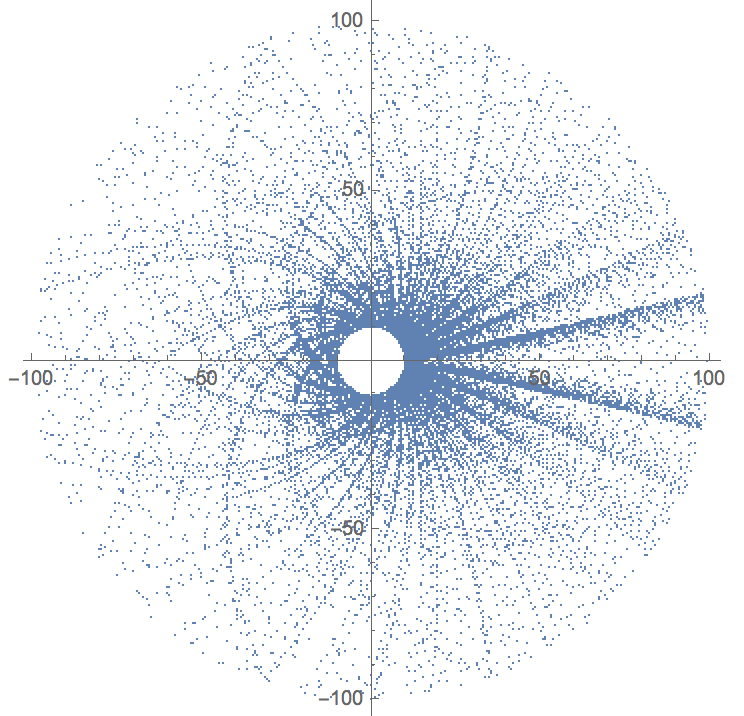
$\overline{f^4(\{1\})}$ is therefore just the image of this set under $\exp$ (viewed as a bijective function from the strip to the punctured complex plane) together with the origin.

Now let's consider $\overline{f^5(\{1\})}$. Recall that the first step is to take the union of lots of homothetic copies of $\overline{f^4(\{1\})}$:

$$ B' :=\left\{ \frac{\pi i}{2}(4n + 1)z : z \in \overline{f^4(\{1\})}, n \in \mathbb{Z} \right\} $$

If we pull this back through the inverse of the $\exp$ map (so we're working on the strip instead of the complex plane), this corresponds to taking the union of lots of translates of $B \mod 2 \pi i$. Specifically, we are interested in the set of points:

$$ \log(B') :=\left\{ w + \log(i) + \log\left(\frac{\pi}{2}(4n + 1)\right) : w \in (B \mod 2 \pi i), n \in \mathbb{Z} \right\} $$

Now, the set $\{ \log\left(\frac{\pi}{2}(4n + 1)\right) : n \in \mathbb{N} \}$ has the very desirable property that the set is unbounded to the right and the gaps between the points become arbitrarily small. Also, the intersection of every horizontal line with the original set $B \mod 2 \pi i$ (the one in the picture above) is unbounded to the left. As such, it follows that their convolution is dense in every horizontal line, and therefore dense in the whole strip.

Consequently, $\overline{f^5(\{1\})}$ is the entire complex plane as you suspected.

Answer 2

The single-valued case of the exponential tower of $i$ was studied by Peter Lynch. The tower converges along three spirals to the fixed point $Q=i^Q$, given in terms of the Lambert W-function by $$Q=\frac{2i}{\pi}W\left(\frac{\pi}{2i}\right)=0.438\cdots+i\,0.361\cdots,$$ see image.