I have some trouble in understanding the significance of some axiom of triangulated category.
If someone could explain to me each axiom with some intuition, and explain to me the intuition behind the translation functor
I would be very grateful !!
Thank you in advance
It is risky to give a motivation for any concept in math and worse that of triangulated category that it is in a sense is a transitional concept form usual mathematics to mathematics up to homotopy.
In any case, I will give a few naive observations with the starting point that the prototypes of triangulated categories are basically the stable homotopy category and the derived category of modules over a ring. It is a good idea to have some notion of at least one of these examples to grasp the ideas behind the axioms.
Distinguished triangles pretend to formalize (co)fibration sequences that in a stable world both concepts agree. From the complexes camp, in the usual category of complexes a short exact sequence yields a long exact sequence in homology, therefore one needs a substitute that works after inverting quasi-isomorphims and very few exact sequences remain.
A distinguished triangle (I much prefer this name over the exact triangle terminology). It has to be a diagram like $$ X \to Y \to Z \overset{+1}\to X $$ Where the label "$+1$" refers to a shift of degrees. Why this shift? Consider the connecting homomorphism in a long exact sequence. In the case of complexes a triangle should arise for an exact sequence of complexes $$0 \to A \to B \to C \to 0.$$ From these one gets maps in homology $H^i(C) \to H^{i+1}(A)$ or otherwise put $H^0(C[i]) \to H^0(A[i+1])$. Notice that instead of $H$ one may use another homological functor. In topology, this is related to a canonical map from the cone $C$ of the map $A \to B$, to the suspension of $A$, i.e. a map $C \to \Sigma A$. The fibration sequence becomes a triangle $$ A \to B \to C \to \Sigma A .$$
This forces the category to be graded: it has to have an automorphism, called "shift" or "suspension", denoted most frequently as $X[1]$ or $\Sigma X$, with the triangles being depicted now as something like $$ X \to Y \to Z \to X[1] $$ The fact that the shift is an automorphism automatically gives a grading into the morphisms so you define $\mathrm{Hom}^i(A,B)$ as $\mathrm{Hom}(A,B[i])$.
Now let's go for the axioms.
TR1. The triangle $X \to X \to 0 \to X[1]$ is distinguished: because the (co)fiber of the identity has to be trivial. Think on the third point as a sort of cokernel, that might as well be interpreted as a kernel when you turn the triangle by TR2. A (co)fiber or (co)kernel exists: any map can be completed to a distinguished triangle. And finally everything isomorphic to a distinguished triangle is a distinguished triangle. I would call this axioms the sanity axioms, they allow everything to make sense.
TR2. The triangles can be turned forward or backward. I would call this the stability axiom. You can't tell a fiber from a cofiber or a kernel from a cokernel in this stable world. If you have a long exact sequence you can't tell where it starts, this axiom reflects that idea.
TR3. A partial map between triangles may be completed to a map of triangles, otherwise said, if you give two maps between the corresponding objects of two triangles (making the corresponding square commutative), one obtains a third one making everything else commutative. Caveat: this map is not unique, and in a sense this makes part of what makes homotopy difficult. To complete, I'd call this the weak functoriality of cones axiom.
TR4. This has a classical name: the octahedral axiom. It was introduced by Verdier whose motivation was to be able to take fractions. He applied it to go form the homotopy category to the derived category avoiding arbitrary long zig-zags, just simple fractions will work. Octahedra are in a sense higher triangles (one step higher in the ladder) and sometimes people look for even higher such diagrams. It is however noteworthy how far one can go with this four axioms plus the existence of arbitrary coproducts, like for instance Brown representability, Verdier quotients and Bousfield localizations.
A view of the octahedral axiom that is enlightening for me is as a version of Noether isomorphisms as it is depicted in the following diagram:
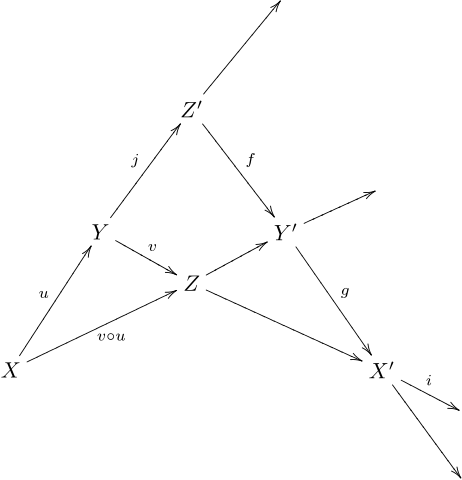
Very roughly: $Z/Y \cong (Z/X)/(Y/X)$.
I should add that this is not the end of then story. In some cases one needs higher diagrams ($n$-triangles) and even worse, one would need some fix for the non-uniqueness of cones. The remedies for this are basically stable $\infty$-categories or alternatively stable derivators. But this takes us far afield into the world of advanced homotopy theory.
TR3 is redundant: it is implied by the other axioms. TR4 is confused by the initial octahedral shape: it starts with a square in which one side is the identity. If one instead starts with a triangle given by a pair of composable arrows and their composition and writes it as a braid, one readily sees that it is just describing the behavior of triangles under composition. The description becomes obvious in the topological framework that was Verdier's starting point (he credited work of Puppe). For the redundancy claim, the braid description, and the behavior of distinguished triangles under products, see https://www.sciencedirect.com/science/article/pii/S0001870801919954 or https://www.math.uchicago.edu/~may/PAPERS/AddJan01.pdf.
