Mapping class group of torus, why is $(ST)^3=S^2$?

Question

In the context of topological quantum field theories, I am interested in the mapping class group of a torus. Here I can consider the torus as a square with identified edges and also decorated with directed curves (see below).

The mapping class group is $\mathsf{SL}(2,\Bbb Z)$ and is generated by $S$ and $T$, which correspond to a 90deg rotation and a Dehn twist respectively. I take the Dehn twist to be along the vertical (meridian). These generators should satisfy $S^2=C$, and $(ST)^3=S^2$, where $C$ is conjugation corresponding to flipping both directions of the torus. The first is easy to see

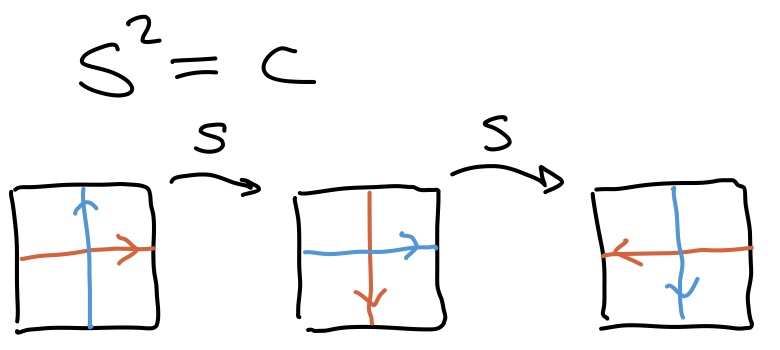
However, I cannot get the second property, here is my working

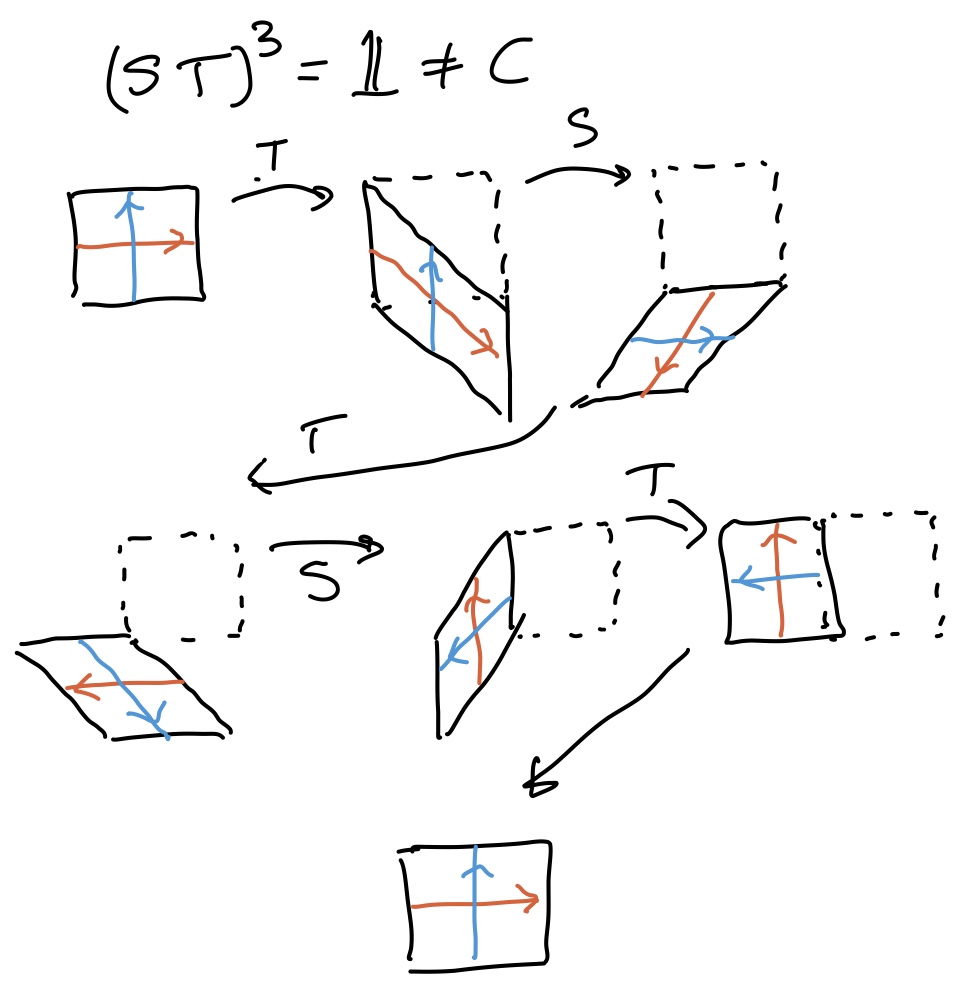
Note in this second case I am not mapping back to the original square using the periodicity to make it clear what transformations I am doing.

$(ST)^3=\Bbb 1$ is true for the modular group $\mathsf{PSL}(2,\Bbb Z)$ but then we should also have $S^2=\Bbb 1$.

Can anyone point out where I am going wrong?

Answer 1

Flip the direction of rotation for $S$, or choose the other meridian for $T$.

We can see this at the level of matrices. Define $$S_1 = \begin{pmatrix} 0 & 1 \\ -1 & 0 \end{pmatrix}, \qquad S_2 = \begin{pmatrix} 0 & -1 \\ 1 & 0 \end{pmatrix}$$ to be the two choices of our rotation matrix. Similarly define $$T_1 = \begin{pmatrix} 1 & 0 \\ 1 & 1 \end{pmatrix}, \qquad T_2 = \begin{pmatrix} 1 & 1 \\ 0 & 1 \end{pmatrix}$$ to be the two choices for our Dehn twist. (I suppose there really are four choices - the off-diagonal entries could just as well be negative, depending on the orientation of the meridian you're Dehn twisting around - but I'll ignore that. It's not too hard to deal with that possibility.)

Then you can do the algebra to check that $S_1^2 = S_2^2 = -\mathrm{Id} = C$, and that $(S_iT_j)^3 = -\mathrm{Id}$ if $i = j$ and $+\mathrm{Id}$ otherwise. This tells you that you need to swap one of $S$ or $T$ as described above.