1
$\begingroup$

Question is ->I am studying research paper: A note on odd zeta values and I am unable to think how to deduce a result which the authors don't prove. This result has to be proved assuming the prime number theorem and it's on Page 12 of the paper :

Prove that $\lim_ {n\to\infty} \frac{\log(\Phi_n) } {n} =\int_0^{1} \rho_0 (t) d(\psi(t) + 1/t) $, where $\psi(t) $ = $\frac {\Gamma'(t) } {\Gamma(t) } $.

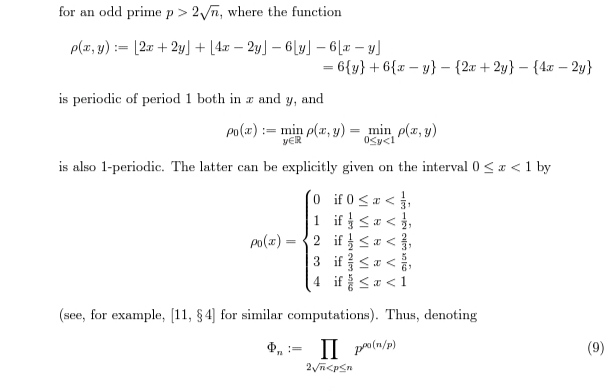
where $\Phi(n)$ and $\rho(n)$ are described in this image: definitions of Phi and rho

Can someone please tell how to prove this result ?

I shall be really thankful.

Improve this question
$\endgroup$
1
  • $\begingroup$ This question already had an accepted answer. Why have you bumped it with an edit? $\endgroup$
    – Yemon Choi
    Commented Jan 6, 2022 at 0:41

1 Answer 1

Reset to default
3
$\begingroup$

If we start by partitioning the range of summation into intervals on which $\rho_0(\frac np)$ is constant, we obtain \begin{align*} \log \Phi_n &= \sum_{2\sqrt n<p\le n} \rho_0\big( \tfrac np \big) \log p \\ &= \sum_{k=6}^{3\sqrt n-1} \sum_{\frac{6n}{k+1} < p \le \frac{6n}k} \rho_0\big( \tfrac np \big) \log p \\ &= \sum_{k=6}^{3\sqrt n-1} \rho_0\big( \tfrac k6 \big) \sum_{\frac{6n}{k+1} p \le \frac{6n}k} \log p = \sum_{k=6}^{3\sqrt n-1} \rho_0\big( \tfrac k6 \big) \big( \theta\big( \tfrac{6n}k \big) - \theta \big( \tfrac{6n}{k+1} \big) \big), \end{align*} where $\theta(x) = \sum_{p\le x} \log p \sim x$ by the prime number theorem. Thus \begin{align*} \log \Phi_n &\sim \sum_{k=6}^{3\sqrt n-1} \rho_0\big( \tfrac k6 \big) \big( \tfrac{6n}k - \tfrac{6n}{k+1} \big) \sim 6n \sum_{k=6}^\infty \frac{\rho_0(k/6)}{k(k+1)}. \end{align*} A similar partitioning calculation on the integral will hopefully lead to the same result.

Improve this answer
$\endgroup$
13
  • $\begingroup$ in the fifth line of your answer how did you change $\sum_{2√n<p$\leq$n$ = $\sum_{k=6}^{3√n-1}$ $\sum_{6n/(k+1) p$\leq$6n/k) . Can you please elaborate why it is right? $\endgroup$
    – Arnold
    Commented Mar 20, 2020 at 5:36
  • $\begingroup$ There was a typo (now fixed). We're just splitting up the interval of summation from the first line into $3\sqrt n-1$ consecutive intervals of summation. $\endgroup$
    – Greg Martin
    Commented Mar 20, 2020 at 7:33
  • 1
    $\begingroup$ On the first line, every prime between $2\sqrt n$ and $n$ appears exactly once, and smaller or larger primes don't appear at all. On the second line, do any primes smaller than $2\sqrt n$ or larger than $n$ appear? Given a prime $p$ between $2\sqrt n$ and $n$, for how many values of $k$ will $p$ appear in the inner sum? $\endgroup$
    – Greg Martin
    Commented Apr 16, 2020 at 15:30
  • 1
    $\begingroup$ We're just splitting up the range of summation into intervals on which the function is simpler. A strong analogy would be the computation $$\int_0^N \lfloor x\rfloor^2\,dx = \sum_{k=0}^{N-1} \int_k^{k+1} \lfloor x\rfloor^2\,dx = \sum_{k=0}^{N-1} k^2 \int_k^{k+1} 1\,dx.$$ $\endgroup$
    – Greg Martin
    Commented Apr 16, 2020 at 15:32
  • 1
    $\begingroup$ @DxdxDxdx: I don't have time for this right now. Also, it is not too much fun to help students understand a particular line from a paper that is not close to my research. I think your best shot is to contact the authors. As I said, they are mathematicians just like me, but they have the advantage that they wrote the paper, and you write a thesis about their work. So don't be shy, just drop them an email! $\endgroup$
    – GH from MO
    Commented May 8, 2020 at 21:32

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .