Regularizing the sum of all primes

Question

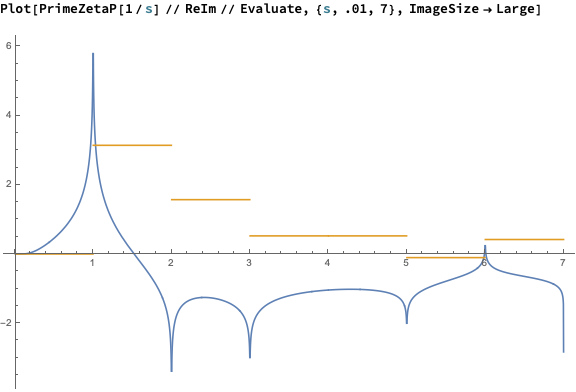
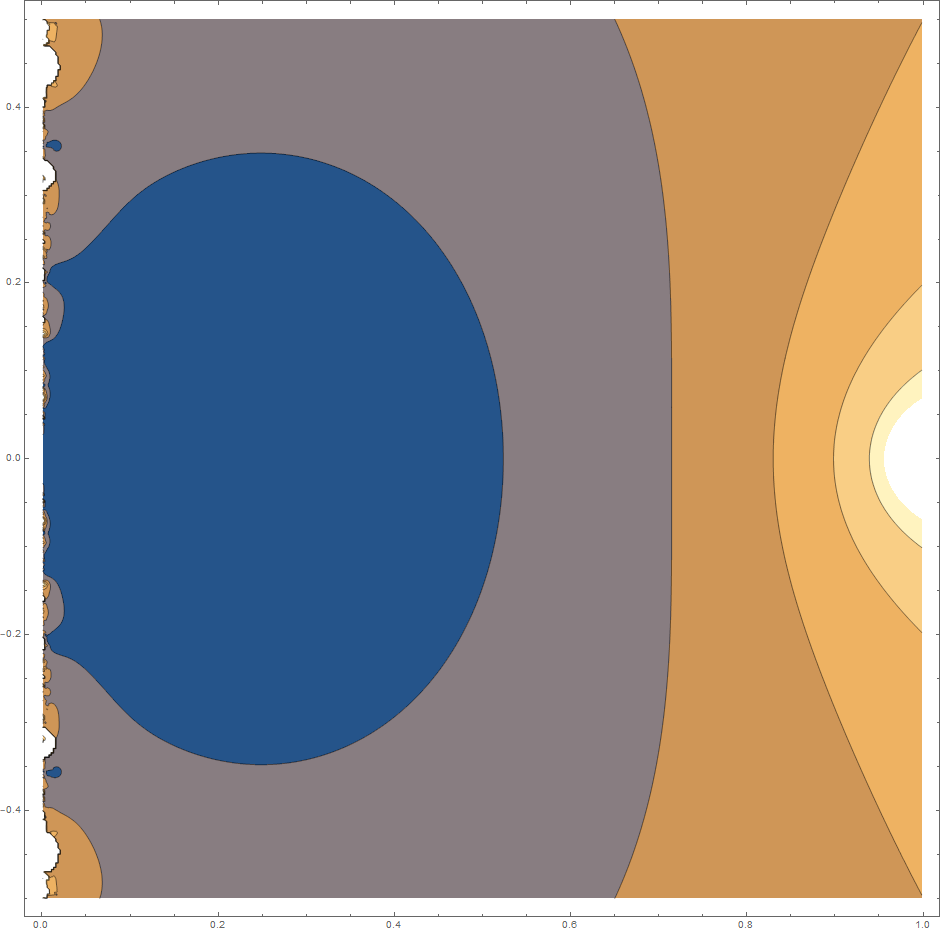
In the spirit of a similar question for the harmonic series, is there a way to regularize the (divergent) sum of all primes?

$$ \sum_{p \text{ prime}} p $$

Neither of these questions obtained a successful regularization:

• Is it possible to assign a value to the sum of primes?

• Is it possible to sum the divergent series with prime coefficients?

• Is it possible to sum the divergent series with prime coefficients?

The prime zeta function, unfortunately, has a natural boundary on the imaginary line that prevents analytic continuation by the usual means.

On the other hand, we know that

$$ \prod_{p \text{ prime}} p = 4\pi^2 $$

To be specific: Is there a different kind of analytic continuation, such as the technique referred to by Gammel in this question, that can be used to continue the prime zeta function beyond its natural boundary?

Another vague idea that occurred to me is, instead of trying to "tunnel through" the natural boundary on the imaginary line, we could try to reach the other side by going around the Riemann sphere in the opposite direction: i.e. through infinity, popping out on the other side of the boundary. My search for something like this yielded Confinement as Analytic Continuation Beyond Infinity by Yamazaki and Yonekura. The image below shows what the function looks like around infinity (on the positive side). Could it be extended to the negative side?

Edit: Möbius inversion yields

\begin{align} P(-1) &= \sum_{p \text{ prime}} p \\ &= \sum_{n \geq 1} \frac{\mu(n)}{n} \log \zeta(-n) \\ &= \sum_{n \geq 1} \frac{\mu(2n)}{2n} \log \zeta(-2n) + \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log \zeta(-(2n-1)) \end{align}

Because the zeta function is zero at the negative even integers, the summands of the first series are undefined, strictly speaking. However, we notice that \begin{align} \sum_{n \geq 1} \frac{\mu(2n)}{2n} \log \zeta(-2n) &= \sum_{n \geq 1} \frac{\mu(2n)}{2n} \log 0 \\ &= \left(\sum_{n \geq 1} \frac{\mu(2n)}{2n}\right) \log 0 \\ &= 0 \log 0 \\ &= \log 0^0 \\ &= \log 1 \\ &= 0 \\ \end{align}

Therefore we can get rid of these problematic terms and end up with \begin{align} P(-1) &= \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log \zeta(-(2n-1)) \\ &= \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log \frac{(-1)^{2n-1} B_{(2n-1)+1}}{(2n-1)+1} \\ &= \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log \left(-\frac{B_{2n}}{2n}\right) \\ &= \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log (-1) - \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log 2n + \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log B_{2n} \\ &= 0 \log (-1) - \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log 2n + \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log B_{2n} \\ &= - \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log 2n + \sum_{n \geq 1} \frac{\mu(2n-1)}{2n-1} \log B_{2n} \\ \end{align}

where the first series converges but the second diverges.

Answer 1

It was too long for a comment and overall as far as possible can be from rigorous so if it isn't helpful just tell me and I'll delete it.

In his blog John Baez talks of particular approach that he learn of to shown that $\sum_{n=1}^{\infty}n=-\frac{1}{12}$ he says that we have the formal series

$f(0)+f(1)+f(2)+...=[(1+e^{D}+e^{2D}+...)f(x)](0)=[\frac{1}{1-e^{D}}f(x)](0)=[\frac{D}{1-e^{D}}F(x)](0)=[(-1+\frac{D}{2}-\frac{D^2}{12}+...)F(x)](0)$

And letting $f(x)=x$ he gets $f(0)+f(1)+f(2)+...=0+1+2+...$ while on the other side it gives $[(-1+\frac{D}{2}-\frac{D^2}{12}+...)\frac{x^2}{2}](0)=[(-\frac{x^2}{2}+\frac{x}{2}-\frac{1}{12}+0)](0)=\frac{-1}{12}$.

---

Now the divergence of harmonic series can be shown be using the Mercator series $\ln(\frac{1}{1-x})=\sum\frac{x^n}{n}$ and letting $x\to1$ which gives $\infty=\ln(\infty)=\sum\frac{1}{n}$

Euler used this to show also the divergence of primes by taking another logarithm of the mercator series and letting again $x\to1$ obtaining $\infty=\ln(\ln(\infty))=\ln(\sum\frac{1}{n})=\ln(\prod_p \frac{1}{1-p^{-1}})=\sum\ln(\frac{1}{1-p^{-1}})=\sum_p\sum_k\frac{1}{kp^k}=\sum_p\frac{1}{p}+\text{constant}$.

Just as $\ln(\frac{1}{1-x})=\sum\frac{x^n}{n}$ for $|x|<1$ we also have that $\ln(\ln(\frac{1}{1-x}))=\sum_p\frac{x^p}{p}$ but only when $x\to 1$ yet we could try to repeat the argument given before.

---

If for $\ln(\frac{1}{1-x})=\sum\frac{x^n}{n}$ we take the derivative and let $x=e^{D}$ then we get the formal series from above $\frac{1}{1-e^{D}}=\sum e^{nD}$.

So doing the same for $\ln(\ln(\frac{1}{1-x}))=\sum_p\frac{x^p}{p}$ gives $\frac{1}{(e^{D}-1)\ln(1-e^{D})}=\sum_p e^{pD}$(+), that is our formal series should be:

$f(2)+f(3)+f(5)+...=[(e^{2D}+e^{3D}+e^{5D}...)f(x)](0)=[\frac{1}{(e^{D}-1)\ln(1-e^{D})}f(x)](0)=[\frac{D}{(e^{D}-1)\ln(1-e^{D})}F(x)](0)=[\text{Taylor series}(\frac{D}{(e^{D}-1)\ln(1-e^{D})})F(x)](0)$

While before we had a finite second derivative $\frac{1}{2!}(\frac{y}{1-e^y})''|_{y=0}=-\frac{1}{12}$

Now we have a undefined second derivative$\frac{1}{2!}(\frac{y}{(e^y-1)\ln(1-e^y)})''|_{y=0}=\text{undefined}$

Answer 2

Alas, it looks like the imaginary axis is the natural analyticity boundary for $P(1/s)$:

Flatten[Table[{x,y,N@Abs[PrimeZetaP[1/(x+I y)]]},{y,-.5,.5,.0025},{x,.001,1,.0025}],1]
//ListContourPlot

gives