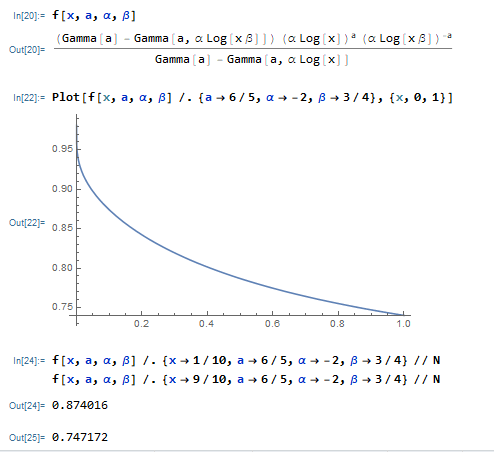
Let $a> 0, \alpha<0$ and $\beta>0$. How to prove that the function: $$f(x)=\frac{(\Gamma(a)-\Gamma(a,\alpha \ln(\beta x))) (\alpha\ln(x))^a}{(\alpha\ln(\beta x))^a (\Gamma(a)-\Gamma(a,\alpha \ln(x)))},$$ is monotonic.
I tried the sign of derivative but is more delicate.