Non-constant holomorphic map onto a smooth curve

Question

Let $\Gamma$ be a smooth projective curve in $\mathbb{P}^2$ and let $U$ be an open neighborhood of $\Gamma$. Denote by $\Gamma_1,\Gamma_2,\ldots,\Gamma_n$ a finite collection of smooth curves intersecting $\Gamma$ transversally. My question is:

Does there exist a non-constant holomorphic function $\pi: U \setminus \bigcup\limits_{i=1}^n \Gamma_i \to \Gamma \setminus \bigcup\limits_{i=1}^n \Gamma_i$ ?

The situation as in the following picture.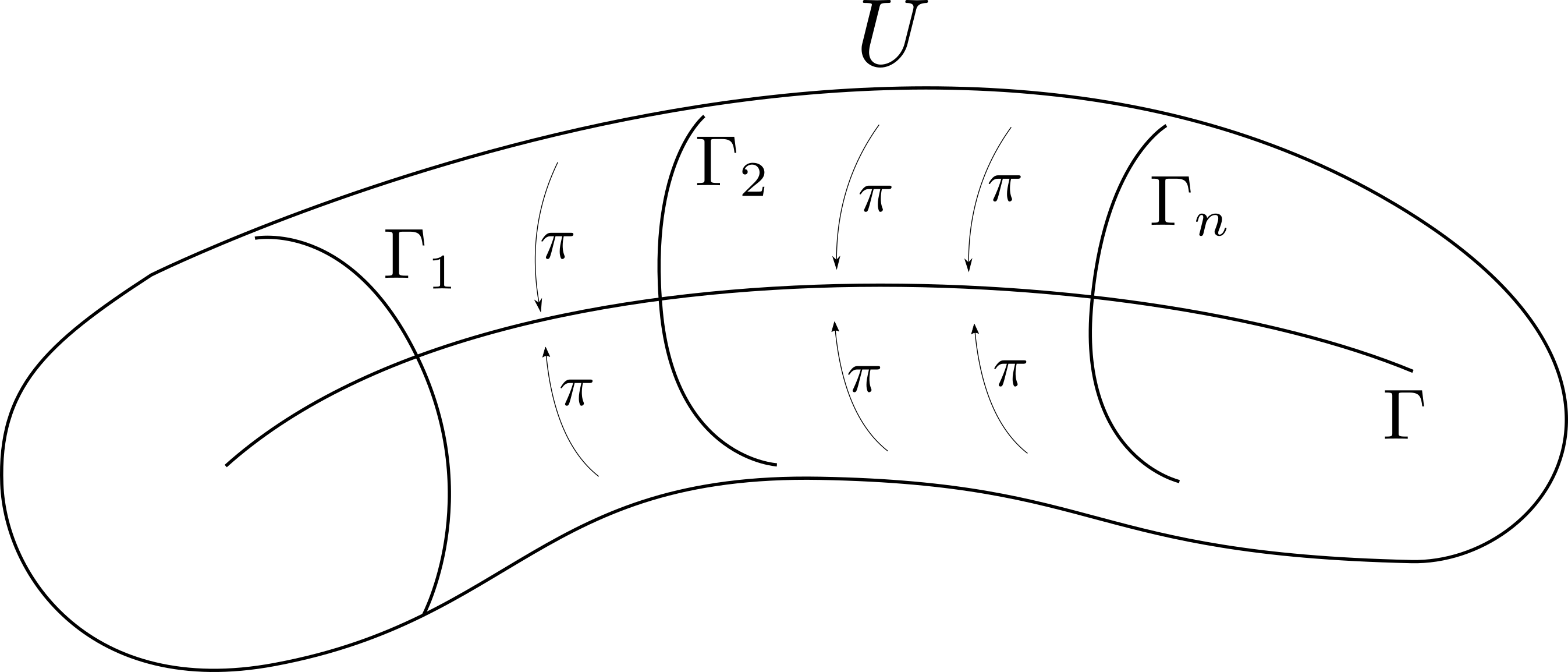

I can build a smooth map $\pi$ easily by partition of unity. Intuitively, $\pi$ seems like the tubular neighborhood projection of $\Gamma$ ? But unfortunately, a holomorphic tubular neighborhood does not exist in general as discussed in Is there any holomorphic version of the tubular neighborhood theorem?. And I realized that even the existence of a non-constant holomorphic map $\pi : U \to \Gamma$ (without removing any curve) is not obvious to me.

Any comment is welcome. Thanks in advance!

Answer 1

Let me show that such a map usually doesn't exist (even if we don't remove any additional curves). Consider the case when $\Gamma$ is a curve of degree $\ge 4$. Then one can slightly perturb $\Gamma$ in $\mathbb CP^2$ to a curve $\Gamma'$ that is not isomorphic to $\Gamma$ (so that it stays in a small neighbourhood of $\Gamma$). If the map $\pi$ would exists, we can restrict it to $\Gamma'$, and it will be extendable to the whole $\Gamma'$. So we get a holomorphic (non constant) map $\pi: \Gamma'\to \Gamma$, which is impossible, since $\Gamma$ and $\Gamma'$ are not isomorphic (and by Riemann-Hurwitz for two curves of the same genus $\ge 2$ a non-constant holomorphic map $\Gamma\to \Gamma'$ exits only if the curves are isomorphic).

This argument can be easily generalised to curves $\Gamma$ of degree $3$, i.e. cubics. The case of conics and lines should be analysed separately (and for lines $\Gamma$ such a map sometimes exists, for example, when all $\Gamma_i$'s are lines transverse to the line $\Gamma$ and intersecting at one point).