6
$\begingroup$

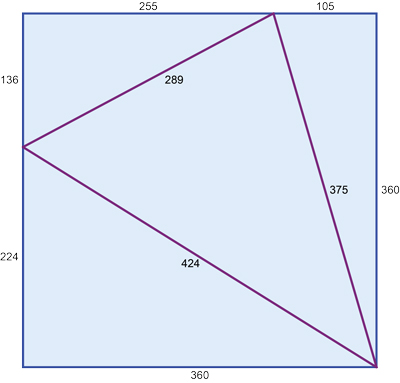
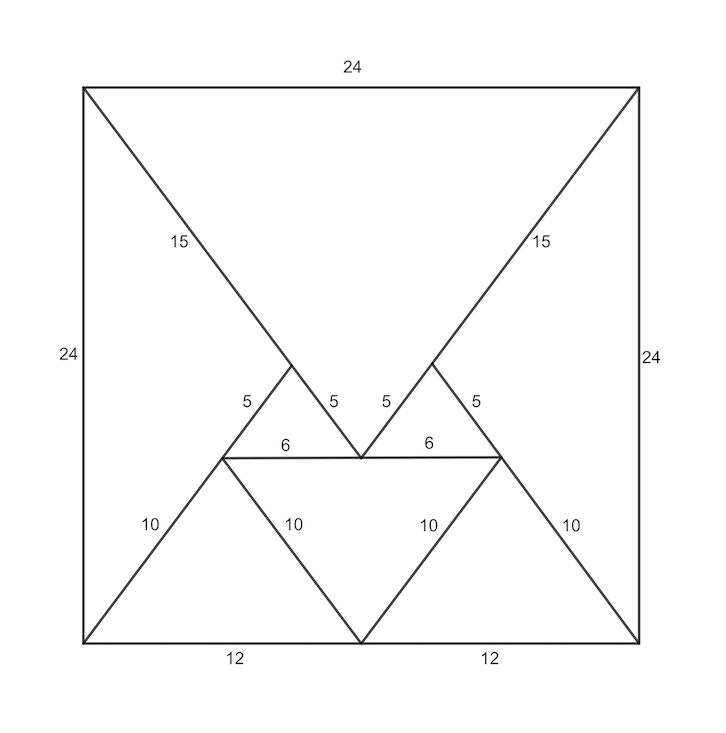
The following questions seem related to the still open question whether there is a point(s) whose distances from the 4 corners of a unit square are all rational.

  1. To cut a unit square into n (a finite number) triangles with all sides of rational length. For which values of n can it be done if at all?

  2. To cut a unit square into n right triangles with all sides of rational length. For which values of n can it be done, if at all?

Remark: If one can find a finite set of 'Pythagorean rectangles' (rectangles whose sides and diagonal are all integers) that together tile some square (of integer side), that would answer this question.

3.To cut a unit square into n isosceles triangles with all sides of rational length. For which values of n can it be done, if at all?

Now, one can add the requirement of mutual non-congruence of all pieces to all these questions. Further, one can demand rationality of area of pieces or replace the unit square with other shapes (including asking for a triangulation of the entire plane into mutually non-congruent triangles all with finite length rational length sides)...

Note: From what has been shown by Yaakov Baruch in the discussion below, cutting the unit square into mutually non-congruent rational sided-right triangles can be done for all n>=4. Indeed, he has shown n=4 explicitly; for higher n, one can go from m non-congruent pieces to m+1 pieces by recursively cutting any of the m right triangular pieces n by joining its right angle to the hypotenuse to cut it into two smaller and mutually similar but non-congruent pieces. That basically settles questions 1 and 2 - the non-congruent pieces case. However, if we need all pieces to be non-congruent and non-similar, the n=4 answer has no obvious generalization to higher n.

References: 1. https://nandacumar.blogspot.com/2016/06/non-congruent-tiling-ongoing-story.html?m=1

  1. On dissecting a triangle into another triangle
Improve this question
$\endgroup$
6
  • 2
    $\begingroup$ Why stop there, and not ask for all ways to cut arbitrary shapes into an arbitrary number of other arbitrary shapes with arbitrary conditions on arbitrary numerical invariants of the shapes? $\endgroup$
    – Gerry Myerson
    Commented Feb 3, 2020 at 21:06
  • $\begingroup$ There are questions on point sets where all or maximally many distances have to be rational. Here the attempt was to think of a family of questions that apply rationality of distances only locally - only sides of each single piece need to be rational; the rest of the distances are free. $\endgroup$
    – Nandakumar R
    Commented Feb 4, 2020 at 4:17
  • 1
    $\begingroup$ 2. You can cut a square into 12 $1/3\times1/4$ rectangles, each of which can be bisected by a diagonal into 2 rational triangles; $n=24$ here. You can then merge some adjacent triangles to form larger triangles and reduce $n$ for both 1. and 2. $\endgroup$
    – Yaakov Baruch
    Commented Feb 4, 2020 at 9:11
  • 2
    $\begingroup$ As for $n=4$, one can split a $360\times 360$ square into 3 right triangles along the perimeter (360-224-424, 360-105-375, 255-136-289) and a middle triangle (375-289-424). $\endgroup$
    – Yaakov Baruch
    Commented Feb 4, 2020 at 11:34
  • 2
    $\begingroup$ @YaakovBaruch, those are worth putting in an answer $\endgroup$
    – user44143
    Commented Feb 4, 2020 at 12:17

1 Answer 1

Reset to default
8
$\begingroup$

@YaakovBaruch's beautiful construction for $n=4$:

          SquarePartition

Edit by Yaakov Baruch. A partition with 8 isoceles triangles (I'm sure not minimal): 8 isoceles

Improve this answer
$\endgroup$
10
  • 2
    $\begingroup$ So the triangles are (8-15-17) * 17, (7-24-25) * 15, and (28-45-53) * 8. That's the first time I remember seeing 28-45-53. $\endgroup$
    – user44143
    Commented Feb 4, 2020 at 13:17
  • 1
    $\begingroup$ Drawing heights in right triangles splits them to produce solutions for $n\ge 5$ too. $\endgroup$
    – Yaakov Baruch
    Commented Feb 4, 2020 at 13:47
  • 1
    $\begingroup$ (It's always amazing what mathematicians find beautiful...) $\endgroup$
    – Yaakov Baruch
    Commented Feb 4, 2020 at 13:58
  • 2
    $\begingroup$ Searching around the internet I see here that the rational distance problem is known to not have solutions on the square's boundary. So that rules out dissections for $n=3$. $\endgroup$
    – Yaakov Baruch
    Commented Feb 4, 2020 at 14:25
  • 1
    $\begingroup$ Notice that the middle triangle has rational area, since the other 3 do and the tota areal is 1; any height of the middle triangle therefore splits it into rational ones (as the sum of 2 square roots is only rational if both roots are). $\endgroup$
    – Yaakov Baruch
    Commented Feb 5, 2020 at 8:11

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .