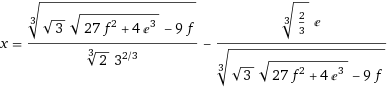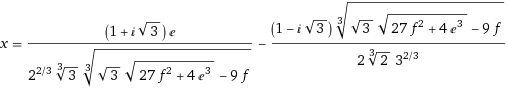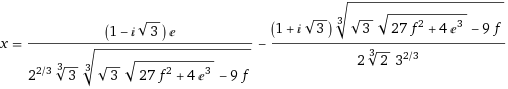I am trying to solve the following integration, where $a,b,c,d,e$ and $f$ are constants:
$$I=\int\frac{x^4+ax^3+bx^2+cx+d}{x^3(x^3+ex+f)}dx$$
I tried to solve the integral using the following two methods, but both seemed to be very much complicated:
Method 1:
Using partial fraction decomposition by calculating the three roots of the denominator. Among the three roots, one root is real and the other two roots are complex (both are complex conjugates of each other). However, the roots are too much complicated and are as follows:
Method 2:
I tried to expand the denominator using binomial series as follows: $$I=\int\frac{\frac{1}{x^2}+\frac{a}{x^3}+\frac{b}{x^4}+\frac{c}{x^5}+\frac{d}{x^6}}{1+\frac{e}{x^2}+\frac{f}{x^3}}dx$$ Then writing $\epsilon=\frac{e}{x^2}+\frac{f}{x^3}$, the above integral becomes $$I=\int\left(\frac{1}{x^2}+\frac{a}{x^3}+\frac{b}{x^4}+\frac{c}{x^5}+\frac{d}{x^6}\right)\left(1+\epsilon\right)^{-1}dx$$ For a quite good approximation, it is required to expand the binomial series up to $\epsilon^{11}$, i.e. $40$ terms in the expression for the integral and this is too much cumbersome.
Even Mathematica expresses the result as a conditional expression and it is required to provide the range of the constants $a,b,c,d,e$ and $f$ to obtain the exact expression.
QUESTION:
Is it possible to solve the integration analytically using any other suitable method? If no such methods are possible, do there exist any approximation technique that might work?