Regarding definition of Kobayashi length [closed]

Question

I am totally new in the area of complex geometry. I have been reading this paper by Steven Krantz The Carath ́eodory and Kobayashi Metrics and Applications in Complex Analysis. In the definition of the Kobayashi length (Section 3)(Please find screenshot), I have the following doubts: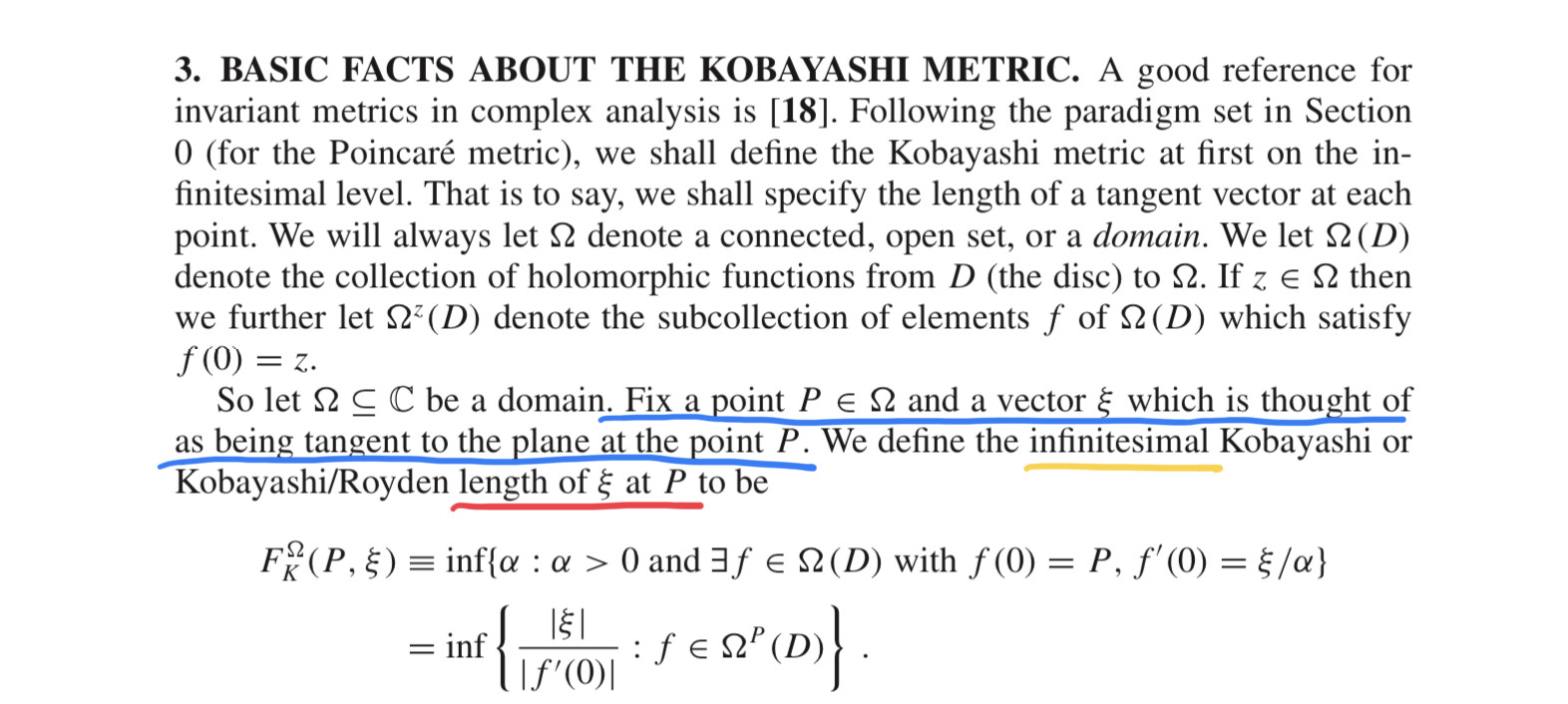

1) Fix a point $P \in \Omega$ and a vector $\xi$ which is thought of as being tangent to the plane at the point $P$

They haven’t mentioned any particular plane in the paper since the beginning so should I assume its the complex plane? What does $\xi$ bieng tangent to the plane at $P$ mean?

2) We define the infinitesimal Kobayashi or Kobayashi/Royden length of $\xi$ at $P$ to be

What is the significance of the word infinitesimal here. And is the length of $\xi$ measured from the origin to the point $P$?

Answer 1

The vector $\xi$ is tangent to the complex line spanned by $\xi$. The significance of "infinitesimal" is that this definition defines a length of a tangent vector (in the sense of differential geometry), which is thought of traditionally as a point infinitesimally near to $P$. There is another definition, which you should find in the same book, of a metric, which will actually give distances between points of $\Omega$ (at least, say, for any bounded domain $\Omega$ in complex Euclidean space). The length is not measured from the origin to $P$, but from the origin of $T_P \Omega$ to $\xi$, i.e. it is the length of a tangent vector, similar to how one measures lengths of tangent vectors in a Riemannian metric. More precisely, the infinitesimal Kobayashi length is a seminorm on each tangent space. It might help to free your intuition if you forget that $\Omega$ is a domain in a complex vector space, and define the infinitesimal Kobayashi length on any complex manifold $\Omega$. There is no reason why $\Omega$ should contain any origin, or have a meaningful notion of distance from any origin.