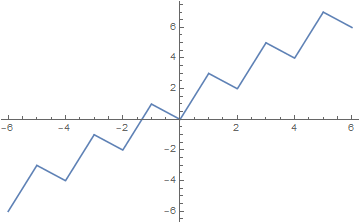
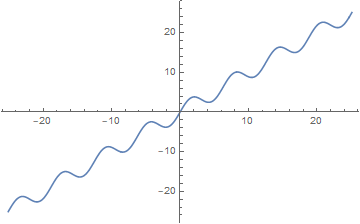
Let $f \in C(\mathbb R,\mathbb R)$, $\text{degree}(f)=\sup\limits_{a \in\mathbb R} \{ \text{card}(f^{-1}(\{a\})) \}$
Is it true that $\forall f \in C(\mathbb R,\mathbb R),\text{degree}(f)=k\in\mathbb N$ iff,
there exists $g$ increasing function and $P \in \mathbb R[x]$, $\text{degree}(P)=k$ with $f=P\circ g$?
The answer is no. Indeed, let $h$ be the periodic function with period $2$ such that $h(x)=2|x|$ if $|x|\le1$. Let $$f(x):=x+h(x)=x+2 \left| x-2 \left\lfloor \frac{x+1}{2}\right\rfloor \right| $$ for all real $x$. Here is a graph of $f$:
Then the function $f$ is of degree $3$. However, if we had $f=P\circ g$ for some polynomial $P$ of degree $3$ and some increasing function $g$, then $f$ would switch at most twice on $\mathbb R$ from increasing to decreasing, or vice versa -- whereas $f$ actually has infinitely many such switches.
One can similarly use $f(x)=x+2\sin x$ instead, with this graph:
