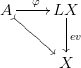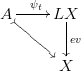Let $LX$ denote the free loop space on $X$. We have an evaluation map $ev\colon LX\to X$ and we have an inclusion $X\hookrightarrow LX$ (where $x\in X$ is mapped to the constant loop at $x$).
Suppose that $A\subset X$ is a cofibration, where $X$ is simply connected (I wouldn't mind to assume that $A$ is also simply connected if that would simplify anything). Let $\varphi\colon A\to LX$ be a map that makes the following diagram commutes:
In other words: $\varphi$ sends $a\in A$ to a loop in $X$ that is based in $a$.
My question: Is it possible to contract all loops simultaneously but in a "basepoint preserving" way (see below)? I.e., is is possible to find a homotopy $\psi_t\colon A\to LX$ where $\psi_0=\varphi$ and $\psi_1$ is the inclusion $A\hookrightarrow LX$, where
commutes for every $t\in [0,1]$?