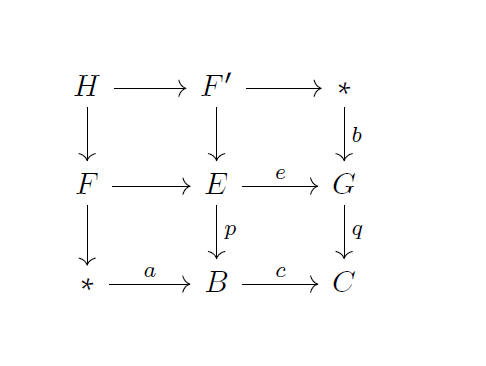
Suppose given a commutative diagram in the $\infty$-category of spaces, as the one depicted below, where all but the bottom-right squares are pullbacks. Is it true that $H$ is (equivalent to) the fiber of the map induced between the fibers of $p$ and $q$ (or, equivalently, $e$ and $c$).
In particular, I would like to know whether it is true or not that $H$ is contractible iff the bottom-right square is a pullback.
Edit: I realized what I actually meant is that $H(a,b)$ (in place of $H$, and defined by such diagram) is contractible iff the map on homotopy fibers induced by $a$ (or, equivalently, by $b$) is a weak equivalence. But I guess the same counterexample should work.