Are these moments related to any usual distribution?

Question

Knowing the moments of a random variable, we are wondering if we can express it in terms of some usual distribution (beta, uniform...), maybe as a product of distributions.

$X$ is a $[0,1]$-valued random variable with the following moments:

$\mathbb{E}[X^k]=\frac{2}{3(k+2)}+\frac{8}{(k+1)(k+2)^2(k+3)}.$

Is it correct to think of $X$ as a mixture of two distributions, one being a size biaised distribution? Is the second term related to some common distribution?

Answer 1

Note that for all real $k\ge0$ and $a>0$ we have $$\frac1{k+a}=\int_0^1 x^k x^{a-1}\,dx. $$ Differentiating in $k$, we further get $$\frac1{(k+a)^2}=-\int_0^1 x^k \ln x\, x^{a-1}\,dx. $$ So, doing the partial fraction decomposition, we have $$\frac2{3(k+2)}+\frac8{(k+1)(k+2)^2(k+3)} =\frac2{3(k+2)}-\frac4{k+3}+\frac4{k+1}-\frac8{(k+2)^2} =\int_0^1 x^k f(x)\,dx=EX^k $$ if the pdf of $X$ is given by $$f(x):=4+\frac{2x}3-4 x^2+8x\ln x \tag{1} $$ for $x\in(0,1)$.

Note also that any compactly supported distribution on $\mathbb R$ is uniquely determined by its moments, because then the characteristic function of the distribution is uniquely determined by the moments; here one can use the moment generating function instead of the characteristic one.

So, here the distribution of $X$ with its pdf $f$ given by (1) is uniquely determined by the moments.

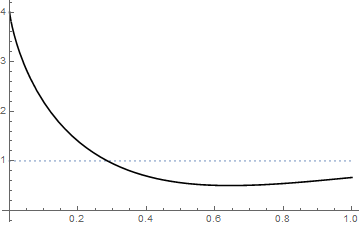
Note further that the function $f$ defined by (1) is indeed a pdf. First here, $\int_0^1 f(x)\,dx=1$. Also, $f''(x)=8/x-8>0$ for $x\in(0,1)$ and $f'(3/5)=-0.219\ldots<0$. So, $f$ is convex on $(0,1)$ and hence $$f(x)\ge f(3/5)+f'(3/5)(x-3/5)\ge f(3/5)+f'(3/5)(1-3/5) =0.42006\ldots>0 $$ for all $x\in(0,1]$. Here is the graph of $f$:

This method should work for any compactly supported distribution on $\mathbb R$ whose $k$th moments are given by a rational (in $k$) expression.

Answer 2

I just found what I was looking for: if I consider two independent random variables $Y$ and $Z$ such that $Y$ has law Beta(1,2) and $Z$ has law Beta(2,2), then their product $W:=YZ$ satifies

$\mathbb{E}[W^k]=\frac{2}{(k+1)(k+2)}\frac{6}{(k+2)(k+3)}$.

So the law of $X$ is a mixture of a size biaised distribution (which is also the Beta(2,1) distribution) and the product distribution of a Beta(1,2) and a Beta(2,2).

If you see anything that could complete this answer, please let me know.