I am thinking about how formally smooth maps of schemes relate to factorization systems.
Let $C$ be the category of schemes. Let $E$ be the class of morphisms of schemes consisting of closed immersions $X \rightarrow X_{th}$, where $X$ is determined by a nilpotent sheaf of ideals on $X_{th}$.
Let $M$ be the class of morphisms in $C$ which lift against $E$. i.e., the maps $f : Y \rightarrow Z$ such that, for each $g : X \rightarrow X_{th}$, and for each commutative diagram as below,
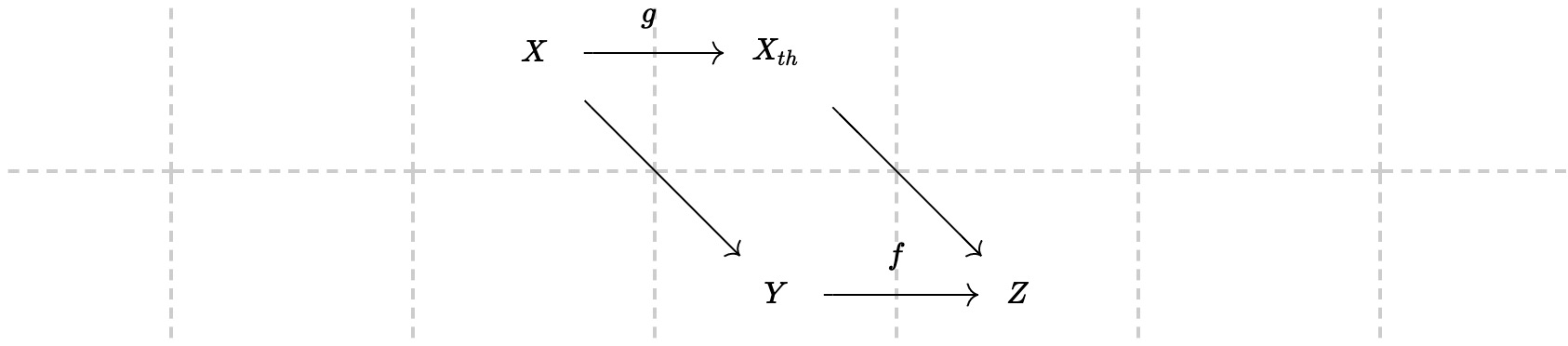there exists a lift like below:
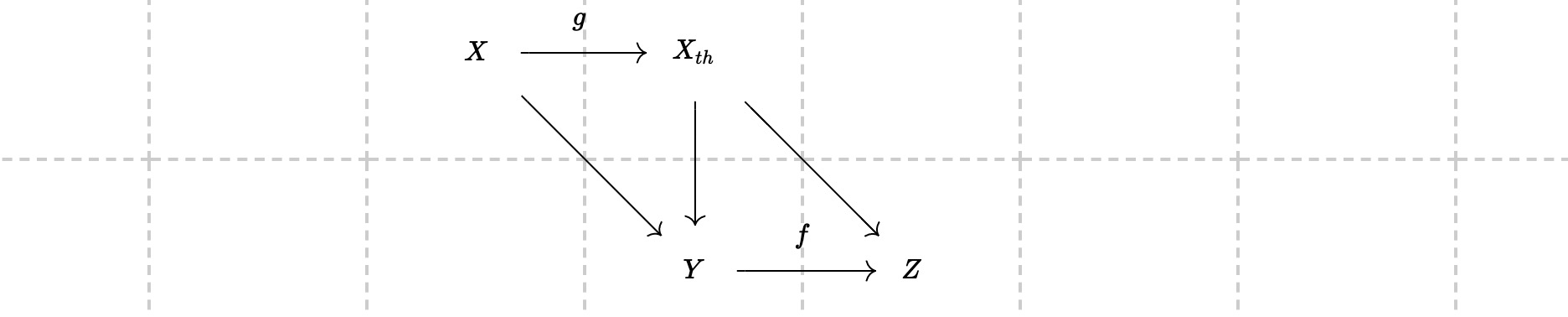These are called formally smooth morphisms.
Let $\overline{E}$ be the class of morphisms of schemes which lift against $M$, i.e., the maps $g : X \rightarrow X_{th}$ such that, for each map $f : Y \rightarrow Z$ in $M$, and for each commutative diagram as below,
there exists a lift like below:
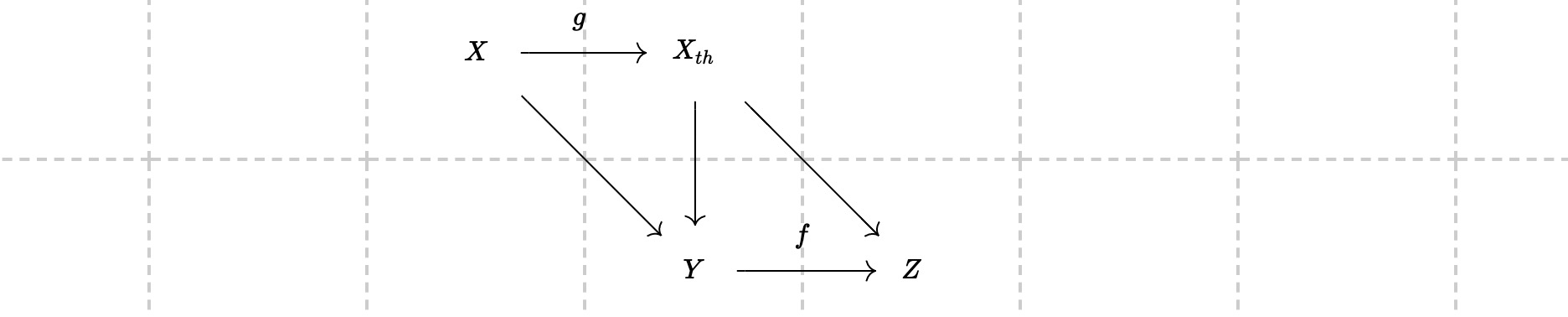
My questions are:
Question: What is this class of maps $\overline{E}$? Obviously it contains the elements of $E$, but what other maps does it contain?
Question: Can we factor each map of schemes $f : X \rightarrow Y$ as the composition $m \circ e$ for $m \in M$ and $e \in \overline{E}$? That is, can we factor any map as a map in $\overline{E}$ followed by a formally smooth map?