-1
$\begingroup$

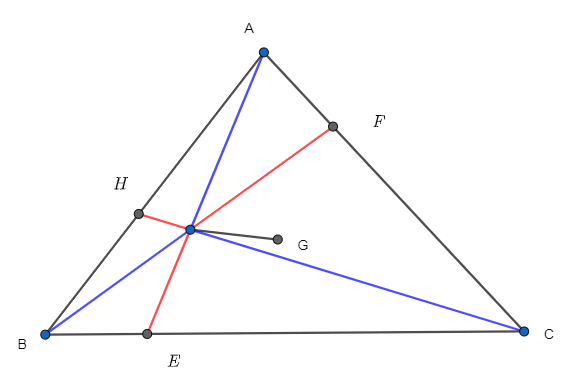
Ono's inequality is true for acute triangle but false with general triangles. The inequality as follows is false with general triangls but I think it true with acute triangle (follows answer by Fedor Petrov)

The inequality as follows like the Erdős–Mordell inequality, I found a year ago, and sent the inequality to some people but I no have a proof until now.

Let $ABC$ be acute triangle (replaced general triangle by acute triangle following Fedor Petrov's answer) with the centroid $G$, $D$ is the point in the plane. Let $EFH$ is a cevian triangle of $D$. How can prove that:

$$DA+DB+DC \le 2(DE+DF+DH)+3DG$$

enter image description here

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ Do not use notation $G$ twice $\endgroup$
    – Fedor Petrov
    Commented Sep 25, 2019 at 9:55
  • $\begingroup$ I will edit as soon as posible $\endgroup$
    – Đào Thanh Oai
    Commented Sep 25, 2019 at 12:18
  • 3
    $\begingroup$ I don't understand changing the question after there has been an accepted answer. Better, I think, to post a new question (and revert this one to its previous form). $\endgroup$
    – Gerry Myerson
    Commented Sep 25, 2019 at 23:22
  • $\begingroup$ Because I think create a new topic from old topic , some one don't like the topic. I am sorry @GerryMyerson $\endgroup$
    – Đào Thanh Oai
    Commented Sep 26, 2019 at 2:07
  • $\begingroup$ As long as you link the two posts, and explain clearly how they differ and why you're doing it, I don't think anyone would mind. What are you going to do now, if someone gives a thorough answer to the acute triangle question? Unaccept Fedor's answer? $\endgroup$
    – Gerry Myerson
    Commented Sep 26, 2019 at 7:23

2 Answers 2

Reset to default
6
$\begingroup$

I am afraid it is false: take isosceles triangle $ABC$ with angle $C$ close to $\pi$, and choose $D$ very close to $C$.

Improve this answer
$\endgroup$
2
$\begingroup$

Intriguingly, there is an equality involving exactly the quantities in your post: $$DA^2+DB^2+DC^2=4(GD^2+GE^2+GF^2) + 3DG^2. $$

I am adding this in case you don‘t know of it—-otherwise, just ignore. I imagine this could be used to modify your conjecture or to specify conditions on the triangle for which it is true but I haven‘t tried to do this.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .