Reference request: Reidemeister type moves for immersed curves on surfaces

Question

Preliminaries

Let $\Gamma$ be a closed $1$-manifold (i.e. a union of finitely many circles) and let $\Sigma$ be a closed $2$-manifold (i.e. a surface). I'll adopt the following terminology.

Definition 1. A clean immersion $\iota:\Gamma \to \Sigma$ is an immersion such that

• $\iota$ restricts to an embedding on each component of $\Gamma$ and
• the self-intersections of the image consist of transverse double points.

Reidemeister-Type Moves For Clean Immersions

Consider the following two analogues of the Reidemeister moves. The first is an analogue of Reidemeister 2.

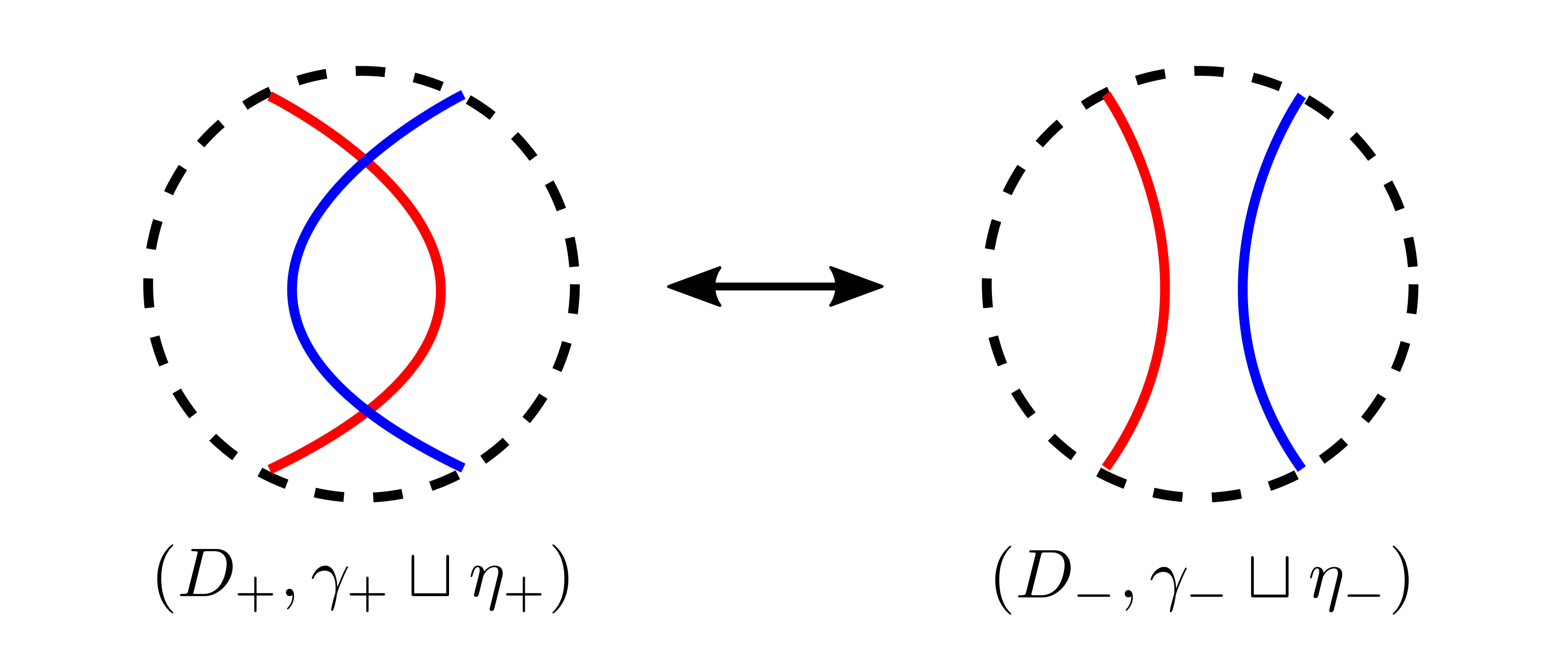
Definition 2. A two-point move on $\iota$ is any modification of $\iota$ to a new clean immersion $\iota'$ as follows. Let $D \subset \Sigma$ be a disk such that $(D,D \cap \iota(\Gamma))$ is diffeomorphic to either $(D_+,\gamma_+ \cup \eta_+)$ or $(D_-,\gamma_-\cup\eta_-)$, which are given by the pictures

Then, swap $(D_+,\gamma_+\cup\eta_+)$ for $(D_-,\gamma_-\cup\eta_-)$ (or visa-versa).

The second is an analogue of Reidemeister 3.

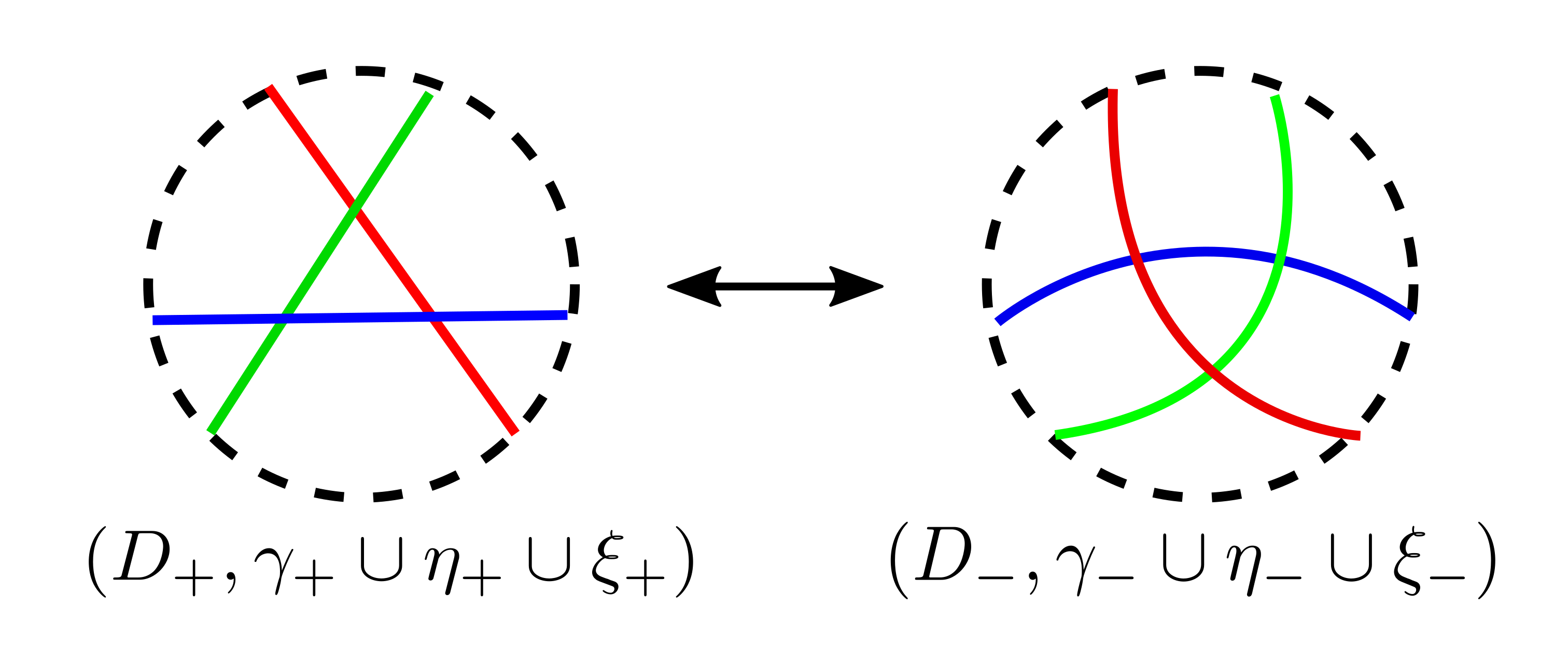
Definition 3. A three-point move on $\iota$ is any modification of $\iota$ to a new clean immersion $\iota'$ as follows. Let $D \subset \Sigma$ be a disk such that $(D,D \cap \iota(\Gamma))$ is diffeomorphic to either $(D_+,\gamma_+ \cup \eta_+ \cup \xi_+)$ or $(D_-,\gamma_-\cup\eta_-\cup\xi_-)$, whcih are given by the pictures

Then, swap $(D_+,\gamma_+ \cup\eta_+\cup\xi_+)$ for $(D_-,\gamma_-\cup\iota_-\cup\xi_-)$ (or visa-versa).

A Reidemeister-Type Theorem

I believe the following result is true of homotopies of clean embeddings.

Proposition. If $\iota_0:\Gamma \to \Sigma$ and $\iota_1:\Gamma \to \Sigma$ are homotopic, then they are related by a sequence of two and three point moves.

Here is a sketch of the argument that I have in mind.

Proof: Pick an smooth homotopy $\iota:\Gamma \times I \to \Sigma$. We can choose this isotopy so that it is an isotopy embeddings on each component. Now consider the trace $I:\Gamma \times I \to \Gamma \times I$ given by $I(s,t) = (\iota_t(s),t)$.

By Papakyriakopoulos (?) we can perturb $I$ away from a neighborhood of the boundary of $\Gamma \times I$ to a map $I'$ with only arcs of double points, isolated triple points and isolated branch points, and where the double point arcs must have boundary on the branch points. Also, we can pick $I'$ so that the components of $\Gamma$ are still embedded. In particular, there can be no branch points and so the double point arcs must be closed curves.

We can perturb again to an embedding $I''$ where the double point circles so that they are transverse to the projection map to $[0,1]$. Then these represent (forward and backward) two-point moves. In a similar manner, the triple points correspond to the triple-point moves.

Main Questions

My issue is that I can't for the life of me find a reference for Proposotion 1! Also I think it would be ideal for the particular project I'm working on to not include a fleshed out version of the proof I discussed above.

Question 1. Where can I find a reference for Proposition 1? Or a statement from which I can conclude Proposition 1 as a quick Corollary?

Also, just to be sure...

Question 2. Proposition 1 is correct, right? Or am I just totally off base?

Answer 1

This isn't true as stated. What is true is that if $\gamma$ and $\gamma'$ two homotopic curves that are in minimal position (i.e. each contains the minimal number of self-intersections in their homotopy class), then $\gamma$ and $\gamma'$ can be connected by a sequence of isotopies and Reidemeister III moves. This was proved in

J. Hass and P. Scott, Shortening curves on surfaces, Topology 33 (1994), no. 1, 25–43.

This paper does not state the precise result I describe above, but see

J. M. Paterson, A combinatorial algorithm for immersed loops in surfaces, Topology Appl. 123 (2002), no. 2, 205–234.

for an alternate proof that states things as I did.

What is needed, then, is a set of moves that take a curve and reduce it to minimal position. Unfortunately, it is not true that you can do this with Reidemeister I and II moves (I am assuming that you meant to include Reidemeister I, by the way), even if you make the obvious modification to allow the curve to pass through the "modification region" in several other arcs that are not involved in the moves. What is needed are moves that eliminate "singular monogons" and "singular bigons" (which can't be assumed to be embedded, as in the usual Reidemeister moves). See the paper

J. Hass and P. Scott, Intersections of curves on surfaces, Israel J. Math. 51 (1985), no. 1-2, 90–120.

for a precise statement and proof (plus examples showing why this is complicated).

Answer 2

Ok, here's a satisfactory answer to my question using the papers that Andy posted (I'm just posting this for clarity). As Andy pointed out, the result is not true if the components of $\Gamma$ are allowed to not be embedded.

Proof: First assume that $\iota_0$ and $\iota_1$ be homotopic clean immersions with the minimal intersection property. That is, $\iota_0(\Gamma)$ and $\iota_1(\Gamma)$ have the minimal possible number of transverse double points in their homotopy class. In Paterson's paper [1], they prove that any minimal two such minimal transversely self-intersecting immersions are ambiently isotopic after a sequence of three-point moves (see p. 231-232 and Lemma 3.4).

Thus it suffices to show that $\iota_0$ and $\iota_1$ can be isotoped to minimal clean immersions $\iota_0'$ and $\iota_1'$ through two-point moves. This is implied immediately by Lemma 3.1 of [2], which states that if $\iota_0$ (for instance) does not minimize intersections, then there is an inner-most $2$-gon (i.e. a copy of $(D_+,\gamma_+ \cup \eta_+)$ as above) on which one can perform a 2-point move to decrease the self-intersections by $1$.

[1] Paterson, J. M., A combinatorial algorithm for immersed loops in surfaces, Topology Appl. 123, No. 2, 205-234 (2002). ZBL1025.57025.

[2] Hass, Joel; Scott, Peter, Intersections of curves on surfaces, Isr. J. Math. 51, 90-120 (1985). ZBL0576.57009.