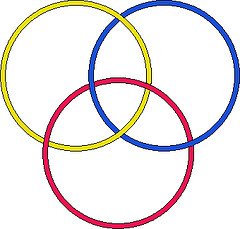4
$\begingroup$

It is know that Borromean rings can be detected by Milnor invariant
$$ \bar{\mu}(\gamma_1,\gamma_2,\gamma_3)= \# (\Sigma_1 \cap \Sigma_2 \cap \Sigma_3)-\frac{1}{2}\sum_{I,J,K}\epsilon_{IJK} \sum_{\scriptsize\begin{array}{c}a\in \gamma_{I}\cap \Sigma_K \\ b\in \gamma_{I}\cap \Sigma_J\\ x_a>x_b \end{array}}(-1)^{\epsilon(a)+\epsilon(b)} $$ or Milnor's triple linking number. See Refs:

  • B. Mellor and P. Melvin, A geometric interpretation of milnor’s triple linking numbers, Algebraic & Geometric Topology 3 (2003) 557–568, [math/0110001].

  • Braiding Statistics and Link Invariants of Bosonic/Fermionic Topological Quantum Matter in 2+1 and 3+1 dimensions Putrov, Wang, Yau; 2016 Annals Phys. 384 (2017) 254-287 (2017-09) DOI: 10.1016/j.aop.2017.06.019 e-Print: https://arxiv.org/abs/1612.09298

enter image description here

From 1612.09298: It is easy to evaluate for the Borromean rings link. Consider realization of Borromean rings shown in Figure with natural choice of Seifert surfaces $\Sigma_I$ lying in three pairwise orthogonal planes. It is easy to see that the first term in is $1$ while all other terms vanish. Terms are defined in 1612.09298.

My question: The above well-known Milnor invariant is evaluated in $S^3$, and there are three $S^1$ circles. Can we define a link invariant, such that we have a set of Borromean lines (three of $R^1$) link in a infinite larger space $R^3$? (I think I can define the 3 lines along the $T^3$ via a surgery.) But I wish to have the following conditions satisfy:

  • triple linking number defined in $R^3$.

  • The three $R^1$ lines are extended in infinity in the space $R^3$.

  • the three $R^1$ are linked in the similar manner as Borromean rings (or lines).

Comments added: For instance, two $S^1$ circles in $R^3$ can form a Hopf link. One can stretch one $S^1$ into two parallel $R^1$ lines in $x$ direction. And we further stretch the other $S^1$ into two parallel $R^1$ lines in $y$ direction, where one of the $y$ line lies in between the two $x$ lines. These four $R^1$ lines form nontrivial linking, which we interpret as an infinite version of the Hopf link. Our question is whether there exists an infinite version of the Borromean ring.

Any Refs/comments are welcome!

If the answer is negative, can we give a proof for impossibility?

Improve this question
$\endgroup$
3
  • $\begingroup$ I'm maybe missing something, but there are no obstructions that would limit three mutually nonintersecting, mutually non-parallel $\mathbb{R}^1$ lines from winding up in any configuration whatsoever, so there's no way of distinguishing 'linked' from 'unlinked' lines in a way that would be invariant even under linear transformations. $\endgroup$
    – Steven Stadnicki
    Commented Sep 11, 2019 at 20:47
  • 1
    $\begingroup$ @StevenStadnicki Thanks! A comment is added in the end, which explains what we mean by $R^1$ lines forming a Hopf link. The question is then about an analogous version for Borromean ring. $\endgroup$
    – user34104
    Commented Sep 11, 2019 at 21:19
  • $\begingroup$ Thank you - the comment is very helpful! Note that just like in the Hopf case, you'll likely need to use two copies of $\mathbb{R}^1$ for each circle, so you may want to talk about six copies of $\mathbb{R}^1$, not three... $\endgroup$
    – Steven Stadnicki
    Commented Sep 12, 2019 at 3:02

0

Reset to default

You must log in to answer this question.