With Ilkka Törmä, we proved that the Grigorchuk group $G$ admits infinite order matrices over any field $K$ and for every dimension $d$. For all claims about the Grigorchuk group and omitted definitions, see e.g. the paper "Topological full groups of minimal subshifts with subgroups of intermediate growth" by Nicolás Matte Bon.
If $\mathrm{char} \; K \neq 2$ then $p = a+b+c+d \in K[G]$ has infinite multiplicative order.
Proof. Recall the form of the Schreier graphs of the Grigorchuk group for its natural action on $\{0,1\}^{\mathbb{N}}$, with generators $a, b, c, d$. On the orbit of $111...$, ignoring the labels, this is the following infinite $4$-regular undirected (multi)graph $S$:
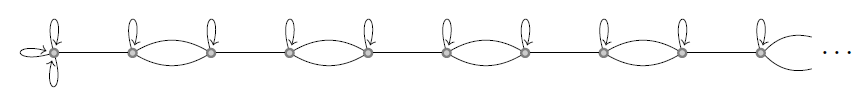and we have some group action $V(S) \curvearrowleft G$ of $G$ on the nodes of $S$, which just follows the edges. This action of course depends on the omitted edge labels, but the argument that follows does not. Label the nodes $V(S)$ of the graph $S$ with nonnegative integers, in order from left to right. Let $\phi : M_4 \to G$ be the natural map from the free monoid on generators $a,b,c,d$ to $G$. Define
$$ T_k = \{g \in G \;|\; 0 \cdot g = k \}, $$
and
$$ V_n = \mathbb{S}_{M_4}(2n) \cap \phi^{-1}(T_{2n}) \subset M_4 $$
where $\mathbb{S}_{M_4}(2n)$ is the Cayley sphere, i.e. words of length exactly $2n$. So $V_n$ is just the set of all words of length $2n$ that map $0$ to $2n$ in the action on the Schreier graph. We clearly have $|V_n| = 2^n$ from the form of the graph. Since $q \nmid 2^n$ and
$$ V_n = \bigsqcup_{h \in T_{2n}} \mathbb{S}_{M_4}(2n) \cap \phi^{-1}(h) $$
not all of $|\mathbb{S}_{M_4}(2n) \cap \phi^{-1}(h_n)|$ can be divisible by $q$, that is,
$$ \exists h_n \in T_{2n}: |\mathbb{S}_{M_4}(2n) \cap \phi^{-1}(h_n)| \not\equiv 0 \bmod q. $$
That means the number of words $w \in M_4$ of length exactly $2n$ which represent $h_n$ is not divisible by $q$. Because $p^{2n}$ is precisely the sum of (elements represented by) all words of length $2n$, we have that the coefficient of $h_n$ in $p^{2n}$ is not zero. Since $h_n \in T_{2n}$, $h_n$ must have word norm exactly $2n$ in $G$, and thus the support of $p^{2n}$ is not globally bounded. QED
The argument doesn't work for $a+b+c+d$ in characteristic $2$ (because a geodesic branches over $\{b,c\}$-bridges into an even number of geodesics), and currently we don't know if $a+b+c+d$ has infinite order over $\mathrm{char} \; 2$. For the same reason, the approach doesn't work for Mark Sapir's suggestion $1+a+b+c$ in characteristic $2$. Maybe it's not so hard to count geodesics directly, we'll try that next.
In any case, the problem can be solved this way: if we pick another polynomial the Schreier graph changes in an entirely local way, and with a bit of experimenting you can find ones where the argument goes through. A particularly nice Schreier graph is obtained for $ada+dad+c$. It has a unique infinite geodesic (and some dead ends which die in a few steps). This gives:
For any field $K$, $ada + dad + c \in K[G]$ has infinite order.
The proof of this is simpler than the above in that you don't need to think about parities (since, as mentioned, geodesics are essentially unique), but you need to do a bit of local analysis on the Schreier graph (which I think is easier to reproduce than to write).
The first argument works directly for all groups $G_\omega$ in the Grigorchuk family. For the second, you can use one of $ada + dad + c$, $aca + cac + b$ or $aba + bab + d$ depending on the first symbol of $\omega$, but otherwise the argument is the same.
I don't know if these polynomials are invertible, but it seems you can make the matrices invertible in a standard way as soon as $d \geq 2$: suppose $p \in K[G]$ has infinite multiplicative order and $K$ is finite (this is the case for our $p$, as we can consider $p$ to be over the prime field). Then $p, p^2, p^3, ...$ must be linearly independent. Consider then $A = \begin{pmatrix} 0 & 1 \\ 1 & p \end{pmatrix}$ (which has inverse $A^{-1} = \begin{pmatrix} -p & 1 \\ 1 & 0 \end{pmatrix}$). By induction $A^n = \begin{pmatrix} O(p^{n-1}) & O(p^{n-1}) \\ O(p^{n-1}) & p^n + O(p^{n-1}) \end{pmatrix}$ (where $O(p^{n-1})$ just means linear combinations of powers of $p$ up to and including $n-1$) so all these matrices are distinct by linear independence of $p^n$ from the previous powers.
For any field $K$, any $d \geq 1$ and any group $G = G_\omega$ in the Grigorchuk family, there exists a matrix $A \in M_d(K[G])$ of infinite order. If $d \geq 2$, there is such an invertible matrix.
