Proof that $Sing^IX$ is $I$-invariant for an interval object in a site by "simplicial decomposition"

Question

I just saw a proof showing that $Sing^{A^1}X$ is $A^1$ invariant where they use an algebraic topological analogue of ``simplcial decomposition'' defined as follow:

The argument works by showing that there is a chain homotopy between the map $\partial_0^*$ and $\partial_1^*$ induced by the $0$ and $1$-section:

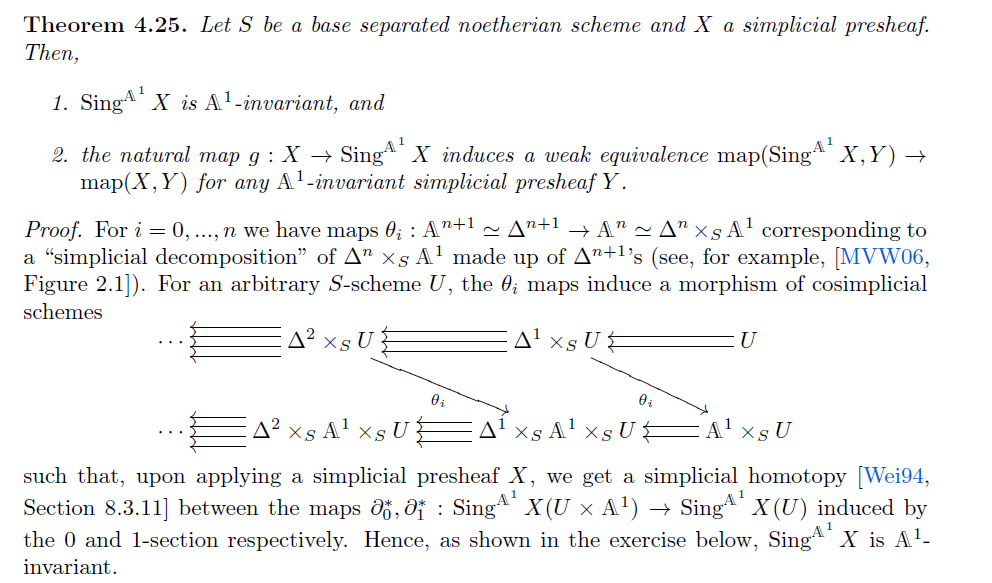
I wonder if this proof works for the case $I$ is an interval object for a site, as defined in Section 2.3, Morel and Voevodsky. If it does, how to define vertex and simplicial decomposition for a general cosimplicial object. I suppose that to define a vertex, one needs the presheaf (or sheaf) $I$ to be a presheaf of group. If not, What is the proof for $Sing^IX$ is $I$-invariant for an interval object?

Answer 1

This is explained in detail in https://ncatlab.org/nlab/show/fundamental+%28infinity%2C1%29-category#FundGeomInftCat, where a cosimplicial object together with the corresponding singular functor is constructed for an arbitrary interval object.