What is the growth rate of the products of binomial coefficients?

Question

Question 1: Are the following empirically observed relationships true

$$ {n \choose 1^a}{n \choose 2^a}{n \choose 3^a}\cdots {n \choose m^a} \sim \exp\bigg(\frac{2n^{1 + \frac{1}{a}}}{a+3}\bigg) $$ where $a$ is a fixed positive integer and $m = \lfloor n^{1/a}\rfloor$.

$$ {n \choose b}{n \choose 2b}{n \choose 3b}\cdots {n \choose mb} \sim \exp\bigg(\frac{n^{2}}{2b}\bigg) $$ where $b$ is a fixed positive integer and $m = \lfloor n/b\rfloor$.

Question 2: What is the growth rate of

$$ {n \choose 1^ab}{n \choose 2^ab}{n \choose 3^ab}\cdots {n \choose m^ab} $$

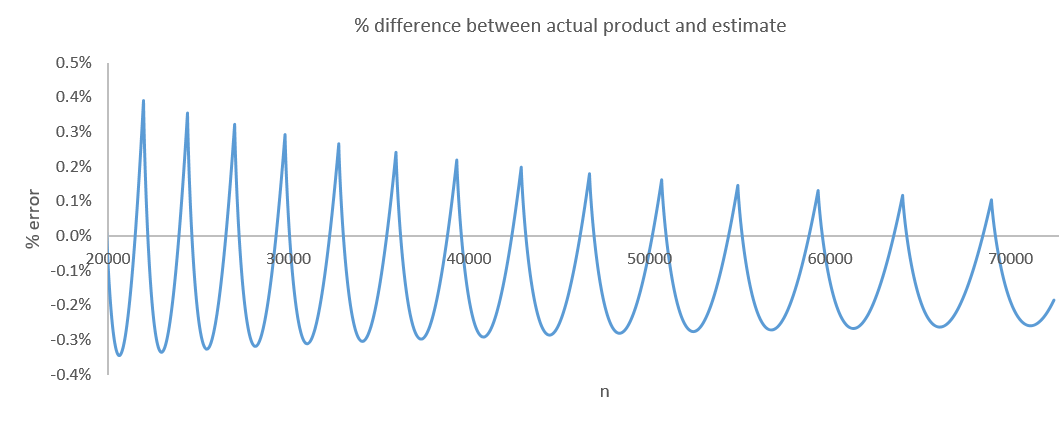
For $a = 3$, the $\%$ error between the asymptotic and the actual product is shown below. We observe that the error is small and is decreasing with $n$?

Note: Posted in MO since it was unanswered in MSE.

Answer 1

Partial answer (but the general case should be similar): using Stirling+Euler-MacLaurin+Glaisher's constant we have for $a=1$: $$\prod_{1\le j\le n}\binom{n}{j}\sim n^{-(n/2+1/3)}e^{n^2/2+n(1-\log(2\pi)/2)+K}$$ with $$K=1/12-2\zeta'(-1)-\log(2\pi)/2\;.$$ Thus I presume that $A\sim B$ in the OP's question means that $\log(A)/\log(B)$ tends to 1.