0
$\begingroup$

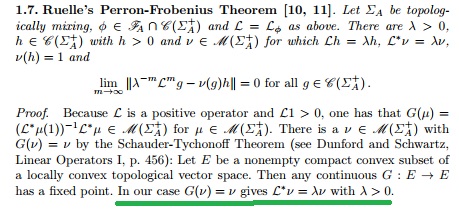
In the passage that I marked in green apparently the author uses a relationship between fixed point and eigenvalues. The result that I know of to ensure the existence of this eigenvalue requires that the domain be a banach space and have a normal cone as a subset, in which case this eigenvalue would be exactly the spectral radius of the dual operator. My question is whether in this theorem are we using this result to guarantee the existence of this eigenvalue? Or is there another result that can guarantee this existence?

enter image description here

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ I think there is no normal cone here, but instead compactness of the unit ball in the weak* topology (and continuity of $\mathcal L^*$ in that topology. $\endgroup$
    – Anthony Quas
    Commented Aug 7, 2019 at 19:47
  • 1
    $\begingroup$ @AnthonyQuas: Well, the argument in the quote certainly refers to what you write in your comment. Still, if one likes cones, one can also argue as follows: Given that the space of contiunous functions $C(K)$ on a compact space $K$ has a normal cone with non-empty interior, it follows, for every positive operator $T$ on $C(K)$, that the spectral radius of $T$ is an eigenvalue of the dual operator $T^*$. $\endgroup$
    – Jochen Glueck
    Commented Aug 7, 2019 at 20:28

0

Reset to default

You must log in to answer this question.