Distinguishing Square Knot and Granny Knot using Quandles

Question

It is known that the square knot and the granny knot are nonequivalent although they have isomorphic fundamental groups.

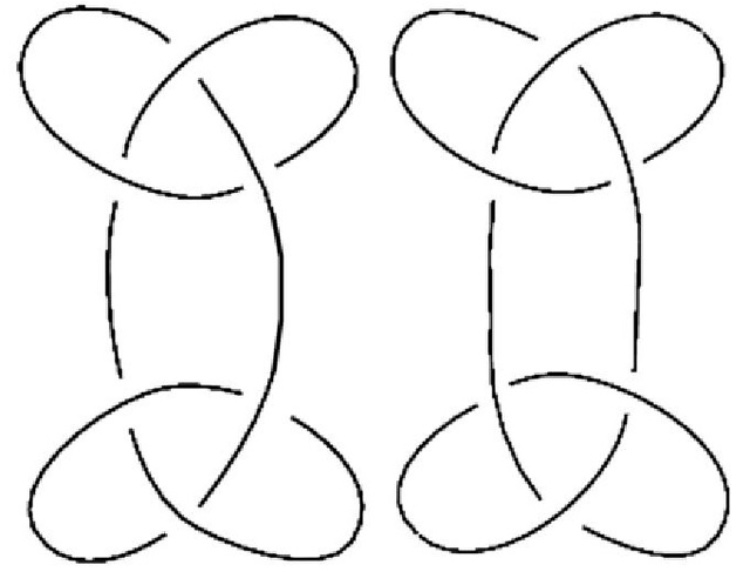
I want to write a work on knot theory and provide these knots as an example where quandles are a stronger invariant than fundamental groups.

I want an example of a finite quandle such that the square knot and the granny knot have a different number of colorings.

As far as I know, it is not guaranteed that such a quandle exists. In his paper „A combinatorial approach to knot recognition“, Stanovsky links a List of 354 Simple Quandles, which I tried through without success. I tried the first 25 of the 26 quandles mentioned in „Quandle Colorings of Knots and Applications“, but didn't have the resources to try the last one.

The program output was kind of weird at some places so I wouldn't be surprised if I made a mistake.

Answer 1

See Example 3.6 of Ellis and Fragnaud, Computing with knot quandles. It says,

The following commands use the quandle invariant ColQ(K) to establish that the granny knot is not equivalent to the square knot. We take Q to be the seventeenth quandle in our enumeration of connected quandles of order 24.

gap> Q:=ConnectedQuandle(24,17,"import");;
gap> K:=PureCubicalKnot(3,1);;
gap> L:=ReflectedCubicalKnot(K);;
gap> square:=KnotSum(K,L);;
gap> granny:=KnotSum(K,K);;
gap> gcsquare:=GaussCodeOfPureCubicalKnot(square);;
gap> gcgranny:=GaussCodeOfPureCubicalKnot(granny);;
gap> Qsquare:=PresentationKnotQuandle(gcsquare);;
gap> Qgranny:=PresentationKnotQuandle(gcgranny);;
gap> NumberOfHomomorphisms(Qsquare,Q); 408
gap> NumberOfHomomorphisms(Qgranny,Q); 24

[Please don't ask me what any of that means. I'm sure it's explained in the paper.]