Let $Q$ be a polygon in the plane. Modify $Q$ by rotating each edge about its midpoint by $180^\circ$. The result is $Q$ again: No change.
This suggests exploring a similar operation in $\mathbb{R}^3$ (somewhat analogous to the earlier questions, The limit of edge-midpoint convex polyhedra , and "Derived" polyhedra and polytopes .).
Let $P_0$ be a (closed) convex polyhedron. Modify $P_0$ by rotating each face about its centroid, about the face normal, by $\theta=2\pi/3=120^\circ$. This produces a jumble of polygons (triangles if $P_0$ has all faces triangles). Let $P_1$ be the convex hull of these twisted faces. Continue, producing $P_2, P_3, \ldots$. For example:
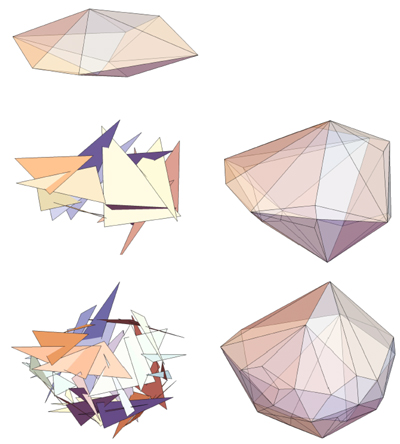
$P_0, P_1, P_2$, of $10, 21, 29$ vertices respectively.
With $\theta=2\pi/3$, the deltahedra (with equilateral triangles faces) are fixed points. My question is:
Q1. Does the iteration "generally" tend to a smooth surface in the limit, perhaps even a sphere? If not, can any characteristics of the limit be identified?
Starting with quite non-spherical $P_0$ does seem to tend toward "rounding out":
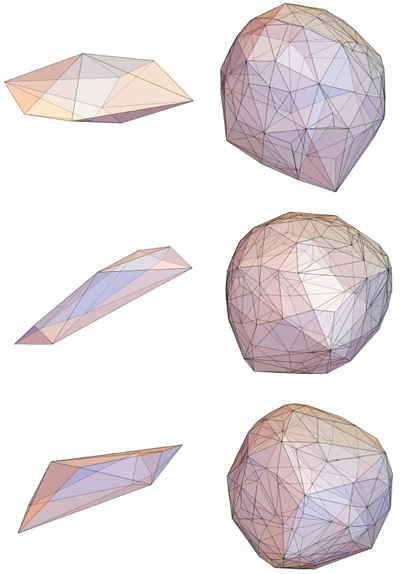
Three random $10$-vertex $P_0$, and their corresponding $P_{20}$.
Q2. Are there any "fixed points" aside from the deltahedra, i.e., some $P_0$ that eventually leads to $P_k$ that is similar to $P_0$, congruent after scaling and rotation?
