I am wondering whether the following concept appears in the group theory literature under some (perhaps different) name. Let $G$ be a group and let $A,B$ be subgroups of $G$.
Definition. Say that $(A,B)$ is a normal pair if $[A,B]\subseteq A\cap B$.
Here, $[A,B]$ denotes the group generated by the commutators $\{[a,b]\colon a\in A,b\in B\}$. Thus, the condition is equivalent to requiring that $[a,b]\in A\cap B$ for all $a\in A$ and $b\in B$. Yet another equivalent condition is that $bA=Ab$ and $aB=Ba$ for all $a,b$. Note that while $A,B$ are required to be subgroups of a common group, the definition is intrinsic to $A$ and $B$ in the sense that for all groups $G,H$ containing $A\cup B$ we have that $(A,B)$ is a normal pair when regarded as subgroups of $G$ if and only if it is a normal pair when regarded as subgroups of $H$.
The concept of a normal pair generalizes the concept of a normal subgroup. Indeed, $N\trianglelefteq G$ is equivalent to $N$ being a subgroup of $G$ for which $(G,N)$ is a normal pair.
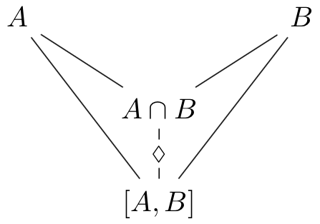
One nice consequence of the definition is that whenever $(A,B)$ is a normal pair, $[A,B]\trianglelefteq A\cap B$. So even though we started by just assuming a subgroup relationship, it was upgraded to a normal subgroup "for free" (see diagram below). To see why this is the case, note (by the previous paragraph) that it suffices to show that $$(A,B)\text{ is a normal pair}\implies([A,B],A\cap B)\text{ is a normal pair}.$$ It is clear that given the hypothesis, $\bigl[[A,B],A\cap B\bigr]\subseteq [A\cap B,A\cap B]$. The latter group is immediately seen to lie both in $A\cap B$ as well as $[A,B]$. This proves the claim.
In fact, one can go even further and observe that the factor group $$\frac{A\cap B}{[A,B]}$$ (which is well-defined due to the previous paragraph) is always abelian. Indeed, it is a general fact (directly from the definitions) that a quotient group $G/N$ is abelian if and only if $[G,G]\subseteq N$. Applied to $G=A\cap B$ and $N=[A,B]$, it yields the claim.
Similar reasoning shows that if $(A,B)$ is a normal pair, then not only is $[A,B]$ a normal subgroup of $A\cap B$, but it is also a normal subgroup of $A$ (and therefore $B$, by symmetry). Indeed, $[[A,B],A]\subseteq [A\cap B,A]$ and the latter subgroup is seen to be contained in both $A$ and $[A,B]$. However, contrasting with the situation in the previous paragraph, $A/[A,B]$ is no longer necessarily abelian - for example, consider the normal pair $(G,\{\textrm{id}\})$ for any non-abelian group $G$.
Following the spirit that "everything in sight is normal", it also follows from normality of the pair $(A,B)$ that $A\cap B$ is a normal subgroup of $A$ (and therefore $B$, by symmetry). Indeed, $[A,A\cap B]\subseteq A$ is clear and $[A,A\cap B]\subseteq [A,B]\subseteq A\cap B$ establishes the claim. (Note that this case of normality is implied by what we have already established in previous paragraphs as well.)
Relations entailed by a normal pair $(A,B)$:
Legend: lines indicate normal subgroups (lower object contained in upper object) and $\Diamond$ means that the quotient group is abelian.
In summary, I think this normal pair concept is quite a nice way to think about normal subgroups. I would be surprised if such a nice concept doesn't exist in the literature. And if it does exist, I would be curious to see some applications of it. One basic application that comes to mind is that various basic properties of the lower central series of a group follow immediately from our observations above.
Note: This question is cross-posed from Math.SE