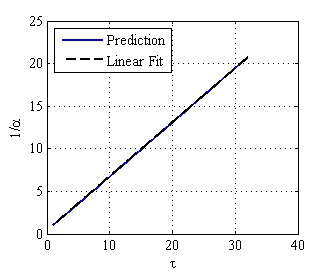
Examine the polynomial $$ x^{\tau+1}-x^{\tau}+\alpha=0\, $$ and denote by $\left|x_{\max}\left(\tau,\alpha\right)\right|$ as the maximal magnitude of a root of this equation. For $\tau>1$, I observed numerically that this root lies on the unit circle, i.e. $$ \left|x_{\max}\left(\tau,\alpha\right)\right|=1 $$ if and only if $$ \frac{1}{\alpha}= a\tau+b $$ for some constants $a$ and $b$. This numerical relation ("prediction"), and it's linear fit could be seen here
I wonder if this linear relationship could be proved (at least in some limit).
Thanks in advance!